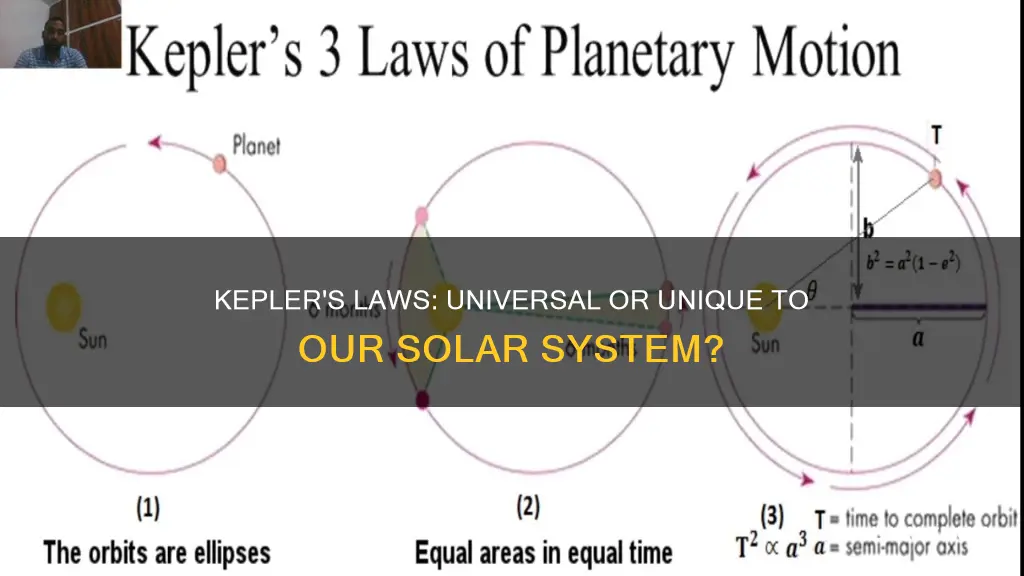
Kepler's laws of planetary motion describe how planets orbit the Sun. They state that planets move in elliptical orbits with the Sun as a focus, a planet covers the same area of space in the same amount of time no matter where it is in its orbit, and a planet's orbital period is proportional to the size of its orbit. These laws were formulated by German mathematician and astronomer Johannes Kepler in the early 17th century, revolutionizing humanity's understanding of the solar system. But do Kepler's laws apply to other solar systems? The short answer is yes. Kepler's laws can be applied to understand the motions of extrasolar planets and stars in binary systems. However, modifications are needed to account for the different masses of stars compared to our Sun. This involves using the formula R = (T^2 x Ms)^1/3, where Ms is the star's mass relative to the Sun's mass.
| Characteristics | Values |
|---|---|
| Do Kepler's laws apply to other solar systems? | Yes |
| Number of laws | 3 |
| First law | All planets move around the Sun in elliptical orbits, with the Sun as one focus of the ellipse. |
| Second law | A radius vector joining any planet to the Sun sweeps out equal areas in equal lengths of time. |
| Third law | The squares of the orbital periods of the planets are directly proportional to the cubes of their mean distances from the Sun. |
| Named after | Johannes Kepler |
| Year of publication | 1609, 1619 |
What You'll Learn
- Kepler's laws describe how planets orbit the Sun
- Kepler's laws explain how planets move in elliptical orbits with the Sun as a focus
- Kepler's laws describe how a planet covers the same area of space in the same amount of time
- Kepler's laws explain how a planet's orbital period is proportional to the size of its orbit
- Kepler's laws apply to other solar systems

Kepler's laws describe how planets orbit the Sun
The first law states that the orbit of a planet is an ellipse with the Sun at one of the two foci. This means that the distance between the planet and the Sun is constantly changing as the planet moves along its elliptical path. The Sun is located at one focus point, offset from the centre of the ellipse.
The second law states that a line joining a planet and the Sun sweeps out equal areas of space during equal time intervals as the planet orbits. In other words, planets do not move at a constant speed along their orbits. Instead, their speed varies so that the line joining the centres of the Sun and the planet covers an equal area in equal amounts of time. This law implies that a planet moves fastest when it is closest to the Sun (perihelion) and slowest when it is furthest away (aphelion).
The third law states that the square of a planet's orbital period is proportional to the cube of the length of the semi-major axis of its orbit. In simpler terms, this means that the period of a planet's orbit increases rapidly with the radius of its orbit. For example, Mercury, the innermost planet, takes only 88 days to orbit the Sun, while Saturn, the sixth planet from the Sun, takes 10,759 days.
Kepler's laws were a significant advancement in our understanding of planetary motion and played a crucial role in the development of Isaac Newton's theory of universal gravitation. They also served as a springboard for newer theories that more accurately describe planetary orbits. Furthermore, Kepler's laws have applications beyond our solar system, as astronomers have discovered over 4,000 exoplanets and can use these laws to calculate their orbits and masses.
Administrative Law: Beyond Traffic Violations?
You may want to see also

Kepler's laws explain how planets move in elliptical orbits with the Sun as a focus
Kepler's laws describe how planets move in elliptical orbits with the Sun as a focus. An ellipse is a shape that resembles a flattened circle, and the specific type of ellipse that defines a planet's orbit is known as an orbital ellipse. The sum of the distances from the two foci of an ellipse to any point on the ellipse is always constant. In the case of planetary orbits, one focus is the Sun, and the other is empty. This means that the planet and the Sun orbit a mutual centre of mass, but because the Sun's mass is so much larger than that of the planet, this centre of mass is usually beneath the Sun's surface.
The orbit of each planet is an ellipse with the Sun at one of the two foci. The Sun is not at the centre of the ellipse but at an offset point, and the planet-Sun distance is constantly changing as the planet moves along its orbit. The orbit of a planet is not a perfect circle but an elongated or flattened circle, with the specific difficulties of predicting the movement of Mars being due to its orbit being the most elliptical of the planets for which there is extensive data.
The orbit of a planet is also defined by its eccentricity, which measures how flattened a circle it is. Eccentricity is a number between 0 and 1, with 0 representing a perfect circle and 1 representing a flat line, technically known as a parabola. The orbit of the Earth, for example, has an eccentricity of 0.0167, making it very nearly a perfect circle.
Kepler's laws also describe how a planet covers the same area of space in the same amount of time, no matter where it is in its orbit. This means that planets do not move at a constant speed along their orbits. Instead, their speed varies so that the line joining the centres of the Sun and the planet sweeps out equal parts of an area in equal times. This results in a planet moving fastest when it is closest to the Sun (perihelion) and slowest when it is furthest away (aphelion).
Fair Housing Laws: Who Do They Protect in Georgia?
You may want to see also

Kepler's laws describe how a planet covers the same area of space in the same amount of time
This law states that the imaginary line joining a planet and the Sun sweeps out equal areas of space during equal time intervals as the planet orbits. In other words, a planet does not move with a constant speed along its orbit. Instead, its speed varies so that the line joining the centres of the Sun and the planet covers an equal area in equal amounts of time.
The point of a planet's nearest approach to the Sun is called the perihelion. The point of greatest separation is the aphelion. Therefore, by Kepler's Second Law, a planet moves fastest when it is at perihelion and slowest when at aphelion.
Kepler's Second Law can be illustrated by drawing a triangle from the Sun to a planet's position at one point in time and its position at a fixed time later. The area of that triangle is always the same, anywhere in the orbit. For all these triangles to have the same area, the planet must move more quickly when it is near the Sun and more slowly when it is farther away.
Kepler's Second Law led to the formulation of his First Law: that planets move in elliptical orbits with the Sun at one focus point, offset from the centre. The orbit of every planet is an ellipse with the Sun at one of the two foci. The Sun is at one focus, and the planet follows the ellipse in its orbit, meaning that the distance between the planet and the Sun is constantly changing as the planet orbits.
Kepler's laws describe the motion of bodies subject to central gravitational force. However, such motion does not always follow the elliptical orbits specified by the First Law but can take paths defined by other, open conic curves. The motion can be in parabolic or hyperbolic orbits, depending on the total energy of the body.
Kepler's laws apply to all inverse-square-law forces. They also apply to electromagnetic forces within the atom, if due allowance is made for relativistic and quantum effects.
HIPAA Laws: Do They Apply to Insurance?
You may want to see also

Kepler's laws explain how a planet's orbital period is proportional to the size of its orbit
Kepler's three laws describe how planets orbit the Sun. Kepler's Third Law states that the squares of the orbital periods of planets are directly proportional to the cubes of the semi-major axes of their orbits. This is written in equation form as p^2=a^3. Kepler's Third Law implies that the period for a planet to orbit the Sun increases rapidly with the radius of its orbit.
For example, Mercury, the innermost planet, takes only 88 days to orbit the Sun. Earth takes 365 days, while distant Saturn requires 10,759 days to do the same.
Kepler's laws apply to other solar systems as well. In 1621, Kepler noted that his third law applies to the four brightest moons of Jupiter. Godefroy Wendelin also made this observation in 1643.
HIPAA Laws: Do They Extend to Military Personnel?
You may want to see also

Kepler's laws apply to other solar systems
Kepler's laws of planetary motion describe how planets orbit the Sun. They state that:
- Planets move in elliptical orbits with the Sun as a focus
- A planet covers the same area of space in the same amount of time no matter where it is in its orbit
- A planet's orbital period is proportional to the size of its orbit (its semi-major axis)
These laws were formulated by German mathematician and astronomer Johannes Kepler in the early 17th century, based on the extensive planetary data of Danish astronomer Tycho Brahe. Kepler's laws built upon Nicolaus Copernicus's heliocentric model of the solar system, which placed the Sun at its centre. However, Copernicus incorrectly assumed that the orbits of the planets were circular. Kepler's insight was that planets move in elongated or flattened circles, or ellipses.
Kepler's laws apply to all planets in our solar system, and they also accurately describe the motion of comets. Beyond our solar system, Kepler's laws have been used to calculate the orbits and masses of over 4,000 exoplanets. This is achieved by modifying the formula to account for the variation in the star's mass compared to that of our Sun.
Kepler's laws also apply to other celestial bodies, such as moons orbiting planets, and artificial satellites placed in orbit around them. They can also be used to calculate the masses of stars in binary systems.
Men's Legal Responsibilities: Unique Laws for Men?
You may want to see also







