Boyle's Law, also known as Mariotte's Law, is an empirical gas law that describes the relationship between the pressure exerted by a gas and the volume it occupies when the temperature and amount of gas are held constant. The law states that the pressure and volume of a gas are inversely proportional, meaning that as the volume of a gas increases, its pressure decreases, and vice versa. This law was discovered by Robert Boyle in 1662 and can be expressed mathematically as PV=K, where P is the pressure, V is the volume, and K is a constant.
| Characteristics | Values |
|---|---|
| What is it? | A gas law describing the relationship between pressure and volume of a confined gas |
| When was it discovered? | 1662 |
| Who discovered it? | Robert Boyle, an Anglo-Irish chemist |
| What does it state? | The absolute pressure exerted by a given mass of an ideal gas is inversely proportional to the volume it occupies if the temperature and amount of gas remain unchanged within a closed system |
| What is the mathematical representation? | P ∝ 1/V or PV=K |
| What is the application of Boyle's law? | It is used to explain how the breathing system works in the human body |
What You'll Learn

Pressure and volume relationship
Boyle's law, also known as Mariotte's law, is a fundamental principle in chemistry that describes the behaviour of a gas held at a constant temperature. The law was formulated by the physicist Robert Boyle in 1662 and published in the Journal of Applied Physiology.
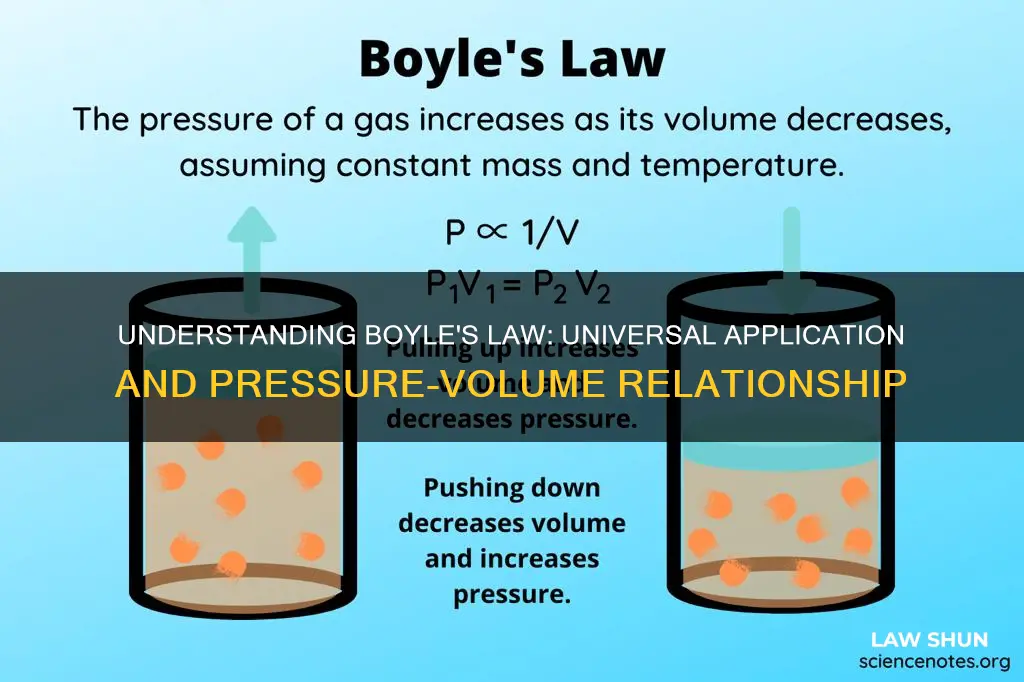
Boyle's law states that the pressure exerted by a gas is inversely proportional to the volume it occupies, as long as the temperature and the amount of gas remain the same. This relationship can be expressed mathematically as:
P ∝ (1/V)
Or:
P = k*(1/V) ⇒ PV = k
Where P is the pressure exerted by the gas, V is the volume it occupies, and k is a constant.
In simple words, this means that in a closed container, the pressure of a gas multiplied by its volume equals and remains a constant as long as the temperature is also constant. For example, if we doubled the volume of a container of gas, the pressure of the gas would be halved.
Boyle's law can be seen in action in everyday examples like a balloon. When air is blown into a balloon, the pressure of that air pushes on the rubber, causing the balloon to expand. If one end of the balloon is squeezed, the volume decreases, and the pressure inside increases, causing the rest of the balloon to expand outward.
Boyle's law is significant because it explains how gases behave and proves that gas pressure and volume are inversely proportional. It also has practical applications in understanding and designing equipment like water tanks, heat pumps, and scuba diving gear.
Agency Law: Commercial and Industrial Real Estate's Legal Framework
You may want to see also

Application in breathing system
Boyle's law, also known as Mariotte's law, is a gas law that describes the relationship between the pressure exerted by a gas and the volume it occupies. It was discovered by Robert Boyle in 1662 and states that, at a constant temperature, the volume of gas is inversely proportional to the pressure exerted by it.
Application in the Breathing System
Boyle's law is often used to explain how the human breathing system works. This involves explaining how the volume of the lungs may be increased or decreased, causing a relatively lower or higher air pressure within them. This creates a pressure difference between the air inside the lungs and the environmental air pressure, which in turn causes inhalation or exhalation as air moves from high to low pressure.
For example, when the volume of the lungs is increased, the pressure inside the lungs decreases, creating a pressure difference that causes air to move from the higher pressure of the environment into the lungs, resulting in inhalation. Conversely, when the volume of the lungs is decreased, the pressure inside the lungs increases, creating a pressure difference that causes air to move from the higher pressure inside the lungs out into the environment, resulting in exhalation.
This application of Boyle's law helps us understand the mechanics of breathing and how changes in lung volume lead to inhalation and exhalation. It also highlights the importance of maintaining a constant temperature in the lungs, as deviations could impact the accuracy of the law's predictions.
In summary, Boyle's law is a fundamental principle that helps explain the human breathing system by describing the relationship between pressure and volume within the lungs and how it influences the exchange of air during respiration.
Usury Laws and Private Loans: What's the Verdict?
You may want to see also

Application in scuba diving
Boyle's Law is a gas law that states that the pressure exerted by a gas is inversely proportional to the volume occupied by it, as long as the temperature and the quantity of the gas remain the same. This means that if the volume of a gas increases, its pressure decreases, and vice versa.
Boyle's Law is extremely important in scuba diving. Air spaces in the body are subjected to pressure and volume changes, in direct proportion to the depth of the dive. This can cause severe damage to the lungs, sinuses, ears, and mask squeeze, and can even be fatal.
A fundamental rule in scuba diving is to "never hold your breath". This is because, according to Boyle's Law, the air in a diver's lungs will increase in volume and decrease in pressure as they ascend, which can cause the lungs to rupture. Divers are trained to gently force air into their Eustachian tubes so that their eardrums do not burst inward from the vacuum created when the volume of air in the inner ear shrinks.
Additionally, the gas density inhaled by the diver increases as they dive deeper, which means that gas absorption also increases. This can lead to nitrogen narcosis, which can cause confusion and be a risk to the diver.
Therefore, it is vital that divers ascend slowly and complete decompression stops to avoid problems caused by Boyle's Law.
Lemon Laws: Do They Cover Motorcycles?
You may want to see also

Application in water tanks
Boyle's Law, also known as the Boyle-Mariotte Law, is an empirical gas law that describes the relationship between the pressure exerted by a gas and the volume it occupies within a closed system. The law states that, provided the temperature and amount of gas remain constant, the pressure and volume of the gas are inversely proportional. This relationship can be expressed mathematically as:
P ∝ (1/V)
Or:
PV = k
Where P is the pressure exerted by the gas, V is the volume occupied by the gas, and k is a constant value representative of the temperature of the system and the amount of gas.
Boyle's Law has various practical applications, including in water tanks. In this context, the law can be used to understand how the volume of air in a water tank changes with variations in pressure, and vice versa.
For instance, consider a water tank with a volume of 10 gallons, containing 10 gallons of water and 20 gallons of air at a pressure of 50 psi. If we were to run all the water out of the tank, the volume of air would increase to 30 gallons, and the pressure would decrease to approximately 33 psi.
Using the formula PV = k, we can calculate the new pressure (P2) by rearranging the formula to P2 = (P1V1)/V2:
P2 = (20 x 50) / 30
P2 = 33.33 psi
So, in this example, as the volume of air in the tank increased, the pressure decreased, in accordance with Boyle's Law.
Boyle's Law can also be applied to calculate the drawdown capacity of a captive air pressure tank in a water system. The drawdown capacity refers to the volume of water that can be drawn from the tank before the pump needs to turn on again. By accounting for factors such as pre-charge pressure, cut-in pressure, cut-out pressure, and total tank volume, Boyle's Law can be used to determine the usable water volume in the tank.
In summary, Boyle's Law provides a fundamental understanding of the relationship between pressure and volume in gases, and this principle can be applied to various scenarios, including water tanks, to predict and explain changes in pressure and volume.
US Territories: Exempt from Federal Drug Laws?
You may want to see also

Application in balloons
Boyle's law, also known as Mariotte's law, is a gas law that describes the relationship between the pressure exerted by a gas and the volume it occupies. The law states that, at a constant temperature, the volume of a gas is inversely proportional to the pressure exerted by it. This means that as the volume of a gas increases, its pressure decreases, and vice versa.
Now, let's explore the application of Boyle's law in balloons in 4-6 paragraphs:
Balloons provide a classic example of Boyle's law in action. When you blow air into a balloon, you are essentially pumping gas into an enclosed space. As per Boyle's law, the pressure of the air inside the balloon is inversely proportional to its volume. So, as you blow air into the balloon and increase its volume, the pressure of the air inside pushes against the rubber surface, causing the balloon to expand. This expansion continues until the pressure exerted by the air inside the balloon equals the pressure outside it (usually atmospheric pressure).
If you squeeze one end of the inflated balloon, you further decrease its volume, which, according to Boyle's law, leads to an increase in pressure. This increased pressure causes the rest of the balloon to expand outward. This is why, when you squeeze a balloon at one end, it seems to get longer. However, there is a limit to how much you can compress the air inside the balloon. If you keep squeezing, the pressure will eventually become so high that it causes the balloon to burst.
Boyle's law can also explain why a balloon rises in the air when released. When you blow air into a balloon, you are filling it with gas molecules, typically a mixture of air and some lighter gases like helium. The pressure of these gas molecules against the inner surface of the balloon is what keeps it inflated. Now, when you release the balloon, it rises because the air pressure inside the balloon is greater than the surrounding atmospheric pressure. As the balloon rises higher, the surrounding atmospheric pressure decreases, allowing the balloon to expand further.
Additionally, Boyle's law can explain why a balloon pops when exposed to a loud noise. When there is a sudden, intense sound, such as a loud bang, the sound waves can create a rapid increase in air pressure. This increase in pressure, when transmitted to the balloon, can exceed the strength of the rubber, causing it to burst. The same principle applies to a balloon that is overinflated; if you keep blowing air into a balloon, the pressure inside will increase to a point where it exceeds the strength of the rubber, leading to a rupture.
Indecent Exposure Laws and Minors in Ohio
You may want to see also