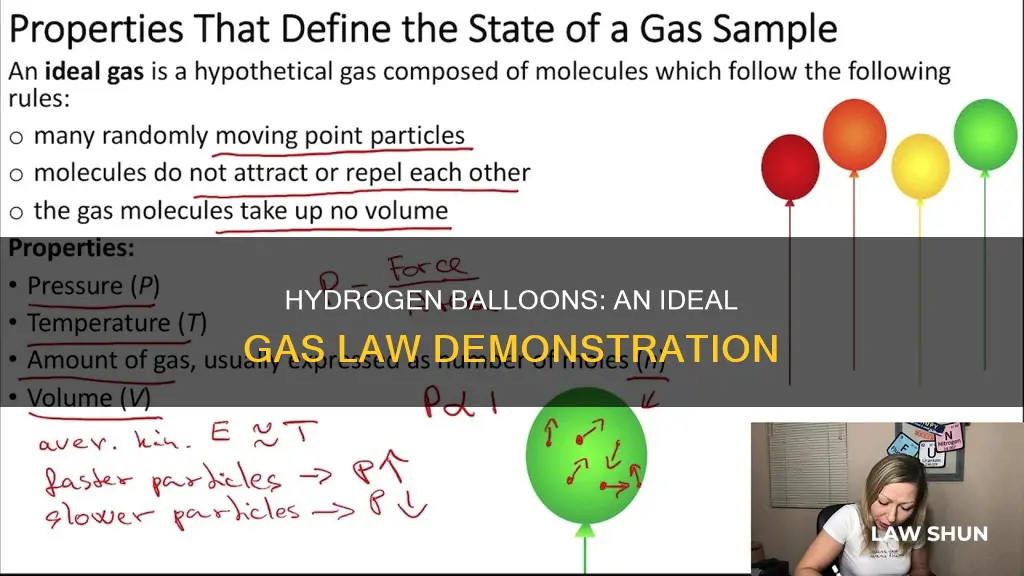
The ideal gas law, derived from the relationships between the pressure, volume, temperature, and amount of a gas, can be used to explain the behaviour of a hydrogen balloon. The law, expressed as PV = nRT, where P is pressure, V is volume, n is the number of moles, T is temperature, and R is the ideal gas constant, helps understand how gases respond to changing conditions. While an ideal gas is a hypothetical concept, the ideal gas law is useful in predicting the behaviour of real gases under most conditions, especially at low pressures and moderate temperatures.
| Characteristics | Values |
|---|---|
| Equation | PV = nRT |
| --- | --- |
| P | Pressure |
| V | Volume |
| n | Number of moles |
| R | Gas constant |
| T | Temperature |
What You'll Learn

Hydrogen balloons and Boyle's Law
Boyle's Law is a fundamental law in chemistry that describes the behaviour of a gas held at a constant temperature. The law, discovered by Robert A. Boyle in 1662, states that at a fixed temperature, the volume of gas is inversely proportional to the pressure exerted by the gas. In other words, when a gas is pumped into an enclosed space, it will shrink to fit into that space, but the pressure that gas puts on the container will increase.
The relationship between pressure and volume can be expressed mathematically as pV=k, where p is the pressure of the gas, V is the volume of the gas, and k is a constant. This means that as the volume of a gas increases, the pressure decreases, and vice versa.
Boyle's Law can be observed in the behaviour of hydrogen balloons. When you blow up a balloon, you are adding air into the system, causing the balloon to get bigger, or increasing in volume. The pressure inside the balloon also increases as you blow air into it, and this pressure is what gives the balloon its shape and makes it expand.
If you squeeze one end of the balloon, making the volume smaller, the pressure inside increases, causing the un-squeezed part of the balloon to expand out. This is because the gas inside the balloon is trying to maintain the same pressure throughout, and when you squeeze one end, the gas molecules in the squeezed area are pushed towards the un-squeezed area, increasing the pressure there.
However, there is a limit to how much you can compress the gas inside the balloon. Eventually, the pressure becomes so great that it causes the balloon to break. This is because the rubber material of the balloon cannot stretch indefinitely and will eventually rupture under the increasing pressure.
Boyle's Law can be applied to hydrogen balloons in various ways. For example, it can help us understand the relationship between the pressure and volume of the gas inside the balloon. It can also help us predict how the balloon will behave under different conditions, such as changes in temperature or pressure.
By using Boyle's Law, we can also calculate the amount of hydrogen gas needed to fill a balloon to a certain volume at a specific temperature and pressure. This can be useful for designing hydrogen balloons for different purposes, such as weather balloons or balloons for scientific experiments.
In summary, Boyle's Law is a fundamental concept in chemistry that helps us understand the behaviour of gases, including those inside hydrogen balloons. By applying this law, we can make predictions, calculations, and design decisions regarding hydrogen balloons and their behaviour under different conditions.
Usury Laws and Commercial Loans: What's the Verdict?
You may want to see also

Hydrogen balloons and Charles' Law
Charles' Law, also known as the law of volumes, describes the relationship between the volume and temperature of a given amount of gas at constant pressure. The law states that the volume of a given amount of gas is directly proportional to its temperature on the Kelvin scale when pressure is held constant. In other words, as the temperature of a gas increases, so does its volume, assuming constant pressure. This relationship can be expressed mathematically as:
> V ∝ T or V = constant x T or V = k x T or V1/T1 = V2/T2
Where V is volume, T is temperature in Kelvin, and k is a proportionality constant.
Now, let's discuss how this law applies to hydrogen balloons. Hydrogen balloons are filled with hydrogen gas, which follows Charles' Law just like any other gas. When a hydrogen balloon is inflated, the volume of the balloon increases as the hydrogen gas inside is heated. This is because the temperature of the gas increases, leading to a corresponding increase in volume according to Charles' Law.
For example, let's consider the first crewed hydrogen balloon flight by Jacques Charles and the Robert brothers in 1783. As the balloon rose, the temperature of the gas inside increased due to the decrease in atmospheric pressure with altitude. As a result, the volume of the gas also increased, causing the balloon to expand. To control the ascent and prevent the balloon from bursting, they had to release some of the hydrogen gas through a valve, which Charles had invented.
Charles' Law also applies when a hydrogen balloon is cooled. If a hydrogen balloon is placed in a cold environment, such as a refrigerator or liquid nitrogen, the temperature of the gas inside decreases, leading to a decrease in volume. This is why a hydrogen balloon will shrink when cooled and expand again when warmed.
In summary, Charles' Law describes the relationship between the volume and temperature of a gas at constant pressure. Hydrogen balloons, like any other gas-filled balloon, follow this law. When a hydrogen balloon is heated, the volume of the gas inside increases, causing the balloon to expand. Conversely, when a hydrogen balloon is cooled, the volume of the gas decreases, leading to shrinkage of the balloon.
Right of Way Laws: City Streets and Keeping Right
You may want to see also

Hydrogen balloons and Avogadro's Law
Avogadro's Law, formulated by the Italian scientist Amedeo Avogadro, states that the volume of a gas is directly proportional to the number of moles of the gas. In other words, equal volumes of gas at the same temperature and pressure contain the same number of molecules. This law is a cornerstone in the study of physical processes and is a special case of the ideal gas law, which relates the pressure, volume, temperature, and number of particles for a given gas.
The law can be expressed mathematically as:
> V ∝ n
Where:
- V is the volume of the gas
- N is the number of moles of the gas
This means that if you double the number of moles of gas, you will also double the volume it occupies, assuming the temperature and pressure remain constant. This principle forms the basis of the ideal gas equation and can be applied to everyday situations such as blowing up a balloon or inflating a tire.
For example, when you blow up a balloon, you are adding more gas molecules to it, which increases the volume of the balloon. Similarly, a flat tire takes up less space than an inflated tire because it contains less air.
Avogadro's Law also has implications for the density of gases. Since the volume of a gas is directly proportional to the number of moles, and the mass of a gas is equal to its molar mass multiplied by the number of moles, it follows that the density of a gas is equal to its molar mass multiplied by the pressure and divided by the temperature and the gas constant. This relationship can be used to calculate the density of gases under specific conditions.
In summary, Avogadro's Law states that the volume of a gas is directly proportional to the number of moles of gas, assuming temperature and pressure remain constant. This law has important applications in understanding the behaviour of gases and can be used to solve problems related to everyday situations.
Faraday's Law: Powering Generators with Electromagnetic Induction
You may want to see also

Hydrogen balloons and the Ideal Gas Law equation
The ideal gas law, derived from the empirical relationships among the pressure, volume, temperature, and number of moles of a gas, can be used to calculate any of these properties if the other three are known. The ideal gas law is defined as:
> PV = nRT
Where:
- P = Pressure of a gas
- V = Volume of a gas
- N = Number of moles of the gas
- T = Temperature of a gas in Kelvin
- R = Ideal gas constant
The ideal gas law can be used to explain the behaviour of hydrogen balloons. Hydrogen balloons were first flown in 1783, and since then, scientists have been able to establish mathematical relationships between the physical properties of gases, including pressure, volume, temperature, and amount of gas.
The ideal gas law is particularly useful when dealing with items such as balloons, as it can be used to calculate the final state of a sample of gas following any changes in conditions. For example, if we know the initial state of a balloon and then alter its conditions, we can use the ideal gas law to predict its final state.
The ideal gas law can also be used to calculate the density of a gas if its molar mass is known or, conversely, the molar mass of an unknown gas sample if its density is measured.
The ideal gas law is a useful tool for understanding the behaviour of gases and can be applied to various situations, including those involving hydrogen balloons.
Muffler Deletes and Exhaust Laws: What You Need to Know
You may want to see also

Hydrogen balloons and the General Gas Equation
The ideal gas law is a fundamental concept in chemistry and physics, and it plays a crucial role in understanding the behaviour of gases, including those in hydrogen balloons. The ideal gas law is derived from empirical relationships among the pressure, volume, temperature, and number of moles of a gas. It can be used to calculate any of these four properties if the other three are known. The equation for the ideal gas law is:
> PV = nRT
Where:
- P is the pressure of the gas
- V is the volume of the gas
- N is the number of moles of the gas
- R is the gas constant
- T is the temperature in Kelvin
The ideal gas law describes the behaviour of an ideal gas, which is a hypothetical substance that follows the ideal gas law and the kinetic molecular theory of gases. While there is no such thing as a true ideal gas, this concept is useful for understanding how real gases behave under certain conditions. The ideal gas law is especially accurate for gases at low pressures and moderate temperatures.
The general gas equation is derived from the ideal gas law and is used to describe the relationship between the initial and final conditions of a gas. It is given as:
> (P1V1) / (n1T1) = (P2V2) / (n2T2)
Where the subscripts 1 and 2 refer to the initial and final conditions, respectively. This equation is useful when one or more of the gas properties are held constant between the two conditions. By rearranging the equation and solving for the unknown variable, we can determine how changes in temperature, pressure, volume, or amount of gas impact the behaviour of the gas.
When applying the ideal gas law to hydrogen balloons, we can gain insights into how the gas inside the balloon responds to changes in its environment. For example, if we know the initial pressure, volume, temperature, and amount of hydrogen gas inside the balloon, we can use the ideal gas law to predict how these properties will change as the balloon ascends or descends to different altitudes, where the external pressure and temperature differ.
Additionally, the ideal gas law can help explain why hydrogen balloons float. Hydrogen gas has a lower density than air due to its lower molecular weight. When a hydrogen balloon is filled and released, it rises because the surrounding air exerts a greater buoyant force on it than the weight of the displaced air. This is a result of the inverse relationship between the density and temperature of gases, as described by the ideal gas law:
> n/V ∝ 1/T
In other words, as the temperature of a gas increases, its density decreases, and vice versa. This relationship is essential for understanding why hot air balloons rise and why hydrogen balloons are able to float.
In summary, the ideal gas law and the general gas equation provide a quantitative framework for understanding the behaviour of gases, including those in hydrogen balloons. By applying these equations, we can calculate how changes in pressure, volume, temperature, or amount of gas impact the behaviour of the gas and explain phenomena such as buoyancy and changes in volume duringsection.
Anti-Discrimination Laws: Limits on Free Speech?
You may want to see also
Frequently asked questions
The ideal gas law is a relationship between the pressure, volume, temperature, and number of particles for a given gas. The equation looks like this:
PV = nRT
The ideal gas law states that the density and temperature of gases are inversely proportional. This means that hot air will rise in cool air because its density is less, creating buoyancy.
The ideal gas equation is PV = nRT, where P is pressure, V is volume, n is the number of moles, T is temperature, and R is the ideal gas constant.







