Hydrostatics is a branch of fluid mechanics that studies fluids at rest in stable equilibrium. It is fundamental to hydraulics and is relevant to many other fields, such as astrophysics, geophysics, meteorology, and medicine. Hydrostatics can be used to explain various phenomena in our daily lives, such as why oil and wood float on water, why atmospheric pressure changes with altitude, and why the surface of still water is always level according to the curvature of the Earth.
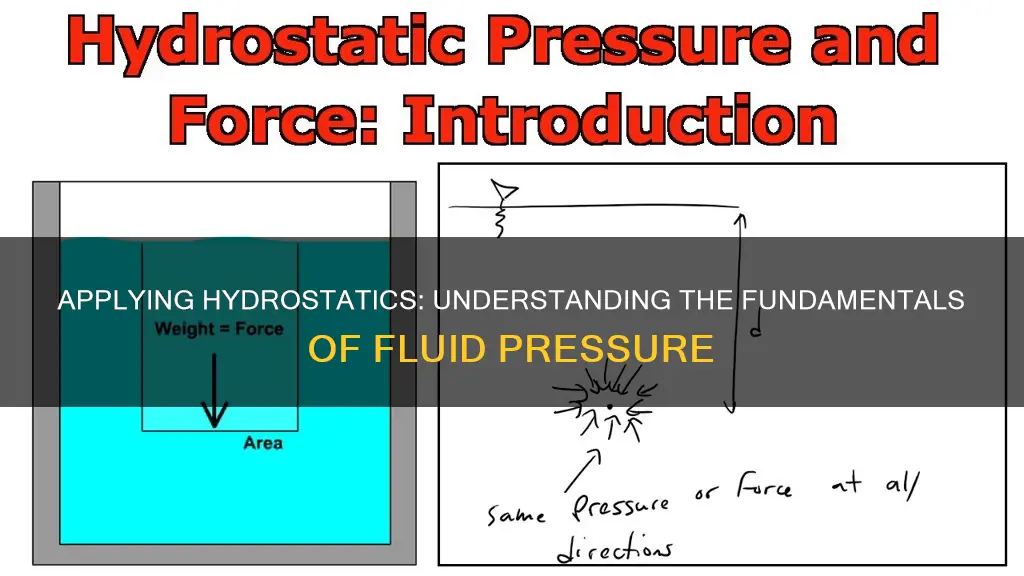
The French mathematician, philosopher, and physicist Blaise Pascal formulated the concept of pressure and its transmission by fluids in 1647. Pascal's Law, also known as the principle of transmission of fluid pressure, states that any pressure applied to the surface of a fluid at rest is transmitted uniformly throughout the fluid in all directions. This law is applicable to liquids but not gases.
The pressure exerted by a fluid at equilibrium at any point in time due to the force of gravity is known as hydrostatic pressure. It is influenced by the depth measured from the surface, as the weight of the fluid increases when a downward force is applied. The formula for calculating hydrostatic pressure takes into account the density of the liquid, the acceleration due to gravity, and the height of the fluid column.
| Characteristics | Values |
|---|---|
| Definition | The pressure exerted by a fluid at equilibrium at any point in time due to the force of gravity |
| Formula | p = ρgh |
| ρ | Density of the liquid in kg.m-3, slugs.ft-3 |
| g | Acceleration due to gravity taken as 9.81m/s2 |
| h | Height of the fluid column in m |
| Application | Hydrostatics is fundamental to hydraulics, geophysics, astrophysics, meteorology, and medicine |
What You'll Learn

How to calculate hydrostatic pressure
Hydrostatic pressure is the pressure exerted by a fluid at rest due to the Earth's gravitational pull. It is calculated using the following formula:
P = ρ × g × h + p0
Where:
- P = hydrostatic pressure
- Ρ = density of the fluid
- G = gravitational acceleration (9.80665 m/s^2 for Earth)
- H = height or depth of the fluid
- P0 = external pressure (usually atmospheric pressure, 101.33 kPa or 1 atm)
This formula can be used to calculate the hydrostatic pressure in a column of fluid, such as the pressure at the bottom of a swimming pool or in a pipe. The pressure increases with depth, as the weight of the fluid above contributes to the overall pressure.
For example, to calculate the hydrostatic pressure at a depth of 2 meters in a pool, you would use the formula:
P = ρ × g × h + p0
Let's assume the density of water is 1000 kg/m^3 and the external pressure is atmospheric pressure (101.33 kPa). Plugging in the values:
P = 1000 × 9.80665 × 2 + 101.33
P = 19,613 kPa + 101.33 kPa
P = 20,626 kPa or 2.0 atm
So, the hydrostatic pressure at a depth of 2 meters in the pool is approximately 2.0 atmospheres or 20,626 kilopascals.
It's important to note that the shape of the container doesn't affect the hydrostatic pressure, only the height or depth of the fluid. This is known as the hydrostatic paradox.
Hydrostatic pressure calculations are also used in various engineering applications, such as drilling fluids in oilfields, hydraulic systems, and medical applications like blood pressure measurements.
Weber's Law: Understanding Difference Threshold Sensitivity
You may want to see also

Pascal's Law and its applications
Pascal's Law, formulated by French mathematician and philosopher Blaise Pascal in 1647, is a fundamental principle of fluid mechanics. It states that any pressure applied to the surface of a fluid at rest is transmitted uniformly throughout the fluid in all directions, without any change in magnitude. This means that the pressure at a point in a static fluid is the same in all directions.
Pascal's Law can be expressed by the formula:
P = F/A
Where:
- P = Pressure
- F = Force
- A = Area of contact
This law has numerous applications in our daily lives and various fields, including engineering and technology. Here are some examples:
- Hydraulic Machines: Pascal's Law is the basis for hydraulic power machines such as hydraulic lifts, hydraulic jacks, and hydraulic presses. These machines use fluids to transmit pressure and amplify force, allowing for the lifting of heavy objects or the application of a strong retarding force, such as in braking systems.
- Hydraulic Brakes: In automobiles, when a small force is applied to the brake pedal, the master piston moves inside the master cylinder, transmitting pressure through the brake fluid to act on a larger piston. This results in a much larger force being applied to the brake shoes, producing a strong retarding force on the wheel.
- Aircraft Hydraulic Systems: Aircraft use hydraulic power systems based on Pascal's Law for slowing down on runways and controlling landing gears and flight mechanisms.
- Hydraulic Pumps: These are used in the automobile industry for the discharge of fluids.
- Calibration and Compression: Pascal's Law is applied in calibrating pressure gauges and in pressing oils, such as olive and hazelnut oils.
- Pneumatic Devices: Various pneumatic devices, such as dentist's drills, jackhammers, paint sprayers, and air brakes on trucks, utilise Pascal's Law.
- Understanding Atmospheric Pressure: Pascal's Law helps explain why the atmospheric pressure changes with altitude.
- Medical Applications: Pascal's Law is relevant in medicine, particularly in understanding blood pressure and the hydrostatic pressure in blood vessels.
- Understanding Buoyancy: Pascal's Law, along with Archimedes' Principle, helps explain why objects float in fluids and how ships can float despite their weight.
Cube-Root Law: Low Gravity's Relevance Explored
You may want to see also

Archimedes' Principle
According to this principle, when an object is fully or partially submerged in a fluid, it experiences an upward buoyant force equal to the weight of the fluid it displaces. This buoyant force effectively reduces the apparent weight of the object by the weight of the displaced fluid.
Mathematically, the buoyant force (Fb) can be expressed as:
> Fb = ρVg
Where:
- Fb denotes the buoyant force applied to the submerged object
- Ρ denotes the density of the fluid
- V represents the volume of the displaced fluid
- G is the acceleration due to gravity
For example, consider a 5-kg object immersed in water. According to Archimedes' principle, it will experience a buoyant force of 2 kg, which is equal to the weight of the displaced water. This reduces the apparent weight of the object from 5 kg to 3 kg.
On the other hand, if the weight of the object is greater than the weight of the displaced fluid, the object will sink. This is because the buoyant force can counteract the force of gravity, preventing the object from sinking.
Tennessee Maternity Laws: Who is Bound by Them?
You may want to see also

The buoyancy and stability of floating objects
Archimedes' principle states that the buoyant force on an object immersed in a fluid is equal to the weight of the fluid displaced by the object. This can be calculated using the equation:
> Fb = ρVg
Where:
- Fb denotes the buoyant force
- Ρ denotes the density of the fluid
- V represents the volume of the displaced fluid
- G is the acceleration due to gravity
The net force on an object is the difference between the magnitudes of the buoyant force and its weight. If the net force is positive, the object rises; if negative, the object sinks; and if zero, the object remains in place with neutral buoyancy.
For example, a rock that weighs 10 newtons in a vacuum will exert a force of 7 newtons on the string it hangs from when lowered into water and displacing 3 newtons of water.
The stability of a floating object refers to its ability to restore itself to an equilibrium position after a small displacement. Vertical stability is common among floating objects, as a downward force will create a greater buoyancy force, pushing the object back up. Rotational stability is also important, especially for vessels. A floating object will be stable if its center of gravity is beneath its center of buoyancy, as any angular displacement will result in a 'righting moment'.
The shape of a floating object also plays a role in its stability. For instance, a submarine can rise by expelling water from its buoyancy tanks, as its volume remains constant while its mass decreases. Similarly, a balloon rises as it increases in volume with reducing atmospheric pressure, but not as much as the air on which it rides, causing its average density to decrease less than that of the surrounding air.
In summary, the buoyancy and stability of floating objects are key concepts in hydrostatics, with Archimedes' principle providing a fundamental understanding of how objects float and remain buoyant in fluids.
Kepler's Laws: Universal or Not?
You may want to see also

The pressure and other fluid properties inside containers
The pressure inside a fluid-filled container is influenced by the fluid's density and the depth of the fluid column above a specific point. This is known as hydrostatic pressure, which is the pressure exerted by a fluid at equilibrium due to the force of gravity. The hydrostatic pressure is proportional to the depth from the surface, as the weight of the fluid increases with a downward force.
According to Pascal's Law, the pressure at a point in a fluid at rest is the same in all directions. This means that the pressure on the bottom of a container is the same, regardless of its shape or the volume of liquid above it. This is known as the hydrostatic paradox.
The pressure inside a container can be measured using a piezometer tube or a U-tube manometer. A piezometer tube is a simple device that measures pressure by observing the height of a fluid column above a specific point. A U-tube manometer, on the other hand, measures pressure differences by balancing the weight of a manometric liquid between two applied gas pressures.
In addition to pressure, other fluid properties that can be analysed inside containers include temperature and density. The equation of state can be used to relate the pressure, density, and temperature of a gas. For liquids, there is no equivalent equation of state because they are considered incompressible, and their density remains constant.
When Do Laws of War Apply?
You may want to see also
Frequently asked questions
Hydrostatics is a branch of fluid mechanics that studies fluids at rest in stable equilibrium. It is concerned with studying the equilibrium conditions of a floating body and a submerged body.
Pascal's Law states that any pressure applied to the surface of a fluid is transmitted uniformly throughout the fluid in all directions, without any loss. In other words, the pressure at a point in a fluid at rest is the same in all directions.
The formula for hydrostatic pressure is:
p = ρgh
Where:
- p is the pressure exerted by the liquid (in N.m^-2 or Pa)
- ρ is the density of the liquid (in kg.m^-3, slugs.ft^-3)
- g is the acceleration due to gravity (approximately 9.81 m.s^-2)
- h is the height of the fluid column (in metres)
Archimedes' Principle states that the upthrust or buoyancy force on a submerged object is equal to the weight of the fluid that the object displaces. This applies regardless of the object's shape and whether it is fully or partially submerged.







