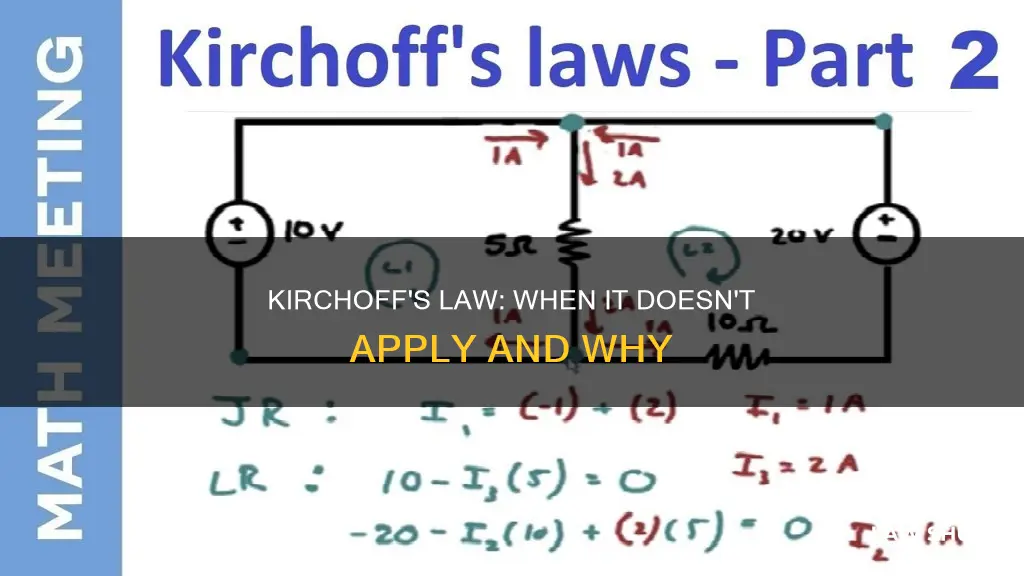
Kirchhoff's circuit laws, widely used in electrical engineering, are two equalities that deal with the current and potential difference (voltage) in the lumped element model of electrical circuits. However, there are certain scenarios where these laws do not hold true. Kirchhoff's laws are accurate for DC circuits and AC circuits with very low frequencies, where the wavelengths of electromagnetic radiation are significantly larger than the circuits. The laws are based on the assumption that the lumped element model applies to the circuit in question. When this model is not applicable, Kirchhoff's laws do not hold. For instance, in high-frequency AC circuits, the lumped element model breaks down due to varying charge densities in conductors, and Kirchhoff's laws become invalid. Additionally, there has been a vigorous debate about the validity of Kirchhoff's laws when energy is dynamically added to a circuit.
| Characteristics | Values |
|---|---|
| When Kirchhoff's law doesn't work | When energy is dynamically added to the circuit |
| Kirchhoff's voltage law doesn't apply | When there is a varying magnetic field enclosed by the circuit being studied |
| Kirchhoff's current law is limited | When there is a voltage source with a very high frequency and effects like parasitic capacitance can no longer be ignored |
| Kirchhoff's law doesn't apply | When the lumped element model is not applicable to the circuit in question |
| Kirchhoff's current law is dependent | On the assumption that the net charge in any wire, junction or lumped component is constant |
| Kirchhoff's voltage law relies | On the fact that the actions of time-varying magnetic fields are confined to individual components, such as inductors |
What You'll Learn
- Kirchhoff's laws don't apply to circuits with a varying magnetic field
- They don't apply to high-frequency AC circuits
- They don't apply when energy is dynamically added to the circuit
- They don't apply when the net charge in a wire, junction or component is not constant
- They don't apply when the circuit is not a lumped network

Kirchhoff's laws don't apply to circuits with a varying magnetic field
Kirchhoff's laws, which deal with the current and potential difference (commonly known as voltage) in electrical circuits, are widely applied in electrical engineering. However, these laws are not without their limitations and do not apply to circuits with a varying magnetic field.
Kirchhoff's current law, or the junction rule, states that for any node (junction) in an electrical circuit, the sum of currents flowing into that node equals the sum of currents flowing out. This principle is based on the conservation of charge.
The second law, or the loop rule, states that the directed sum of potential differences (voltages) around any closed loop is zero. This law is derived from the Maxwell-Faraday equation for static magnetic fields.
When a circuit has a varying magnetic field, the measured voltage becomes non-unique and depends on the branch used to make the measurement. This is because the presence of a time-varying magnetic field means the electric field is no longer conservative, and the usual understanding of the Kirchhoff voltage law is invalidated.
In such cases, the Kirchhoff voltage law can be reconciled by considering the inductance of the loop as an additional circuit element. However, this requires a more detailed model of the circuit, and the Kirchhoff laws in their basic form would not apply.
In summary, Kirchhoff's laws are not universally applicable and do not hold true for circuits with a varying magnetic field. The laws are based on certain assumptions, and when these assumptions are violated, the laws may break down and need to be modified or replaced with alternative approaches.
Fitts' Law: When It Doesn't Apply and Why
You may want to see also

They don't apply to high-frequency AC circuits
Kirchhoff's laws, or Kirchhoff's rules, are two equalities that deal with the current and potential difference (commonly known as voltage) in the lumped element model of electrical circuits. They were first described in 1845 by German physicist Gustav Kirchhoff, and they form the basis for network analysis.
Kirchhoff's laws are accurate for DC circuits and for AC circuits where the wavelengths of electromagnetic radiation are very large compared to the circuits. However, they do not apply to high-frequency AC circuits. Here's why:
Kirchhoff's current law, or KCL, is based on the conservation of charge. It states that for any node (junction) in an electrical circuit, the sum of the currents flowing into that node is equal to the sum of the currents flowing out. This relies on the assumption that the net charge in any wire, junction, or lumped component is constant.
In high-frequency AC circuits, the lumped element model is no longer applicable. The leaked fluxes and varying charge densities in conductors become significant. As a result, the net charge in a wire may not remain constant, and Kirchhoff's current law breaks down.
Kirchhoff's voltage law, or KVL, is based on the conservation of energy. It states that the directed sum of the potential differences (voltages) around any closed loop is zero. This law assumes that the actions of time-varying magnetic fields are confined to individual components, such as inductors, and that the induced electric field produced by an inductor is negligible outside of it.
In high-frequency AC circuits, these assumptions may not hold true. The induced electric fields produced by inductors can leak and become significant. Therefore, Kirchhoff's voltage law may not be valid in such cases.
At high frequencies, it may be necessary to simulate the fields directly using finite element modelling or other techniques rather than relying on Kirchhoff's laws.
HIPAA Laws: Who Are They Really For?
You may want to see also

They don't apply when energy is dynamically added to the circuit
Kirchhoff's laws, which are fundamental to circuit analysis, are based on the law of conservation and the assumption that energy is always conserved. However, a controversy has emerged regarding the applicability of Kirchhoff's laws when energy is dynamically added to a circuit.
The debate was sparked by Mehdi Sadaghdar, an electrical engineer with a substantial online following, who challenged former MIT professor Walter Lewin's assertion that Kirchhoff's voltage law (KVL) does not always hold. In response, Lewin, a physicist, defended the validity of Faraday's law over Kirchhoff's.
The crux of the issue lies in the interpretation of Kirchhoff's law. Lewin's circuit example, which includes a solenoid and two resistors, demonstrates that energizing or turning off the solenoid induces a current in the loop, resulting in voltage differences across the resistors. This contradicts Kirchhoff's law, which states that the sum of voltages around a closed loop should be zero.
Electrical engineers argue that Kirchhoff's law should be understood as a refined principle that incorporates time-dependent circuits and energy addition. They contend that Kirchhoff's law, as originally stated, does not account for dynamic energy input, but it can be adapted to include these factors. Lewin's example is seen as a modelling problem that can be rectified by accounting for the solenoid's effect as a transformer element in the circuit.
In conclusion, while Kirchhoff's laws are foundational in circuit analysis, they do not directly apply when energy is dynamically added to a circuit. However, it is possible to modify and adapt these laws to accommodate such scenarios, ensuring their continued relevance in electrical engineering.
Citizenship Diversity: Laws and Their Applications
You may want to see also

They don't apply when the net charge in a wire, junction or component is not constant
Kirchhoff's laws are based on the law of conservation and can be applied to any circuit with a loop or junction. Kirchhoff's loop rule, also known as Kirchhoff's voltage law (KVL), is derived from the Maxwell-Faraday equation for static magnetic fields. On the other hand, Kirchhoff's junction rule, or Kirchhoff's current law (KCL), is derived from the charge continuity equation.
Kirchhoff's laws do not apply when the net charge in a wire, junction, or component is not constant. This can occur in several situations, such as when there is a varying magnetic field enclosed by the circuit or when there is a voltage source with a very high frequency. In these cases, the presence of time-varying magnetic fields or parasitic capacitance can affect the accuracy of Kirchhoff's laws.
For example, in the case of a varying magnetic field, the measured voltage becomes non-unique and depends on the branch used to measure it. This means that the application of Kirchhoff's voltage law would not yield accurate results. Similarly, when dealing with high-frequency voltage sources, the effects of parasitic capacitance can no longer be ignored, and wires or conductors must be treated as transmission lines. In such cases, Kirchhoff's current law may not hold true, as a current can flow even in an open circuit.
Additionally, Kirchhoff's laws may not apply when energy is dynamically added to a circuit. This has been a subject of debate among engineers and physicists, with some arguing that Kirchhoff's laws fail in such cases, while others suggest that a refined interpretation of the laws can account for time-dependent circuits and energy addition.
It is important to note that Kirchhoff's laws are based on the assumption of a lumped element model, and when this model is not applicable, the laws may not hold. Overall, while Kirchhoff's laws are widely applicable, there are certain scenarios, such as those involving varying magnetic fields, high-frequency sources, or dynamically added energy, where these laws may not provide accurate results.
Vaping vs Smoking: Are Vaping Laws Different?
You may want to see also

They don't apply when the circuit is not a lumped network
Kirchhoff's laws are rooted in the law of conservation and assume that all components of a circuit are concentrated at a single point. This is known as the lumped-element model, which simplifies the description of a circuit's behaviour into a topology.
Kirchhoff's laws do not apply when the circuit in question is not a lumped network. In such cases, the lumped-element model is not applicable, and the laws are invalidated.
Lumped elements are characterised by their size being smaller than the wavelength of the applied signals. They are used in microwave circuits, where transmission lines can be considered lumped elements or distributed elements based on their dimensions.
Distributed elements, on the other hand, have physical dimensions comparable to the operating wavelength. They are distributed over lengths in an RF or microwave circuit.
In distributed elements, physical quantities like voltage and current are dependent on time and space, and partial differential equations are used to describe them. Kirchhoff's laws, which are based on ordinary differential equations, do not account for this variation in quantities over the length of the circuit element.
Therefore, when a circuit is not a lumped network, and instead contains distributed elements, Kirchhoff's laws are not applicable.
Understanding Snells Law in a Single Medium
You may want to see also
Frequently asked questions
Kirchhoff's laws don't apply when energy is dynamically added to the circuit. They also don't apply when the net charge in any wire, junction or lumped component is not constant, such as in high-frequency AC circuits.
Kirchhoff's first law, or Kirchhoff's current law, states that the sum of currents flowing into a node is equal to the sum of currents flowing out of that node.
Kirchhoff's second law, or Kirchhoff's voltage law, states that the directed sum of the potential differences (voltages) around any closed loop is zero.







