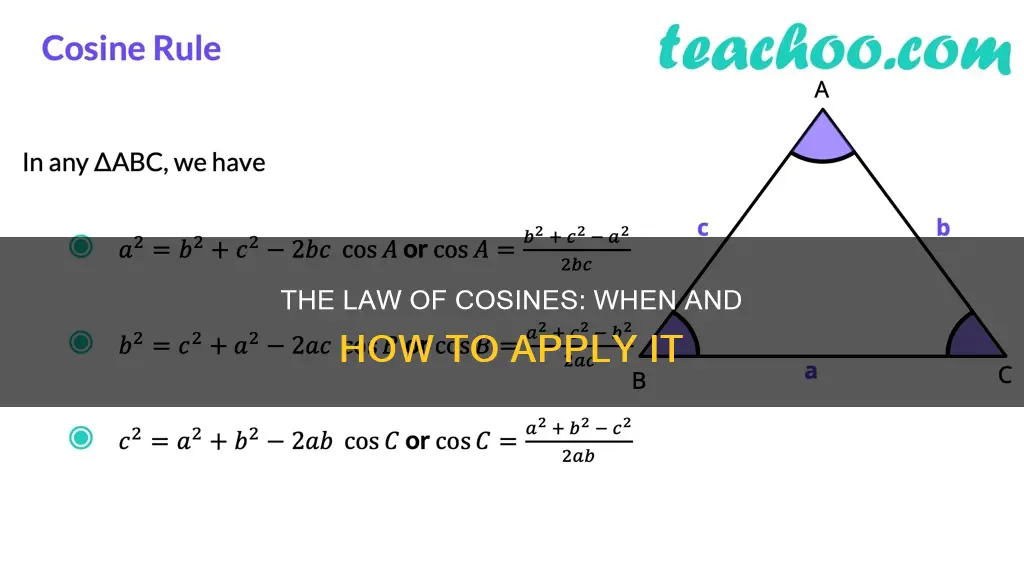
The law of cosines, also known as the cosine rule or cosine formula, is used in trigonometry to relate the lengths of the sides of a triangle to the cosine of one of its angles. It is applied when you want to find the third side of a triangle when you know the lengths of the other two sides and the angle between them, or when you want to find the angles of a triangle when you know the lengths of all three sides. The formula for the law of cosines is:
c^2 = a^2 + b^2 - 2ab cos(C)
| Characteristics | Values |
|---|---|
| What is the Law of Cosines used for? | Finding the third side of a triangle when we know the lengths of the other two sides and the angle between them |
| What is the Law of Cosines? | A trigonometric rule that relates the lengths of the sides of a triangle to the cosine of one of its angles |
| When should the Law of Cosines be used? | When we want to find the third side of a triangle when we know two sides and the angle between them or when we know all three sides and want to find one of the angles |
| Can we use the Law of Cosines for all triangles? | Yes, it can be used for all types of triangles |
What You'll Learn
- Finding the third side of a triangle when two sides and the angle between them are known
- Finding the angles of a triangle when all three sides are known
- Finding the third side of a triangle when two sides and an angle opposite to one of them is known
- Finding the angles of a triangle when the lengths of all three sides are known
- Finding the third side of a triangle when the lengths of the other two sides and the angle between them are known

Finding the third side of a triangle when two sides and the angle between them are known
The law of cosines, also known as the cosine formula or cosine rule, is used in trigonometry to relate the lengths of the sides of a triangle to the cosine of one of its angles.
The law of cosines is applied when you want to find the third side of a triangle when the lengths of the other two sides and the angle between them are known.
The formula for the law of cosines is:
C^2 = a^2 + b^2 - 2ab cos(C)
Where:
- A, b, and c are the sides of the triangle
- C is the unknown side you want to find
- A and b are the known sides
- C is the angle between the known sides a and b
For example, let's say you have a triangle with sides a = 3 cm, b = 5 cm, and an angle C = 60 degrees between them. To find the third side, c, you can use the law of cosines as follows:
C^2 = 3^2 + 5^2 - 2 * 3 * 5 * cos(60)
C^2 = 9 + 25 - 30 * cos(60)
C^2 = 34 - 30 (as cos(60) is approximately -0.5)
C^2 = 4
C = 2 cm
So, the third side of the triangle is 2 cm.
The law of cosines is a versatile tool in trigonometry, applicable to all types of triangles, and it helps determine unknown sides or angles when sufficient information is provided.
Anti-Sodomy Laws: Were Lesbians Included or Excluded?
You may want to see also

Finding the angles of a triangle when all three sides are known
The law of cosines, also known as the cosine formula or cosine rule, relates the lengths of the sides of a triangle to the cosine of one of its angles.
The law of cosines is used to solve a triangle when all three sides or two sides and their included angle are given.
When all three sides of a triangle are known, the law of cosines can be used to find the angles. This is known as solving an SSS triangle, where SSS stands for side, side, side.
The formula for the law of cosines is:
Cos(C) = (a^2 + b^2 - c^2) / (2ab)
Cos(A) = (b^2 + c^2 - a^2) / (2bc)
Cos(B) = (c^2 + a^2 - b^2) / (2ca)
These are all the same formula, just with different labels.
To solve for the angles, follow these steps:
Use the law of cosines to find one of the angles. It doesn't matter which one. For example, to find angle A:
Cos(A) = (b^2 + c^2 - a^2) / (2bc)
Use the law of cosines again to find another angle. For example, to find angle B:
Cos(B) = (c^2 + a^2 - b^2) / (2ca)
Finally, find the last angle by using the fact that the angles of a triangle add up to 180 degrees.
For example, if you found angle A to be 75.5 degrees and angle B to be 46.6 degrees, then:
Angle C = 180 degrees - 75.5 degrees - 46.5 degrees = 57.9 degrees
Now you have solved the triangle and found all its angles.
Example
Consider a triangle with sides a = 6, b = 7, and c = 8.
Use the law of cosines to find angle A:
Cos(A) = (b^2 + c^2 - a^2) / (2bc)
Cos(A) = (7^2 + 8^2 - 6^2) / (2 * 7 * 8)
Cos(A) = (49 + 64 - 36) / 112
A = 75.5 degrees (to one decimal place)
Use the law of cosines to find angle B:
Cos(B) = (c^2 + a^2 - b^2) / (2ca)
Cos(B) = (8^2 + 6^2 - 7^2) / (2 * 8 * 6)
Cos(B) = (64 + 36 - 49) / 112
B = 46.6 degrees (to one decimal place)
Find angle C using the fact that angles of a triangle add up to 180 degrees:
Angle C = 180 degrees - 75.5 degrees - 46.5 degrees = 57.9 degrees
So, for the triangle with sides a = 6, b = 7, and c = 8, the angles are: A = 75.5 degrees, B = 46.6 degrees, and C = 57.9 degrees.
Joshua Law: Applicability to 17-Year-Olds Explored
You may want to see also

Finding the third side of a triangle when two sides and an angle opposite to one of them is known
The law of cosines, also known as the cosine formula or cosine rule, is a trigonometric formula that relates the lengths of a triangle's sides to the cosine of one of its angles.
The law of cosines states:
C^2 = a^2 + b^2 - 2ab x cos(C)
Where:
- C, a, and b are the sides of the triangle
- C is the angle between sides a and b
This formula can be used to find the third side of a triangle when two sides and the angle between them are known.
For example, if we know sides a and b, and the angle between them C, we can use the law of cosines to find the length of the third side, c:
C = √(a^2 + b^2 - 2ab x cos(C))
This formula is particularly useful when dealing with non-right triangles, as it provides a more generalised version of the Pythagorean theorem.
It's important to note that the law of cosines is typically more challenging to use and remember than other formulas, such as the law of sines. However, it is sometimes necessary to apply it to solve complex problems.
In addition to finding the third side, the law of cosines can also be used to determine the angles of a triangle when the three sides are known. By rearranging the formula, we can find the angle C:
C = arccos((a^2 + b^2 - c^2) / (2ab))
This demonstrates the versatility of the law of cosines in solving triangles.
Understanding EEOC Laws: Comprehensive Guide to Equal Employment
You may want to see also

Finding the angles of a triangle when the lengths of all three sides are known
The law of cosines is a formula that relates the lengths of the sides of a triangle to the cosine of one of its angles. The formula is:
`cos(C) = a^2 + b^2 - c^2 / 2ab`
Or
`c^2 = a^2 + b^2 - 2abcos(C)`
Where `a`, `b`, and `c` are the sides of the triangle and `C` is the angle opposite side `c`.
The law of cosines can be used to find the angles of a triangle when the lengths of all three sides are known. This is known as solving an SSS (side-side-side) triangle. Here are the steps to solve an SSS triangle:
Use the law of cosines to calculate one of the angles. It doesn't matter which one you choose. For example, let's find angle `A` in a triangle with sides `a = 8`, `b = 6`, and `c = 7`:
`cos(A) = (b^2 + c^2 - a^2) / (2*b*c)`
`cos(A) = (6^2 + 7^2 - 8^2) / (2*6*7)`
`cos(A) = (36 + 49 - 64) / 84`
`A = cos^-1(0.574) = 75.5°`
Use the law of cosines again to find another angle. For example, let's find angle `B`:
`cos(B) = (c^2 + a^2 - b^2) / (2*c*a)`
`cos(B) = (7^2 + 8^2 - 6^2) / (2*7*8)`
`cos(B) = (49 + 64 - 36) / 112`
`B = cos^-1(0.643) = 46.6°`
Finally, find the third angle using the fact that the angles of a triangle add up to 180°. For the above example:
`C = 180° - 75.5° - 46.5° = 57.9°`
And that's it! You've solved the triangle and found all three angles. You can use the same method for any triangle where you know all three sides. Just remember to use the appropriate version of the law of cosines formula depending on which angle you're trying to find.
HIPAA Laws: Do They Apply at the VA?
You may want to see also

Finding the third side of a triangle when the lengths of the other two sides and the angle between them are known
The law of cosines, also known as the cosine formula or cosine rule, is a trigonometric concept that relates the lengths of a triangle's sides to the cosine of one of its angles. This principle is particularly useful when solving triangles, and it can be applied in various scenarios, including finding the third side of a triangle when the lengths of the other two sides and the angle between them are known.
To find the third side of a triangle when the other two sides and the included angle are given, you can use the law of cosines formula:
C^2 = a^2 + b^2 - 2ab * cos(C)
In this formula, 'a' and 'b' represent the lengths of the known sides, and 'C' is the measure of the angle between them. By inputting the given values and solving for 'c', you can determine the length of the third side.
For example, let's say you have a triangle with sides "a" and "b", and you know that "a" is 5 units long, "b" is 7 units long, and the angle between them, angle "C", is 49 degrees. To find the length of the third side, "c", you would plug these values into the formula:
C^2 = 5^2 + 7^2 - 2 * 5 * 7 * cos(49°)
Now, calculate the value of "cos(49°)" using a calculator, and then perform the multiplication and subtraction. Finally, take the square root of the result to find the length of the third side, "c".
This application of the law of cosines is particularly useful when dealing with non-right triangles, as it allows you to find the unknown side length without relying on the Pythagorean theorem, which only applies to right triangles.
Understanding Work Laws: 1099 Employee Rights and Regulations
You may want to see also
Frequently asked questions
The law of cosines is used to determine the third side of a triangle when we know the lengths of the other two sides and the angle between them.
The law of cosines is not just restricted to right triangles, and it can be used for all types of triangles where we need to find any unknown side or unknown angle.
The cosine law is used to find the unknown side of a triangle when the length of the other two sides is given and the angle between the two known sides.
The formula to find the unknown angles using the cosine law is: cos α = [b^2 + c^2 – a^2] / (2bc).
The law of cosines signifies the relation between the lengths of sides of a triangle with respect to the cosine of its angle.







