Closed Systems: Overcoming Entropy's Inevitable Grip?

The second law of thermodynamics states that the entropy of a closed system can never decrease. In other words, natural processes only run in one direction and cannot be reversed without increasing the entropy of the system's surroundings. This has sparked debates about whether the second law applies only to isolated systems or if it can be extended to open systems by including the environment in the system's definition. The Poincaré recurrence theorem, which considers the microscopic state of an isolated system, has been perceived as contradicting the second law. However, it is simply a model of thermodynamic equilibrium. The applicability of the second law to open systems and the concept of equilibrium raise questions about whether a closed system can ever overcome the second law of thermodynamics.

Entropy and the Second Law

The Second Law of Thermodynamics is concerned with the direction of natural processes. It states that a natural process runs in only one direction and is not reversible. In other words, the state of a natural system can be reversed, but not without increasing the entropy of the system's surroundings. This means that both the state of the system and its surroundings cannot be fully reversed without the destruction of entropy. This law is particularly relevant to closed systems.

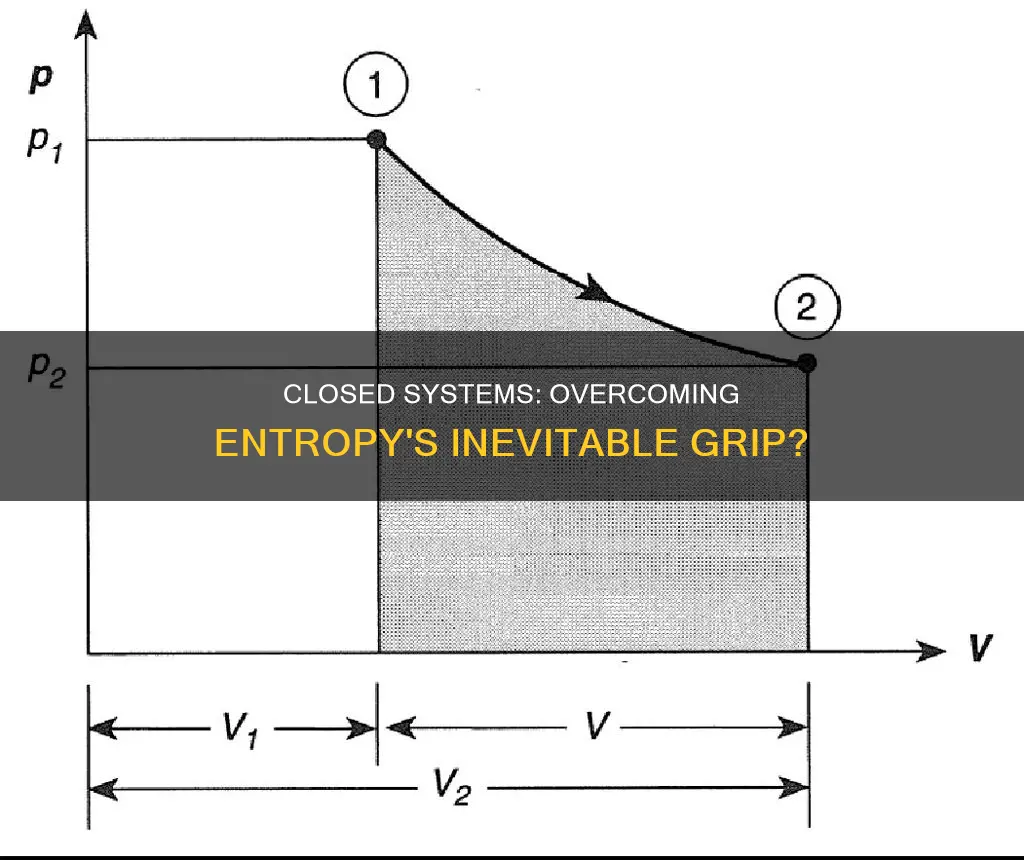
The Second Law of Thermodynamics applies to closed systems, where the entropy, S, of the system can never decrease in any process. If the process is reversible, the entropy of the closed system remains constant. However, if the process is irreversible, the entropy of the closed system increases. This is because, as the number of microstates increases, so does entropy. The Second Law of Thermodynamics assures that the total change in entropy will always be positive.

The Poincaré recurrence theorem may be considered a contradiction to the Second Law of Thermodynamics. This theorem describes a theoretical isolated physical system that will, after a sufficiently long time, return to a state very close to its initial one. However, it is important to note that the recurrence time is exceedingly long, likely longer than the life of the universe.

While the Second Law is commonly associated with isolated systems, it can also be applied to open systems by expanding the system's definition to include the rest of the universe, thereby making it closed. For example, a solar panel powering a refrigerator violates the Second Law when considered as an open system, but when the sun is included as the energy source, the system becomes closed, and the Second Law is upheld.

Reversible vs Irreversible Processes

The second law of thermodynamics states that a natural process can only run in one direction and is not reversible. In other words, the state of a natural system can be reversed, but not without increasing the entropy of the system's surroundings. This means that the system and its environment cannot return to their initial states.

A reversible process is one in which both the system and its environment can be restored to their exact prior states by reversing the process. This is a challenging feat to achieve, as it is relatively simple to restore a system to its original state, but it is difficult to do so without altering something in its surroundings. For example, an ideal gas can be compressed back to its original volume with a piston, but the process cannot be reversed without changing something in the surroundings, such as adding heat.

The necessary condition for a reversible process is the quasi-static requirement, which is rarely met in reality. Isothermal and adiabatic processes are theoretically reversible, as the system is always in an equilibrium state and can move forward or backward along the given curves. However, in practical terms, a system with a large number of molecules or particles experiences numerous collisions that tend to erase any memory of the initial trajectory of each particle, making the process irreversible.

An irreversible process is one in which the system and its environment cannot return to their exact prior states. Irreversibility implies that there is a form of dissipative work, and information is lost during the operation. This loss of information prevents the system from returning to its initial state, even by reversing the operation. Examples of irreversible processes include the expansion of an ideal gas, which is not a quasi-static process, and spontaneous heat flow between macroscopic objects with a large number of molecules.

Isolated vs Closed Systems

The first law of thermodynamics defines the internal energy of a thermodynamic system and expresses its change for a closed system in terms of work and heat. It is linked to the law of conservation of energy, which states that energy is neither created nor destroyed but is converted from one form to another. The second law of thermodynamics is concerned with the direction of natural processes and asserts that a natural process runs only in one sense and is not reversible.

An isolated system is one in which neither matter nor energy can enter or exit. In other words, energy and quantity of matter are conserved. This is in contrast to a closed system, in which matter cannot enter or exit, but energy can. For example, a closed system can lose heat through radiation or conduction. The Poincaré recurrence theorem considers a theoretical microscopic description of an isolated physical system. It states that after a sufficiently long time, the system will return to a state very close to the initial one. However, the recurrence time is exceedingly long, likely longer than the life of the universe.

The second law of thermodynamics states that a natural process runs only in one direction and cannot be reversed. This implies that the total entropy of a system and its surroundings cannot be reduced. However, it is important to note that this law applies specifically to spontaneous processes. In some cases, non-spontaneous processes can be used to decrease the entropy of a system. For example, a heat pump can reverse the heat flow in a closed system, but the reversal process and the original process both cause entropy production, increasing the entropy of the surroundings.

While the second law of thermodynamics is a fundamental principle, there have been some proposed exceptions. For example, the Poincaré recurrence theorem describes a scenario in which an isolated system returns to a state very close to its initial one after a sufficiently long time. However, it is important to note that the time required for this recurrence is likely longer than the life of the universe. Additionally, the concept of a Maxwell's demon, a hypothetical entity that can manipulate individual molecules, has been proposed as a potential violation of the second law. However, further analysis has shown that the presence of a Maxwell's demon would increase, rather than decrease, the overall entropy of a system.

Carathéodory's Principle

The Second Law of Thermodynamics states that the entropy of a closed system can never decrease. In other words, the state of entropy of the entire universe, as an isolated system, will always increase over time. This is often referred to as the "arrow of time", where certain things happen in one direction and not the other.

Constantin Carathéodory, a Greek mathematician, formulated his own statement of the Second Law, known as Carathéodory's Principle, in 1909. Carathéodory's Principle can be formulated as: "In every neighbourhood of any state S of an adiabatically enclosed system, there are states inaccessible from S." This statement introduced the concept of adiabatic accessibility for the first time and laid the foundation for a new subfield of classical thermodynamics, known as geometrical thermodynamics.

Combining Carathéodory's Principle with Planck's principle, we can understand the Second Law of Thermodynamics for a closed system. The entropy balance equation for a closed system can be written as:

> S_2-S_1=∫_1^2δQ/T+S_gen≅ΣQ_k/T_k+S_gen

This equation represents the entropy transfer caused by heat transfer between the system and its surroundings.

Gibbs Free Energy

The second law of thermodynamics states that a natural process can only occur in one direction and is not reversible. In other words, while the state of a natural system can be reversed, it cannot be reversed without increasing the entropy of the system's surroundings. This means that the state of the system and its surroundings cannot be fully reversed without the destruction of entropy.

The concept of Gibbs free energy, originally called "available energy", was developed in the 1870s by the American scientist Josiah Willard Gibbs. It combines enthalpy and entropy into a single value, with the change in free energy, ΔG, being equal to the sum of the enthalpy plus the product of the temperature and entropy of the system.

In the context of a closed system, the second law of thermodynamics can be understood through the Clausius inequality, ΔS > Q/Tsurr, which transforms into a condition for the change in Gibbs free energy, dG < 0. This means that for a spontaneous chemical process in a closed system at constant temperature and pressure without non-PV work, the change in Gibbs free energy must be negative.

In conclusion, while a closed system cannot fully overcome the second law of thermodynamics as the natural process will still be irreversible, it can be manipulated through the input of external energy to make the process spontaneous in either direction. This is reflected in the sign of ΔG, which indicates the direction of a chemical reaction and whether it is spontaneous or not.

Frequently asked questions