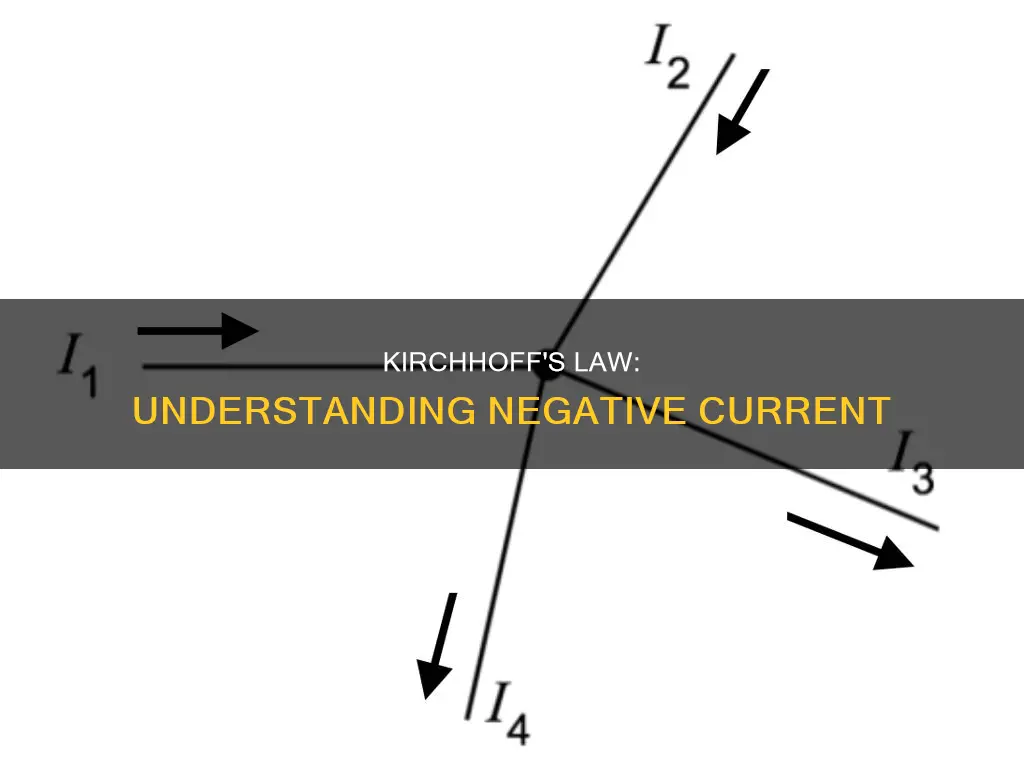
Kirchhoff's laws are two simple relationships that can be used to determine the value of currents in circuits. They are named after German physicist Gustav Robert Kirchhoff, who formulated them. The first law, or the junction rule, states that the sum of currents entering a junction is equal to the sum of currents leaving that junction. The second law, or the loop rule, states that the algebraic sum of changes in potential around any closed circuit path (loop) must be zero. In some cases, the current in a circuit may flow in a direction opposite to the indicated direction, resulting in a negative value for the current. This negative sign indicates that the current is flowing in the opposite direction.
| Characteristics | Values |
|---|---|
| Can current be negative in Kirchhoff's law? | Yes, if the value of E2 is greater than E1, the solution for the current i would be a negative value. |
| What does a negative current value indicate? | That the current in the circuit is flowing in the opposite direction. |
| What is Kirchhoff's first law? | The sum of currents entering a junction equals the sum of currents leaving that junction. |
| What is Kirchhoff's second law? | The algebraic sum of changes in potential around any closed circuit path (loop) must be zero. |
| What is the loop rule? | Energy remains conserved, which means that when a charge travels around any loop in a network to return to where it started, its potential energy (qV) should be the same as when it previously occupied that position. |
What You'll Learn
- Kirchhoff's first rule, also known as the junction rule, states that the sum of currents entering a junction equals the sum of currents leaving that junction
- Kirchhoff's second rule, or the loop rule, states that the sum of the voltage drops around any closed loop is zero
- Kirchhoff's laws can be applied to circuits with multiple loops, but the algebra becomes more complex as the number of loops increases
- The direction of current flow can be determined by solving a system of equations, with negative values indicating a direction opposite to the labelled one
- Kirchhoff's rules are based on the conservation of charge and energy and can be applied to any circuit, including those with resistors and capacitors

Kirchhoff's first rule, also known as the junction rule, states that the sum of currents entering a junction equals the sum of currents leaving that junction
The junction rule is based on the conservation of electric charge, which dictates that the total charge flowing into a junction must be equal to the total charge flowing out. In other words, the algebraic sum of all currents at a junction point in a circuit network is always zero. This can be expressed mathematically as Σ I_IN = Σ I_OUT, where Σ I_IN represents the sum of currents entering the junction, and Σ I_OUT represents the sum of currents leaving it.
For example, consider a scenario where two currents, I1 and I2, enter a junction, and a single current, IT, leaves it. Kirchhoff's first rule would dictate that I1 + I2 = IT. By assuming consistent current directions at each node, we can analyse the circuit and determine the actual current directions. This principle can be applied to any number of junctions or nodes within a circuit.
Kirchhoff's first rule is particularly useful when dealing with complex circuits that contain multiple loops or junctions. By applying this rule, we can gain insights into the behaviour of electric currents within the circuit, ensuring that charge is conserved at each junction. This rule, along with Kirchhoff's second rule (the loop rule), provides a powerful framework for understanding and analysing electric circuits.
While Kirchhoff's laws are commonly applied to circuits with consistent current directions, they can also accommodate scenarios with negative currents. In such cases, a negative sign indicates that the current flows in the opposite direction to the indicated or expected path. This notion of negative currents adds flexibility to Kirchhoff's rules, allowing them to be applied to a broader range of circuit configurations.
Contractual Obligations: Can They Ignore the Law?
You may want to see also

Kirchhoff's second rule, or the loop rule, states that the sum of the voltage drops around any closed loop is zero
Kirchhoff's rules are based on the conservation of charge and energy. They are used to determine the value of currents in circuits and can be applied to any circuit, regardless of complexity.
Kirchhoff's second rule, also known as the loop rule, states that the sum of the voltage drops around any closed loop is zero. This means that when a charge travels around a loop in a network and returns to its starting point, its potential energy remains conserved. In other words, the energy supplied by the emf source is equal to the energy converted by the circuit's resistance, and the kinetic energy of the charge remains unchanged.
The loop rule can be applied to circuits with multiple loops, and it helps to simplify complex problems. When using the loop rule, it is important to choose a loop direction (clockwise or counterclockwise) and a starting point. The procedure involves following the chosen direction and constructing a sum of the voltage drops. If the loop journey crosses from the negative terminal to the positive terminal, a positive value equal to the battery's emf should be added since this represents an increase in potential.
It is worth noting that the presence of negative currents or voltages in a circuit does not violate Kirchhoff's rules. Negative values simply indicate that the actual direction of the current or voltage is opposite to the indicated or reference direction. This can occur in situations where the current alternates its direction periodically, such as in alternating current (AC) circuits.
City Laws: Can They Override State Laws?
You may want to see also

Kirchhoff's laws can be applied to circuits with multiple loops, but the algebra becomes more complex as the number of loops increases
Kirchhoff's rules, named after German physicist Gustav Kirchhoff, are two simple relationships that can be used to determine the value of currents in circuits. They are founded on the two most broadly applicable principles in physics: conservation laws. The first rule, also known as the junction rule, states that the sum of currents entering a junction equals the sum of currents leaving that junction. The second rule, or the loop rule, states that the algebraic sum of changes in potential around any closed circuit path (loop) must be zero.
These rules can be applied to circuits with multiple loops, but as the number of loops increases, the algebra becomes more complex. For instance, consider a circuit with three unknown currents, I1, I2, and I3. Applying the junction rule at a junction point will yield an equation with three unknowns, requiring the application of the loop rule to solve for the unknown currents. This involves choosing a direction to go around the loop and carefully determining the signs of the potential changes for each element. The process becomes more intricate with each additional loop, necessitating careful checking and rechecking of calculations.
Kirchhoff's rules are particularly useful for analysing complex circuits that cannot be simplified using series-parallel techniques. They can be applied to any circuit, regardless of its complexity, and are foundational principles in circuit analysis. However, the algebra involved in applying these rules to circuits with multiple loops can become tedious and challenging.
Negative currents can arise in Kirchhoff's circuit due to the direction of the current. If the calculated direction of the current is opposite to the indicated direction, it will be considered negative. This does not violate Kirchhoff's laws, as they allow for currents in opposite directions. For example, in the United States, the current alternates its direction in electric conducting wires, flowing in one direction and then the opposite direction, 60 times per second.
Marriage Laws: Congress' Legislative Powers Explored
You may want to see also

The direction of current flow can be determined by solving a system of equations, with negative values indicating a direction opposite to the labelled one
Kirchhoff's laws are two simple relationships that can be used to determine the value of currents in circuits. The first law, also known as Kirchhoff's Junction Rule, states that the sum of currents entering a junction is equal to the sum of currents leaving that junction. The second law, or Kirchhoff's Loop Rule, states that the algebraic sum of currents in a closed loop is zero. These laws can be applied to circuits with multiple loops, although the complexity of the algebra increases as the circuits become more intricate.
When applying Kirchhoff's laws to a circuit, the direction of current flow is crucial. The actual direction of current through a specific circuit element is typically unknown until the analysis is completed. Therefore, reference directions for currents are often arbitrarily assigned. This means that the direction of current flow is chosen as a starting point for analysis, and the resulting calculations determine whether the current flows in the same or opposite direction of the chosen reference.
The direction of current flow can be determined by solving a system of equations, where negative values indicate a direction opposite to the labelled one. For example, consider a circuit with two sources of electromotive forces, E1 and E2, and two resistances, R1 and R2. By applying Kirchhoff's second law and Ohm's law, we can set up a system of equations to find the current, i. If the solution for i is negative, it implies that the actual direction of current flow is opposite to the chosen reference direction.
In another example, a circuit diagram may indicate a voltage source with a negative value. This non-standard notation can be confusing, as it implies that the polarity is opposite to the reference polarity. In this case, the current direction is opposite to the indicated direction, flowing out of the positively labelled terminal.
It is important to note that the convention for current direction is based on the movement of positive charges, also known as conventional current. In metals, where the charge carriers are negatively charged electrons, the conventional current is in the opposite direction of the overall electron movement. This convention was established to solve practical problems, and it yields correct results when analyzing circuits and applying theorems.
How Congress Can Pass Laws Without Senate Approval
You may want to see also

Kirchhoff's rules are based on the conservation of charge and energy and can be applied to any circuit, including those with resistors and capacitors
Kirchhoff's rules are a set of laws that can be used to analyse any circuit, from simple to complex, and are based on the conservation of charge and energy. They are named after their inventor, Gustav Kirchhoff (1824-1887). Kirchhoff's rules are particularly useful for complex circuits that cannot be simplified by combining elements in series and parallel.
Kirchhoff's first rule, also known as the junction rule, states that the sum of currents entering a junction equals the sum of currents leaving that junction. This is commonly referred to as Kirchhoff's first law or Kirchhoff's current law (KCL). This rule is an application of the principle of conservation of electric charge.
Kirchhoff's second rule, also known as the loop rule, states that the algebraic sum of changes in potential around any closed circuit path (loop) must be zero. This is Kirchhoff's voltage law (KVL) and is based on the principle of conservation of energy. This rule is particularly useful for series circuits, as they also act as voltage dividers.
Kirchhoff's rules can be applied to circuits with various components, including resistors and capacitors. For instance, consider a circuit with a capacitor and a resistor connected to a 50-volt battery. Initially, the capacitor has no charge, and the initial current can be calculated using Ohm's law, V = iR. As the capacitor begins to charge, a positive charge accumulates on one plate, while a negative charge accumulates on the other.
Kirchhoff's laws can also be applied to circuits with negative currents. A negative current indicates that the current is flowing in the opposite direction to the one indicated. For example, in alternating current (AC) systems, the direction of current flow alternates, resulting in both positive and negative currents in the circuit.
Congress' Power: Can They Repeal Previous Laws?
You may want to see also
Frequently asked questions
Yes, current can be negative in Kirchhoff's law. A negative current indicates that the actual direction of the current is opposite to the indicated direction.
Kirchhoff's first rule, also known as the junction rule, states that the sum of currents entering a junction is equal to the sum of currents leaving that junction.
Kirchhoff's second rule, also known as the loop rule, states that the algebraic sum of changes in potential around any closed circuit path or loop must be zero.
Kirchhoff's rules are used to analyze complex circuits that cannot be simplified using Ohm's law or series-parallel techniques. They are based on the conservation of charge and energy.
The direction of the current in a circuit is indicated by the current's positive value. A positive value means the current flows in the direction of the circuit's orientation.







