The natural logarithm, often denoted as ln, is the logarithm with a base of e, where e is a mathematical constant with a value of approximately 2.71828. Natural logs are used extensively in mathematics and economics, particularly in scenarios involving compound interest, growth equations, and decay equations. The rules of logarithms, such as the product rule, quotient rule, and power rule, apply equally to natural logs. For example, the natural log of the product of two numbers is the sum of the natural logs of each number, and the natural log of the quotient of two numbers is the difference in their natural logs. These rules can be used in combination to solve more complex problems involving natural logs.
| Characteristics | Values |
|---|---|
| General rule | Given the exponential equation ex=y, the associated logarithmic equation is ln(y)=x |
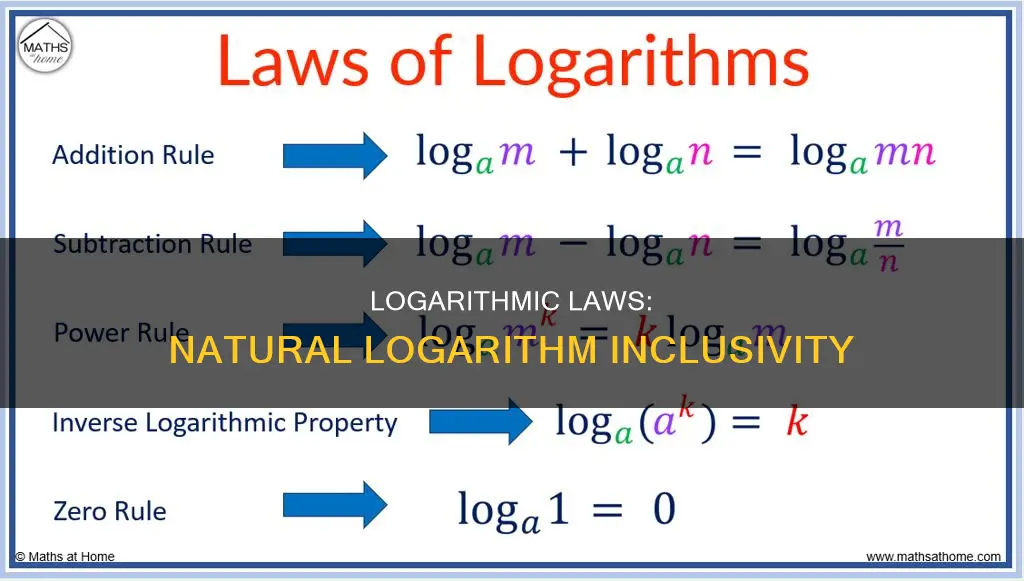
| Product rule | ln(xy) = ln(x) + ln(y) |
| Quotient rule | ln(x/y) = ln(x) - ln(y) |
| Power rule | ln(x^n) = nln(x) |
What You'll Learn
- The natural log of the multiplication of two numbers is the sum of the ln of those numbers
- The natural log of the division of two numbers is the difference of the ln of those numbers
- The natural log of the reciprocal of a number is the opposite of the ln of that number
- The natural log of a number raised to the power of another number is the latter number multiplied by the ln of the former
- The natural log of a number is the same as the log of that number, with base e

The natural log of the multiplication of two numbers is the sum of the ln of those numbers
The logarithm laws do apply to natural logs. Natural logs, or logarithms with a base of "e", follow the same rules as common logarithms.
The product rule for logarithms states that the logarithm of the product of two terms is equal to the sum of the logarithms of the individual terms. In other words, the rule states that:
> logb mn = logb m + logb n
This rule can be applied to natural logs, as well. The natural log of the multiplication of two numbers is the sum of the ln of those numbers. This can be written as:
> ln(xy) = ln(x) + ln(y)
For example, let's say we want to find the value of ln(8)(6). Using the product rule for natural logs, we can rewrite this as:
> ln(8)(6) = ln(8) + ln(6)
Now, we can use a calculator to find the approximate values of these natural logs. So, ln(8)(6) is equal to the sum of the approximate values of ln(8) and ln(6).
> ln(8)(6) ≈ 2.08 + 1.79
Therefore, the value of ln(8)(6) is approximately 3.87.
The product rule for natural logs allows us to simplify expressions involving the multiplication of two numbers. By breaking down the multiplication into the sum of the natural logs of the individual numbers, we can more easily evaluate the expression. This rule is particularly useful when working with complex expressions or when trying to solve equations involving natural logs.
In addition to the product rule, natural logs also follow the quotient rule and the power rule. The quotient rule states that the natural log of the division of two numbers is the difference between the ln of the numbers. The power rule states that the natural log of a number raised to a power is equal to the power multiplied by the ln of the number.
By applying these rules, we can manipulate and simplify expressions involving natural logs. These rules are derived from the rules of exponents, as logarithms are simply the inverse of exponents. The natural log, specifically, is the inverse of the mathematical constant "e", which is approximately equal to 2.71828.
Knife Laws and Machetes: What's the Verdict?
You may want to see also

The natural log of the division of two numbers is the difference of the ln of those numbers
Logarithm laws do apply to natural logs. The natural logarithm, or "ln", is the logarithm with base "e". The rules of logs are the same for all logarithms, including the natural logarithm.
The log of a quotient is the difference of the logs. This means that the log of a division of two numbers is equal to the difference between the logs of those two numbers.
For example, if we want to find the natural log of 7 divided by 4, we would subtract the natural log of 4 from the natural log of 7:
Ln(7/4) = ln(7) - ln(4)
This is one of the four main rules of natural logs that you need to know when working with them.
Limits and Laws: When One Limit Doesn't Exist
You may want to see also

The natural log of the reciprocal of a number is the opposite of the ln of that number
The natural logarithm, or ln, is the inverse of e. The letter 'e' represents a mathematical constant known as the natural exponent, with a value of approximately 2.71828. The natural logarithm of a number is its logarithm to the base of e.
The natural logarithm follows the same rules as other logarithms. These include the product rule, quotient rule, and power rule. The product rule states that the logarithm of the product of two terms is equal to the sum of the logarithms of the individual terms. The quotient rule states that the logarithm of the quotient of two terms is equal to the difference of the logarithms of the individual terms. The power rule states that the logarithm of a number raised to a power is equal to the power times the logarithm of the number.
The natural logarithm of the reciprocal of a number is indeed the opposite of the natural logarithm of that number. This is a result of the quotient rule, which can be expressed as:
> ln(x/y) = ln(x) - ln(y)
In this formula, if we set y = 1, we get:
> ln(x/1) = ln(x) - ln(1)
Since ln(1) = 0, this simplifies to:
> ln(x/1) = ln(x) - 0
> ln(x) = ln(x/1)
Therefore, ln(1/x) = -ln(x).
Retroactive Law Application: Litigation's Impact Explored
You may want to see also

The natural log of a number raised to the power of another number is the latter number multiplied by the ln of the former
Logarithm laws do apply to natural logs. The natural logarithm, or "ln", is the inverse of "e", a mathematical constant with a value of approximately 2.71828. Natural logs are used as a shortcut to write and calculate the logarithm with a base of "e". The rules of logs are the same for all logarithms, including natural logarithms.
The natural log of a number raised to the power of another number follows the power rule of logarithms, which states that:
> The natural log of a number raised to the power of another number is the latter number multiplied by the ln of the former.
In other words, if we have "x" raised to the power of "y", the natural logarithm of this expression, ln(x^y), is equal to y * ln(x). For example, if x = 5 and y = 2, we get:
> ln(5^2) = 2 * ln(5)
Using a calculator, we can find that ln(5) is approximately 1.609, so:
> ln(5^2) = 2 * 1.609 = 3.218
So, the natural logarithm of 5 raised to the power of 2 is approximately 3.218.
The power rule for logarithms is derived from the rules of exponents. Since taking a logarithm is the opposite of exponentiation, we can derive the basic rules for logarithms from the basic rules for exponents. The power rule for logarithms states that if the argument of a logarithm has an exponent, then the exponent can be brought outside the logarithm. This rule applies to natural logs as well, so we can rewrite ln(x^y) as y * ln(x).
Meeting Laws and Nonprofits: Understanding Compliance Requirements
You may want to see also

The natural log of a number is the same as the log of that number, with base e
Logarithm laws do apply to natural logs. The natural log, or ln, is the inverse of e, a mathematical constant with a set value of approximately 2.71828. The natural log of a number is the same as the log of that number, with base e. This is because the natural logarithm is simply the logarithm of base e. In other words, loge(a) is the same as lna.
The rules for natural logarithms are the same as the rules for logarithms of other bases. The rules are as follows:
- The natural log of the multiplication of x and y is the sum of the ln of x and ln of y: ln(xy) = ln(x) + ln(y).
- The natural log of the division of x and y is the difference of the ln of x and ln of y: ln(x/y) = ln(x) - ln(y).
- The natural log of x raised to the power of y is y times the ln of x: ln(xy^) = y*ln(x).
- The natural log of the reciprocal of x is the opposite of the ln of x: ln(1/x) = -ln(x).
These rules can be used in combination to solve problems with natural logs.
Finland's Laws: Universal or Selective Application?
You may want to see also
Frequently asked questions
No, the rules for natural logs are the same as those for other bases.
The natural log of the product of two numbers is the sum of the natural logs of the individual numbers. For example, ln(8)(6) = ln(8) + ln(6).
The natural log of the quotient of two numbers is the difference between the natural logs of the individual numbers. For example, ln(7/4) = ln(7) - ln(4).
The natural log of a number raised to a power is equal to the power multiplied by the natural log of the number. For example, ln(5^2) = 2 * ln(5).