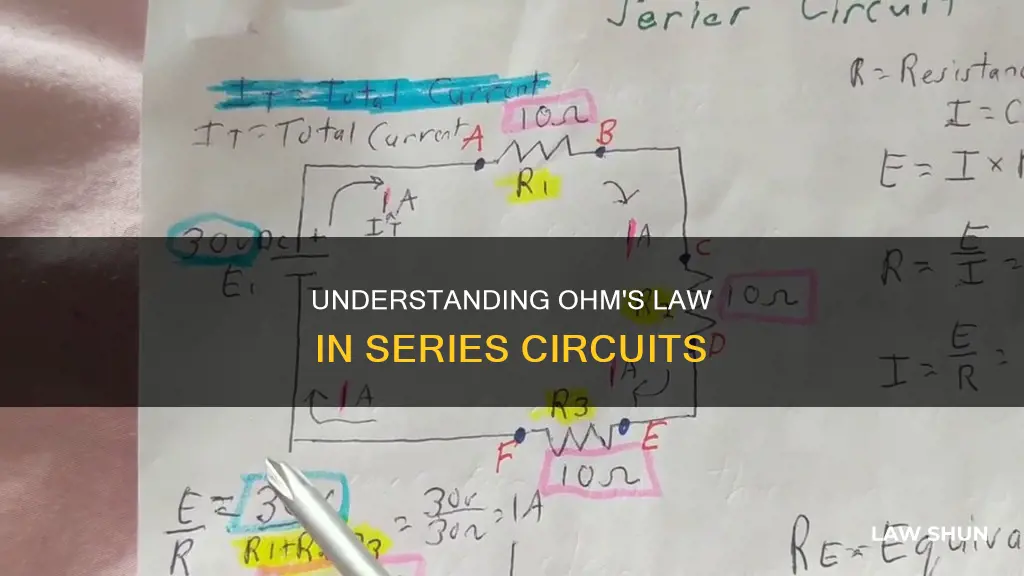
Ohm's Law states that the voltage across a conductor is directly proportional to the current flowing through it, provided that the temperature of the conductor remains constant. In other words, given any two of voltage, current, and resistance, the third can be calculated.
Ohm's Law can be applied to series circuits, but there are some important rules to keep in mind. In a series circuit, the current is the same through any component in the circuit because there is only one path for the current to flow. The total resistance in a series circuit is equal to the sum of the individual resistors, and the total voltage drop is equal to the sum of the individual voltage drops across those resistors.
When applying Ohm's Law to a series circuit, it is important to ensure that all quantities (voltage, current, resistance, and power) relate to each other in terms of the same two points in the circuit. This is a common mistake made by beginners.
| Characteristics | Values |
|---|---|
| Ohm's Law | The relationship between voltage, resistance and current: given any two, you can calculate the third. |
| Application to Series Circuits | Ohm's Law can be applied to series circuits, but only when considering constant-value resistive elements in a lumped-element circuit model. |
| Application to AC Circuits | Ohm's Law does not always apply to AC circuits. |
| Application to Other Materials | Ohm's Law does not apply to insulators, capacitors, inductors, switches, transistors, vacuum, voltage sources, current sources, dielectrics, semiconductors, and many others. |
What You'll Learn

Ohm's Law in a Simple, Single Resistor Circuit
Ohm's Law states that the current flowing through most substances is directly proportional to the voltage applied to it. This relationship was first demonstrated by German physicist Georg Simon Ohm in the 1800s.
The formula for Ohm's Law is:
$$I = \frac{V}{R}$$
Where:
- I = Current (amperes)
- V = Voltage (volts)
- R = Resistance (ohms)
Ohm's Law can be applied to a simple, single-resistor circuit to calculate the current, voltage, or resistance. For example, let's consider a circuit with a voltage of 9 V and a resistance of 3 kΩ. Using Ohm's Law, we can calculate the current as follows:
$$I = \frac{9 \text{ V}}{3 \text{ k}\Omega} = 3 \text{ mA}$$
So, the current in the circuit is 3 mA.
It is important to note that Ohm's Law assumes a linear relationship between voltage and current, and it does not hold for all circuit elements or under all conditions. For example, it does not apply to diodes, transistors, or other non-ohmic materials where the relationship between voltage and current is nonlinear.
In summary, Ohm's Law can be a useful tool for analysing simple, single-resistor circuits, but it has limitations and may not always provide accurate results, especially in more complex circuits or with non-ohmic materials.
Minimum Wage Law: W9 Worker Rights Explained
You may want to see also

Using Ohm's Law in Circuits with Multiple Resistors
Ohm's law can be applied to series circuits, but it is easy to misapply the equations.
When using Ohm's law to calculate a variable of a single component, be sure the voltage, current, and resistance you're referencing are solely across that single component. Likewise, when calculating a variable of a set of components in a circuit, be sure that the voltage, current, and resistance values are specific to that complete set of components only.
In a series circuit, the same amount of current flows through each component in the circuit. This is because there is only one path for the current flow. However, the voltage drop across each resistor in the series will be different.
To calculate the total resistance in a series circuit, simply add up the values of each resistor in the series.
For example, in a series circuit with three resistors of 3 kΩ, 10 kΩ, and 5 kΩ, the total resistance would be 18 kΩ.
Now that we know the total resistance, we can use Ohm's law to calculate the total current.
Using the same example, with a voltage of 9 V and a total resistance of 18 kΩ, the total current would be 500 µA.
We can then use Ohm's law to determine the voltage drop across each resistor.
For the 3 kΩ, 10 kΩ, and 5 kΩ resistors, the voltage drop would be 1.5 V, 5.0 V, and 2.5 V, respectively.
It is important to note that Ohm's law only applies to resistors and a few other resistive components. It does not apply to other materials and devices, such as insulators, capacitors, inductors, switches, transistors, and vacuum.
Skype Surveillance: Are Your Calls and Chats Being Watched?
You may want to see also

Combining Multiple Resistors into an Equivalent Total Resistor
Resistors can be connected in series and parallel to form complex resistive circuits. The method of calculating the circuits' equivalent resistance is the same as that for any individual series or parallel circuit.
In a series circuit, the total resistance is equal to the sum of the individual resistors. For example, if you need 1,100 ohms of resistance and can't find an 1,100 Ω resistor, you can combine a 1,000 Ω resistor and a 100 Ω resistor in series, giving you a total resistance of 1,100 Ω.
In a parallel circuit, the total resistance is calculated by taking the reciprocal of the sum of the reciprocals of the individual resistors. For example, if you have two 1 kΩ resistors in parallel, the total resistance is 500 Ω.
You can also combine resistors in both series and parallel within the same circuit to produce more complex resistive networks. For example, consider the following circuit:
! [Circuit diagram with resistors R1, R2, R3, and R4 connected in series and parallel]('circuit_diagram.png')
Here, resistors R2 and R3 are connected in series, so we can replace them with a single resistor of resistance R2 + R3 = 8 Ω + 4 Ω = 12 Ω. Now we have a single resistor RA in parallel with R4. Using the formula for two parallel resistors, we find the equivalent resistance:
! [Equivalent resistance formula for two parallel resistors]('equivalent_resistance_formula.png')
RA and R1 are now in series, so we can add their resistances to get the total resistance between points A and B: R(AB) = Rcomb + R1 = 6 Ω + 6 Ω = 12 Ω. Thus, we can replace the original four resistors with a single resistor of just 12 Ω.
By using Ohm's Law, we can calculate the current flowing around the circuit:
! [Ohm's Law formula]('ohms_law_formula.png')
We can also calculate the two branch currents, I1 and I2:
! [Equations for calculating branch currents]('branch_currents_equations.png')
Since the resistive values of the two branches are the same (12 Ω), the two branch currents are also equal (500 mA each), giving a total supply current of 1.0 amperes.
We can take this one step further and calculate the voltage drop across each resistor:
! [Equations for calculating voltage drop across each resistor]('voltage_drop_equations.png')
This gives us voltage drops of 1.5 V, 5.0 V, and 2.5 V, respectively. Notice that the sum of these voltage drops (9.0 V) is equal to the battery (supply) voltage of 9 V.
In summary, when combining multiple resistors in series, simply add their resistances to find the total resistance. In parallel, take the reciprocal of the sum of the reciprocals of the individual resistances. For circuits with both series and parallel connections, replace each combination with its equivalent resistance until you're left with a single equivalent resistance.
HIPAA Laws: What Happens After Death?
You may want to see also

Calculating Circuit Current Using Ohm's Law
Ohm's Law is a fundamental formula in electrical and electronics engineering. It can be used to calculate the relationship between voltage, current, and resistance in a circuit. The law is expressed as:
Voltage = Current x Resistance
Volts = Amps x Ohms
V = A x Ω
Where:
- V = Voltage (measured in volts)
- I = Current (measured in amps)
- R = Resistance (measured in ohms)
Using Ohm's Law, if two of these values are known, the third can be calculated.
In a series circuit, all components are connected end-to-end, forming a single path for current flow. The total resistance in a series circuit is the sum of the individual resistors, and the total voltage drop equals the sum of the individual voltage drops across those resistors.
To calculate the total current in a series circuit, you can use the formula:
Total Current (I_total) = Total Voltage (V_total) / Total Resistance (R_total)
Here's an example to illustrate this:
Let's say you have a series circuit with a 9-volt battery and three resistors with resistances of 3 kΩ, 10 kΩ, and 5 kΩ, respectively. To find the total current, follow these steps:
Calculate the total resistance:
- R_total = R1 + R2 + R3
- R_total = 3 kΩ + 10 kΩ + 5 kΩ
- R_total = 18 kΩ
Plug the values into the formula:
- I_total = V_total / R_total
- I_total = 9 V / 18 kΩ
Calculate the total current:
I_total = 500 μA
So, the total current in the circuit is 500 microamperes (μA).
Ohm's Law is a valuable tool for calculating circuit parameters and is especially useful when you need to determine resistance in an operating circuit without shutting it off.
Curfew Laws: Juvenile-Specific or Universal?
You may want to see also

Calculating Component Voltages Using Ohm's Law
Ohm's Law states that the current through a conductor between two points is directly proportional to the voltage. This law is true for circuits that contain only resistive elements, regardless of whether the driving voltage or current is constant (DC) or time-varying (AC).
Ohm's Law can be used to calculate the voltage of components in series circuits. In a series circuit, all components are connected end-to-end to form a single path for current flow. The total voltage drop in a series circuit is equal to the sum of the individual voltage drops across each component.
To calculate the voltage drop across each component, you can use the equation:
V = I x R
Where:
- V is the voltage in volts
- I is the current in amperes
- R is the resistance in ohms
For example, let's say you have a series circuit with a 9-volt battery connected to three resistors with resistances of 3 kΩ, 10 kΩ, and 5 kΩ, respectively. To find the voltage drop across each resistor, you can use Ohm's Law:
For the first resistor (R1):
V_R1 = I_R1 x R1
Where I_R1 is the current through R1, which is the total current supplied by the battery divided by the total resistance of the circuit.
So, if the total current supplied by the battery is 500 µA (calculated using the equation I = V/R, where V is the voltage of the battery and R is the total resistance of the circuit), then:
V_R1 = 500 µA x 3 kΩ = 1.5 V
You can calculate the voltage drop across the other two resistors in the same way.
It's important to note that Ohm's Law assumes a linear relationship between voltage and current, and it may not always hold true for certain components or circuit configurations.
Left Lane Laws: City Street Exception?
You may want to see also
Frequently asked questions
Ohm's Law states that the voltage can be found by multiplying the current and resistance in a circuit or component.
The key principles of a series circuit are:
- The amount of current is the same through any component in a series circuit.
- The total resistance of a series circuit is equal to the sum of the individual resistances.
- The supply voltage in a series circuit is equal to the sum of the individual voltage drops.
When applying Ohm's Law to a series circuit, it is important to remember that all quantities (voltage, current, resistance and power) must relate to each other in terms of the same two points in a circuit.
Kirchhoff's Voltage Law states that the algebraic sum of all voltages in a loop must equal zero. This applies to series circuits as the individual voltage drops add up to the total applied voltage.
The total resistance in a series circuit is equal to the sum of the individual resistances.