Fick's Law, first posited by Adolf Fick in 1855, describes the movement of molecules from a region of higher concentration to a region of lower concentration. It is a law of diffusion, and a diffusion process that obeys Fick's laws is called normal or Fickian diffusion. Fick's first law can be used to derive Fick's second law, which is identical to the diffusion equation.
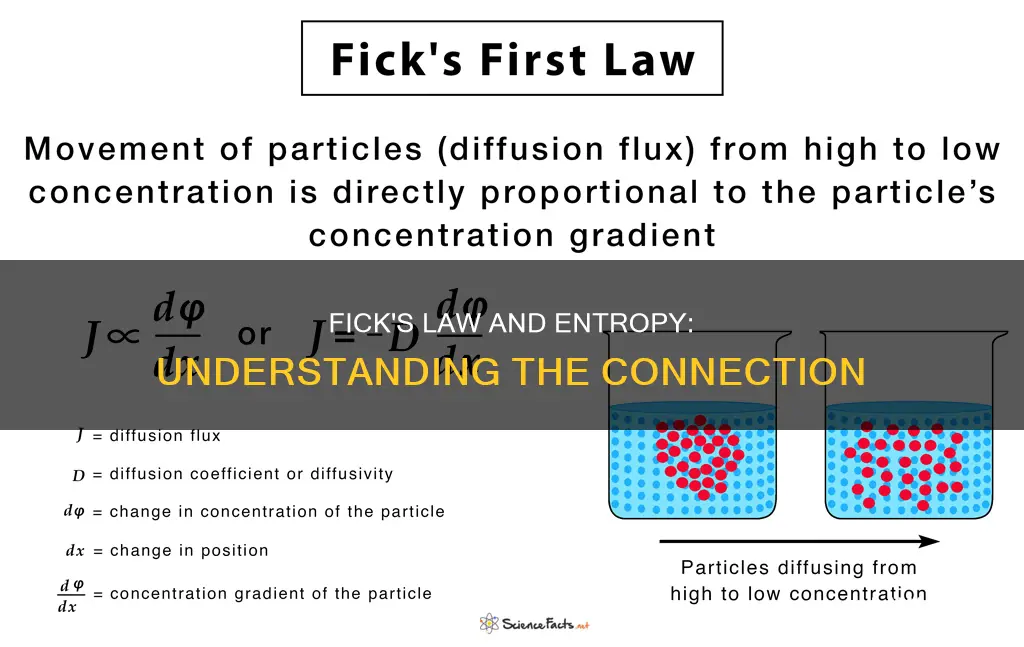
Fick's first law states that the movement of particles from high to low concentration (diffusive flux) is directly proportional to the particle's concentration gradient. Fick's second law predicts how the concentration of particles changes over time due to diffusion.
Fick's law is analogous to other relationships discovered in the same period, such as Darcy's law (hydraulic flow), Ohm's law (charge transport), and Fourier's law (heat transport).
Fick's law is particularly relevant to the concept of entropy, which is a measure of the disorder of a system. Entropy also describes how much energy within a system is not available to do work. Fick's law describes the movement of particles from a higher to a lower concentration, which can be thought of as a movement from a less disordered state to a more disordered state, or a state of higher entropy. This is because, in a system with a concentration gradient, particles will naturally move from areas of higher concentration to areas of lower concentration until the gradient is eliminated, resulting in a more disordered system.
| Characteristics | Values |
|---|---|
| Fick's Law | Describes the movement of molecules from a higher concentration to a lower concentration region |
| Was first posited by Adolf Fick in 1855 | |
| Can be used to solve for the diffusion coefficient, D | |
| Fick's First Law | Can be used to derive Fick's Second Law |
| Relates the diffusive flux to the gradient of the concentration | |
| States that the flux goes from regions of high concentration to regions of low concentration, with a magnitude that is proportional to the concentration gradient | |
| Fick's Second Law | Predicts how diffusion causes the concentration to change with respect to time |
| Is a partial differential equation | |
| Is identical to the diffusion equation | |
| Fickian Diffusion | A diffusion process that obeys Fick's Laws |
| Non-Fickian Diffusion | A diffusion process that does not obey Fick's Laws |
What You'll Learn

Fick's law and the second law of thermodynamics
Fick's law can be broken down into two parts: Fick's first law and Fick's second law. Fick's first law states that the movement of particles is directly proportional to the concentration gradient, meaning that particles will move from a region of higher concentration to a region of lower concentration. This law can be expressed mathematically as:
> J = -D * (∂ϕ/∂x)
Where:
- J is the diffusion flux ( [amount of substance] per unit area per unit time)
- D is the diffusion coefficient ( [length^2] per unit time)
- Φ is the concentration ( [amount of substance] per unit volume)
Fick's second law describes how the concentration changes over time due to diffusion. It states that the rate of change of concentration concerning time is proportional to the second derivative of concentration with respect to position, with the diffusion coefficient (D) as the proportionality constant. The mathematical representation of Fick's second law is:
> ∂ϕ/∂t = D * ∂^2ϕ/∂x^2
Where:
- ∂ϕ/∂t represents the rate of change of concentration with time
- ∂^2ϕ/∂x^2 is the second spatial derivative of concentration, indicating how the concentration profile changes with space
The second law of thermodynamics, on the other hand, establishes the concept of entropy as a physical property of a thermodynamic system. It predicts whether processes are forbidden despite obeying the requirement of conservation of energy as stated in the first law of thermodynamics. The second law asserts that natural processes have a specific direction and are not reversible. In simpler terms, it tells us that heat always flows spontaneously from hotter to colder regions of matter.
Fick's law has found applications in various fields, including biological systems, pharmaceutical sciences, chemical engineering, and food science. It is particularly useful for modelling drug release and diffusion processes in drug delivery systems, as well as understanding gas exchange in respiratory membranes. Additionally, Fick's law plays a crucial role in semiconductor fabrication, where it is used to control and predict the diffusion of chemicals or dopants during the manufacturing process.
In summary, Fick's law and the second law of thermodynamics are fundamental principles that govern the behaviour of particles and energy in various systems. Fick's law describes the rate and direction of diffusion, while the second law of thermodynamics establishes the concept of entropy and provides insights into the directionality and spontaneity of processes. Together, these laws provide a deeper understanding of the complex behaviour of matter and energy in the universe.
Stark Laws and Nurse Practitioners: Understanding the Legal Boundaries
You may want to see also

Diffusion and entropy
Fick's law, established by Adolf Fick in 1855, describes the diffusion process, which is the movement of molecules from a region of higher concentration to a region of lower concentration. This movement is governed by the concentration gradient, with the rate of diffusion being directly proportional to the gradient. In other words, Fick's law states that there is a net flow of particles from areas of higher concentration to areas of lower concentration, with the magnitude of this flow depending on the steepness of the concentration gradient.
Now, let's delve into how Fick's law applies to entropy:
Entropy and Disorder
Entropy is a measure of the disorder in a system. When molecules diffuse, they tend to spread out and mix, increasing the disorder in the system. This is because the molecules are moving from a region where they are more concentrated (higher concentration) to a region where they are less concentrated (lower concentration). This dispersal of molecules is a form of disorder, and it contributes to an increase in entropy. In the context of Fick's law, the concentration gradient drives the increase in entropy by facilitating the movement of molecules from high to low concentration regions.
Second Law of Thermodynamics
The second law of thermodynamics states that in any spontaneous process, the total entropy of a closed system either increases or remains constant; it never decreases. This law is closely related to Fick's law and diffusion. When heat is transferred from a hotter object to a cooler one, the entropy of the system increases. This is because the heat energy disperses from a concentrated region (the hot object) to a less concentrated region (the cooler object), mirroring the movement of molecules in diffusion.
Non-Equilibrium Thermodynamics
Fick's law also has implications for non-equilibrium thermodynamics. In systems far from equilibrium, diffusion plays a crucial role in helping the system reach a state of maximum entropy. This increase in entropy occurs in a near-equilibrium manner, where the flux of particles is linearly proportional to the driving force causing the flux. In this context, the driving force can be thought of as the chemical potential gradient, which is the difference in chemical potential between two regions. The chemical potential is related to the concentration of particles, so a concentration gradient can drive the flux of particles, increasing entropy in the process.
Applications
Fick's law and its connection to entropy have practical applications in various fields. For example, in semiconductor fabrication, Fick's law is used to predict and control the diffusion of dopants or chemicals during the manufacturing process. In biology, Fick's law helps explain the movement of molecules across cell membranes and the diffusion of nutrients and gases in tissues. In pharmaceutical and food industries, Fick's law is applied to understand the release of drugs from delivery systems and the diffusion of flavours and colours in food products.
The Law of Expansion: Seawater's Response
You may want to see also

Fick's law and entropy in semiconductor fabrication
Fick's laws of diffusion, first posited by Adolf Fick in 1855, describe the movement of particles from high to low concentration (diffusive flux) and are directly proportional to the particle's concentration gradient. Fick's first law can be used to derive his second law, which is identical to the diffusion equation. Fick's laws form the core of our understanding of diffusion in solids, liquids, and gases.
Fick's law can be applied to semiconductor fabrication to control and predict the diffusion of chemicals or dopants from one layer to another. By knowing the concentration of dopants or chemicals moving per meter and second through mathematics, different types and levels of semiconductors can be fabricated. Integrated circuit fabrication technologies, such as CVD, thermal oxidation, wet oxidation, and doping, use diffusion equations obtained from Fick's law.
For example, in the CVD method of semiconductor fabrication, a wafer is coated with a layer of CVD-created polymer chains and films, which contain n-type and p-type dopants. The CVD process relies on gas-phase and gas-solid chemical reactions to create thin films. The velocity profile for gas flow is given by:
> δ(x) = (5x/Re^0.5)Re = vρL/η
Where:
- Δ is the thickness
- Re is the Reynolds number
- X is the length of the substrate
- V = 0 at any surface
- Η is viscosity
- Ρ is density
Fick's first law describes diffusion through the boundary layer and can be used to predict the flux of reactants to the substrate and products away from the substrate:
> J = -Di(dci/dx)
Where:
- X is the thickness
- Δ is the thickness
- Dci is the first reactant's concentration
Fick's law tells us that we can use a partial pressure gradient to control the diffusivity and growth of thin films of semiconductors. However, in many realistic situations, Fick's law is not adequate for the semiconductor problem and only applies to certain conditions. For instance, it can be applied to constant source concentration diffusion, limited source concentration, or moving boundary diffusion.
Walther's Law: Examining Applicability and Exceptions to the Rule
You may want to see also

Fick's law and entropy in food production
Fick's laws of diffusion, first posited by Adolf Fick in 1855, describe the movement of particles from areas of high concentration to low concentration. This is directly proportional to the particle's concentration gradient. Fick's laws are used to solve for the diffusion coefficient, D.
Fick's laws are used in a variety of applications, including food production and cooking. For example, Fick's laws can be used to predict the movement of salt and sugar molecules during meat brining and marinating, or the movement of water molecules during dehydration.
Fick's first law can also be used to predict the changing moisture profiles across a spaghetti noodle as it hydrates during cooking. This is an example of how Fick's law can be used to control the cooking time and shape of the food.
Fick's law is also important in the context of semiconductor fabrication. It can be used to control and predict the movement of chemicals or dopants in semiconductor materials, allowing for the fabrication of different types and levels of semiconductors.
In summary, Fick's laws of diffusion provide a framework for understanding and predicting the movement of particles, with applications in a variety of fields including food production, cooking, and semiconductor manufacturing.
Lemon Law and Auction Cars: What's the Deal?
You may want to see also

Fick's law and entropy in biological systems
Fick's laws of diffusion describe the movement of particles from high to low concentration, and they were first posited by Adolf Fick in 1855. Fick's laws are widely applied in biological systems, including:
- Biological molecules: Fick's law can be used to calculate the diffusion coefficients of biological molecules, which typically range from 10−10 to 10−11 m2/s.
- Neurons: Fick's law can be used to model transport processes in neurons.
- Biopolymers: Fick's law can be applied to understand transport processes in biopolymers.
- Blood circulation: Fick's law can be used to estimate the exchange rate of gases across fluid membranes in the circulatory system.
- Brining and marinating meat: The diffusion of salt and sugar molecules into meat can be described by Fick's law.
- Cooking pasta: Fick's law can be used to predict the changing moisture profiles across a spaghetti noodle as it hydrates during cooking.
EMT Wage Laws: Free Housing Impact
You may want to see also
Frequently asked questions
Fick's Law, first posited by Adolf Fick in 1855, describes the movement of molecules from a region of higher concentration to a region of lower concentration. It is used to solve for the diffusion coefficient, D. Fick's first law can be used to derive the second law, which is identical to the diffusion equation.
Fick's Law is related to entropy as it describes the movement of molecules from a region of higher concentration to a region of lower concentration, which is a fundamental concept in understanding entropy. Entropy is a measure of the disorder of a system, and it describes how much energy within a system is not available to do work. The more disordered a system is and the higher its entropy, the less of its energy can be used to do work. Fick's Law helps us understand how molecules disperse and spread out from areas of higher concentration to lower concentration, contributing to the overall increase in entropy of a system.
Fick's Law can be applied to various scenarios involving entropy. For instance, when a hot object is placed in a room, it spreads heat energy in all directions, increasing the entropy of the room. Similarly, when water evaporates from a dish, the water molecules disperse into the surrounding air, leading to an increase in entropy. In these examples, Fick's Law describes the movement of molecules from areas of higher concentration (hot object, water in a dish) to areas of lower concentration (room, surrounding air), aligning with the fundamental principles of entropy.