Gauss's Law, a fundamental principle in electromagnetism, describes the relationship between the distribution of electric charges and the resulting electric field. This law, formulated by Joseph-Louis Lagrange and later by Carl Friedrich Gauss, states that the total electric flux through a closed surface is directly proportional to the net electric charge enclosed by that surface. In other words, it helps us understand how electric charges create electric fields.
Gauss's Law has various real-life applications, particularly in solving complex electrostatic problems with unique symmetries such as cylindrical, spherical, or planar symmetry. Here are some examples of how Gauss's Law is applied in everyday life:
- Understanding Fields Around Conductors: Gauss's Law is used to predict and analyse the behaviour of electric fields around conductors like wires and Faraday cages. This knowledge is crucial for the safe and efficient design of electrical circuits and devices.
- Medical Imaging Technology: While not the primary principle, Gauss's Law aids in understanding the electric fields used in MRI and X-ray machines, contributing to the accuracy and effectiveness of medical imaging techniques.
- Particle Accelerator Design: Electric fields play a crucial role in particle accelerators and radiation therapy. Gauss's Law is used to design electrodes that create these electric fields, allowing for more precise and effective particle acceleration.
- Electronic Circuit Design: Many electronic devices, such as transformers, capacitors, and televisions, rely on the manipulation of electric fields, which is guided by the underlying principles of Gauss's Law.
| Characteristics | Values |
|---|---|
| Total electric flux out of a closed surface | Equal to the charge contained inside the surface divided by the absolute permittivity |
| Electric flux in an area | Electric field multiplied by the surface area projected in a plane perpendicular to the field |
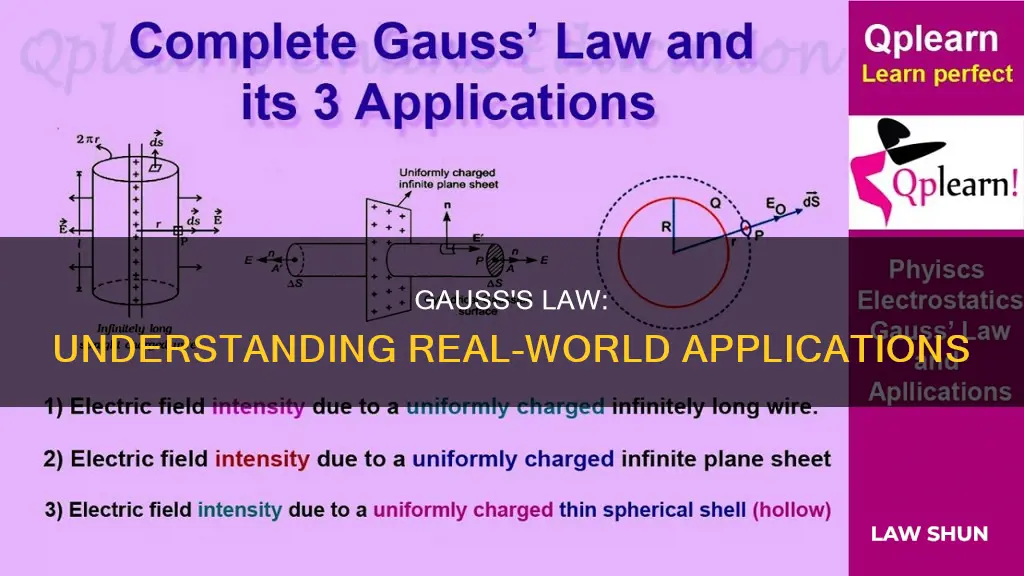
| Electric field due to infinite wire | E = λ/2πrε0 |
| Electric field due to infinite plane sheet | E = σ/2ε0K |
| Electric field due to thin spherical shell | E = σR2/ε0r2 |
| Understanding fields around conductors | Predicting how electric fields will behave around conductors to design electrical circuits and devices |
| Medical imaging technology | Understanding the electric fields used in MRI and X-ray machines |
| Particle accelerator design | Designing electrodes that create electric fields to make particle acceleration more precise and effective |
What You'll Learn
- Understanding Fields Around Conductors: Gauss's Law helps predict electric fields around conductors like wires and Faraday cages, aiding the design of electrical circuits and devices
- Medical Imaging Technology: While not central, Gauss's Law helps understand electric fields used in MRI and X-ray machines, contributing to the accuracy of medical imaging methods
- Particle Accelerator Design: Gauss's Law is used to design electrodes that create electric fields for particle acceleration in accelerators and medical treatments like radiation therapy
- Electric Fields of Charged Rods: Students use charged rods and small balls on strings to observe how ball movement changes with distance, relating these observations to Gauss's Law
- Electric Fields Inside Conductors: Using charged electroscopes and hollow metal spheres, students confirm that electric fields inside conductors are zero, aligning with Gauss's Law principles

Understanding Fields Around Conductors: Gauss's Law helps predict electric fields around conductors like wires and Faraday cages, aiding the design of electrical circuits and devices
Gauss's Law, also known as Gauss's flux theorem, is a fundamental principle in physics that helps us understand how electric charges create electric fields. It states that the total number of electric field lines passing through a closed surface is directly related to the total charge enclosed by that surface. In other words, the net electric flux through a closed surface is equal to the total electric charge enclosed by that surface divided by the electric permittivity of the medium.
Gauss's Law is particularly useful for understanding electric fields around conductors, such as wires and Faraday cages. These are metal enclosures that block electric fields, and engineers use Gauss's Law to predict how electric fields will behave around them. This knowledge aids in the design of electrical circuits and devices, ensuring they work safely and efficiently.
For example, Gauss's Law can explain why your phone loses signal in an elevator. It calculates the nearly zero electric field inside the metal enclosure of the elevator. This understanding of fields around conductors is essential for designing electrical devices that function optimally in various environments.
Additionally, Gauss's Law helps in creating safer electrical devices and enhancing medical imaging techniques, such as MRI and X-ray machines. It provides insights into the electric fields used in these technologies, allowing scientists to improve their accuracy and usefulness.
Romeo and Juliet Law: Texas' Take on Young Love
You may want to see also

Medical Imaging Technology: While not central, Gauss's Law helps understand electric fields used in MRI and X-ray machines, contributing to the accuracy of medical imaging methods
Gauss's Law, also known as Gauss's Flux Theorem, is a foundational principle in electromagnetism. It states that the total electric flux passing through a closed surface is directly proportional to the electric charge contained within that surface. In other words, the law describes the relationship between the distribution of electric charges and the resulting electric field.
While Gauss's Law is not central to the functioning of MRI and X-ray machines, it does help us understand the electric fields used in these technologies, thus contributing to the accuracy of medical imaging methods.
MRI (Magnetic Resonance Imaging) machines use strong magnetic fields and radio waves to generate images of the inside of a person's body. The basic principle behind MRI is that when a person is placed in a strong magnetic field, the nuclei of certain atoms in their body (such as hydrogen atoms) align with the direction of the field. When radio waves are then pulsed through the person's body, these aligned nuclei absorb this energy and release it once the radio waves are turned off. This released energy is detected by the MRI machine and used to create detailed images of the body's internal structures.
Gauss's Law helps us understand the behaviour of electric fields in MRI machines, particularly in relation to the distribution of electric charges. The law can be applied to analyse the electric fields generated by the MRI machine's magnetic field, which can influence the alignment of atomic nuclei and, consequently, the quality of the resulting images.
X-ray machines, on the other hand, use a small amount of radiation to create images of the inside of the body. When X-rays pass through the body, they are absorbed or weakened to varying degrees by different types of tissues. For example, dense materials like bones will block most of the X-rays, while softer tissues will allow more X-rays to pass through. An X-ray detector on the other side of the body captures the X-rays that pass through and translates this information into images.
Similar to its application in MRI machines, Gauss's Law can be used to understand the electric fields involved in X-ray machines and how they interact with the electric charges in different types of tissues. This understanding can help optimise the use of electric fields in X-ray technology, improving image accuracy and patient safety.
Jim Crow Laws: Northern Exposure?
You may want to see also

Particle Accelerator Design: Gauss's Law is used to design electrodes that create electric fields for particle acceleration in accelerators and medical treatments like radiation therapy
Gauss's Law is a fundamental principle in electromagnetism that relates the distribution of electric charge to the resulting electric field. It states that the total electric flux through a closed surface is directly proportional to the total charge enclosed by that surface. This law helps us understand how electric charges create electric fields and is particularly useful in cases with symmetrical charge distributions.
Particle Accelerator Design
Particle accelerators are machines that use electromagnetic fields to accelerate charged particles to very high speeds and energies. They have a wide range of applications, from fundamental research in particle physics to industrial processes and medical treatments. Gauss's Law is used in the design of electrodes that create electric fields for particle acceleration in accelerators and medical treatments like radiation therapy.
Here's how Gauss's Law is applied in particle accelerator design:
- Understanding Electric Fields: Gauss's Law helps engineers understand and manipulate electric fields. It states that the total number of electric field lines passing through a closed surface is directly related to the total charge enclosed by that surface.
- Electrode Design: By using Gauss's Law, engineers can design electrodes that create electric fields. The shape and arrangement of the electrodes are crucial for effective particle acceleration.
- Particle Acceleration: Electric fields generated by the electrodes accelerate charged particles to high speeds. The particles are steered and focused using magnetic fields.
- Precision and Effectiveness: By applying Gauss's Law to shape the electrodes, scientists can make particle acceleration more precise and effective. This is especially important in medical treatments like radiation therapy, where precise control of the particle beam is essential.
- Symmetry Considerations: Gauss's Law is particularly useful in cases with symmetrical charge distributions. In particle accelerators, cylindrical symmetry is often present, and Gauss's Law can be applied accordingly.
- Medical Applications: Particle accelerators are used in medical treatments, such as radiation therapy, to treat cancerous tissue. The precise control of particle acceleration is crucial in these applications.
FAA Laws: International Flights and Their Legal Complexities
You may want to see also

Electric Fields of Charged Rods: Students use charged rods and small balls on strings to observe how ball movement changes with distance, relating these observations to Gauss's Law
Gauss's law is a fundamental principle in physics that describes the relationship between electric charges and the resulting electric field. It states that the total electric flux through a closed surface is directly proportional to the charge enclosed by that surface. In other words, it quantifies the concept of electric flux, which is the number of electric field lines passing through a given surface. This law is particularly useful for calculating electric fields in scenarios with certain symmetries, such as cylindrical, planar, or spherical symmetry.
Now, let's delve into the experiment: "Electric Fields of Charged Rods." In this experiment, students explore the electric fields generated by charged rods and observe how these fields affect the motion of small balls suspended on strings. Here's how it works:
Materials:
- Charged rods: These are typically long, thin rods made of conductive materials like metal. The rods can be charged by rubbing them with appropriate materials (e.g., rubber or silk) or by induction.
- Small balls: Lightweight, non-conductive balls, such as Styrofoam or plastic balls, are used.
- Strings: The strings should be non-conductive and thin enough to minimize their impact on the electric field.
Procedure:
Set up the experiment:
- Place a charged rod on a table or stable surface.
- Suspend a small ball from a string and position it near the charged rod, ensuring it is electrically neutral.
Observe the motion of the ball:
Bring the ball close to the charged rod and observe its movement. Does it move towards or away from the rod? Does the distance between the ball and the rod affect its motion?
Relate observations to Gauss's Law:
Students can calculate the electric field produced by the charged rod using Gauss's Law. They can choose an appropriate Gaussian surface (e.g., a cylinder or a sphere) enclosing the charged rod and apply Gauss's Law to relate the electric flux through that surface to the charge on the rod.
Vary the parameters:
- Experiment with different charges on the rod and observe how it affects the motion of the ball.
- Try using rods of different lengths or materials and note any changes in the ball's movement.
- Adjust the distance between the ball and the rod to see how the electric field strength varies with distance.
Observations and Results:
- Students will observe that the small balls are attracted or repelled by the charged rods, depending on the type of charge. Like charges (positive or negative) will repel each other, while opposite charges will attract.
- By varying the distance between the ball and the rod, students will notice that the electric field strength decreases as the distance increases. This observation aligns with Gauss's Law, which predicts that the electric flux through a closed surface is proportional to the charge enclosed.
- The experiment may also explore the electric field along the axis of the rod and perpendicular to it, observing how the field varies with distance in these different directions.
Explanation:
The experiment "Electric Fields of Charged Rods" helps students visualize and understand the electric fields generated by charged objects and how these fields influence the motion of nearby charged particles. By relating their observations to Gauss's Law, students can calculate the electric field produced by the charged rod and predict how changes in the charge or geometry of the rod affect the resulting electric field. This experiment highlights the practical application of Gauss's Law in understanding electrostatic phenomena.
Who Rules the Ruler? British Law and the Queen
You may want to see also

Electric Fields Inside Conductors: Using charged electroscopes and hollow metal spheres, students confirm that electric fields inside conductors are zero, aligning with Gauss's Law principles
Gauss's Law, also known as Gauss's flux theorem, is a fundamental principle in physics that describes the relationship between electric charge and the resulting electric field. One of the key applications of Gauss's Law is in understanding electric fields inside conductors.
In an experimental setup, students can use charged electroscopes and hollow metal spheres to confirm that electric fields inside conductors are zero, aligning with the principles of Gauss's Law. Here's a step-by-step guide for the experiment:
Materials:
- Charged electroscope
- Hollow metal sphere
- Connecting wires
- Voltage source
Procedure:
- Set up the experiment by connecting the hollow metal sphere to the voltage source using wires. Ensure the sphere is insulated from its surroundings.
- Place the charged electroscope inside the hollow metal sphere, ensuring it is not touching the sphere's inner surface.
- Turn on the voltage source to apply a charge to the hollow metal sphere.
- Observe the behaviour of the electroscope.
Results and Explanation:
During the experiment, students will notice that the electroscope remains unchanged, indicating that there is no electric field inside the hollow metal sphere. This observation confirms that electric fields inside conductors are zero.
According to Gauss's Law, the net electric flux through any closed surface is directly proportional to the charge enclosed by that surface. In the context of this experiment, the hollow metal sphere acts as the closed surface. Since there is no charge enclosed within the sphere (as the charge resides on the outer surface), the net electric flux is zero, resulting in no electric field inside the sphere.
This experiment demonstrates the fundamental principle that electric fields inside conductors are zero, which is a direct consequence of Gauss's Law. By confirming this principle, students gain a deeper understanding of the behaviour of electric fields in conductive materials.
Understanding Romeo and Juliet Laws: Applicability for 21-Year-Olds
You may want to see also
Frequently asked questions
Gauss's Law states that the total electric flux out of a closed surface is equal to the charge enclosed by that surface divided by the permittivity. In simpler terms, it relates the number of electric field lines passing through a closed surface to the total charge enclosed by that surface. This law helps us understand how electric charges create electric fields.
Gauss's Law is used in the design of electronic circuits and devices, helping engineers predict how electric fields will behave around conductors. It also plays an indirect role in the operation of transformers, capacitors, and televisions, as the underlying principles of these devices align with the concepts outlined in Gauss's Law.
Gauss's Law helps scientists understand the electric fields used in MRI and X-ray machines, which create images by applying electric fields to interact with atoms in the body. A better understanding of these fields contributes to the development of more accurate and useful medical imaging methods.
Electric fields are used to push charged particles in particle accelerators and radiation therapy equipment. Gauss's Law is used to design electrodes that create these fields, allowing scientists to make particle acceleration more precise and effective.







