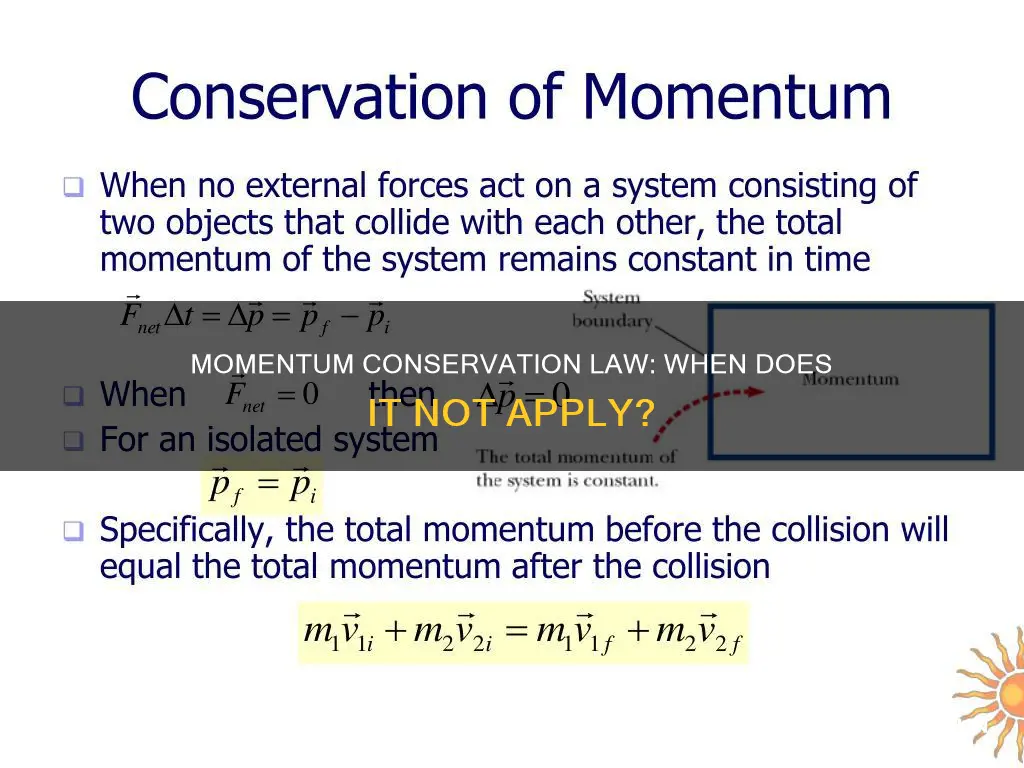
The law of conservation of momentum is a fundamental principle in physics, stating that the total momentum of a system remains constant if no external forces are acting upon it. However, this law has its limitations and does not apply universally. For instance, in the presence of friction or other external forces, the conservation of momentum is not applicable. This is because friction introduces an external force acting on the system, causing a loss of momentum over time. Similarly, in cases where potential energy cannot be defined, such as with the force of friction, the law of conservation of momentum does not hold.
| Characteristics | Values |
|---|---|
| When there is an external force acting on a system | The law of conservation of momentum does not apply |
| When there is friction between moving bodies and their surroundings | The law of conservation of momentum does not apply |

Friction
The law of conservation of momentum states that in a system with no net external forces acting on it, the total momentum of the system remains constant. In other words, if there are no outside forces at play, the total momentum before and after an event will be the same.
However, friction introduces an external force that can disrupt the conservation of momentum. Friction causes a continuous loss of energy in moving objects, resulting in a decrease in velocity over time. This loss of energy and change in velocity violate the principle of conservation of momentum, which requires the absence of external forces.
For example, consider two objects, A and B, on a frictional surface. If object A is moving with a constant velocity and collides with stationary object B, the momentum of the individual objects is not conserved due to the presence of friction. The friction between the objects and the surface results in a loss of energy, leading to a change in velocity.
However, it's important to note that while the momentum of individual objects may not be conserved in the presence of friction, the momentum of the entire system, including the surface or any other objects involved, remains conserved. In the given example, the momentum lost by objects A and B is gained by the surface they are sliding along or even the entire Earth. Since the Earth has a significantly larger mass, the change in its velocity is negligible and often unmeasurable.
In summary, while friction does influence the conservation of momentum by introducing an external force, the overall momentum of the entire system, including all objects and surfaces involved, remains conserved.
Village Laws in Townships: What's the Verdict?
You may want to see also

Non-elastic collisions
The law of conservation of momentum states that in an isolated system, the total momentum of two or more bodies acting upon each other remains constant unless an external force is applied. This means that momentum can neither be created nor destroyed.
Momentum is conserved in any collision, whether elastic or non-elastic. However, kinetic energy is not conserved in non-elastic collisions. In such cases, the kinetic energy is converted into heat energy or potential energy.
A non-elastic collision is one in which the objects stick together after impact and do not separate. In this type of collision, the maximum amount of kinetic energy is lost. The forces between the colliding objects may convert kinetic energy into other forms of energy, such as potential energy or thermal energy.
For example, consider two objects with equal masses heading towards each other at equal speeds. After colliding, they stick together and come to a stop. While momentum is conserved, kinetic energy is not. Some of the energy of motion gets converted to thermal energy, or heat.
Lemon Law: Private Sales in Florida Explained
You may want to see also

External forces
The law of conservation of momentum is a fundamental principle of physics, stating that momentum is neither created nor destroyed within a system, but only altered by the forces described in Newton's laws of motion. This law applies to all three physical directions simultaneously, even when dealing with gases, where forces in one direction can impact momentum in another due to the collisions of molecules.
However, this law only holds true when there are no external forces acting on the system. When external forces are present, the law of conservation of momentum does not apply. External forces can include friction, gravity, applied forces, and air resistance, all of which can affect the total momentum of a system.
Friction, for example, acts as an external force that opposes the motion of an object. When friction is present between moving bodies and their surroundings, conservation of momentum is not applicable as there is a net external force in play. Similarly, air resistance is an external force that acts against the motion of an object moving through a fluid, such as air or water. When an object experiences air resistance, it is subjected to a force that can alter its momentum, thus violating the law of conservation of momentum.
In addition, applied forces, such as a person pushing a box or a car accelerating, are external forces that can change the momentum of an object or system. When these forces are introduced, the total momentum of the system is no longer conserved.
Furthermore, the force of gravity acts as a constant external force on objects with mass near the Earth or other massive bodies. As a result, the momentum of an object can change due to the force of gravity, making the law of conservation of momentum inapplicable in such scenarios.
To summarise, the law of conservation of momentum relies on the absence of external forces to hold true. When external forces are introduced, they can alter the momentum of a system, causing the total momentum to change and violating the fundamental principle of conservation of momentum.
Understanding Rosa's Law: Its Impact and Provisions
You may want to see also

Gases
Dealing with the conservation of momentum in gases can be challenging because gases consist of many molecules that are constantly colliding with each other and their container. This means that forces in one direction can impact the momentum in another direction.
For example, consider the flow of a gas through a pipe, where the only change is in one direction, which we'll call "x". The gas enters the pipe at station 1 with a certain velocity and pressure and exits at station 2 with a different velocity and pressure. In this scenario, the conservation of momentum equation would look something like this:
> F = - [(p * A)2 - (p * A)1] = m * [(u2 - u1) / del t]
Where F is the force, p is the pressure, A is the area, u is the velocity, m is the mass, and del t is the change in time.
This type of problem can be even more complex in the real world, where properties can change in multiple directions and vary over time.
Logarithmic Laws: Natural Logarithm Inclusivity
You may want to see also

Real-world flow problems
The conservation of momentum is a fundamental principle of physics, stating that the amount of momentum within a system remains constant unless acted upon by an external force. It is described by Newton's laws of motion, which state that momentum can only be changed through the action of external forces and that the total momentum within an isolated system remains the same.
However, this principle becomes more complex when dealing with gases and fluids, where real-world flow problems can affect the conservation of momentum. In such cases, the conservation of momentum is influenced by various factors, including the behaviour of gases and fluids, the presence of external forces, and the specific conditions of the flow problem.
For example, gases and fluids exhibit complex behaviour due to the collisions of their molecules. When dealing with a gas or fluid flow, forces in one direction can impact the momentum in another direction due to the random motion and interactions of the particles involved. This makes it challenging to predict and control momentum conservation in these systems.
Additionally, external forces can disrupt the conservation of momentum. For instance, friction and heat loss can affect momentum, causing a decrease in momentum within a system. In real-world flow problems, these external forces can be even more pronounced due to the presence of surfaces, viscosity, and other factors that introduce additional forces and complexities.
Furthermore, the specific conditions of a flow problem can also impact the conservation of momentum. This includes factors such as the shape and size of the domain through which the gas or fluid is flowing, the velocity and pressure gradients, and the density and area through which the flow occurs. All these variables interact to determine the overall momentum conservation within the system.
In conclusion, while the conservation of momentum is a fundamental principle, it becomes more intricate in real-world flow problems involving gases and fluids. The behaviour of these substances, the presence of external forces, and the specific conditions of the flow problem can all influence the conservation of momentum, requiring careful analysis and consideration to fully understand and predict momentum behaviour in these complex systems.
Boyle's Law: Liquids and Gases Explored
You may want to see also







