Gauss's Law is a powerful tool for determining the electric field in situations with specific symmetries, such as spherical, cylindrical, or planar charge distributions. It relates the electric flux through a closed surface to the total charge enclosed by that surface. By choosing an appropriate Gaussian surface, we can apply Gauss's Law to an infinite sheet of charge to find the electric field at a distance from the sheet. The symmetry of the situation allows us to deduce that the electric field is uniform and perpendicular to the sheet, with the direction depending on the sign of the charge. To apply the law, we need to determine the total charge enclosed by the Gaussian surface and the net electric flux passing through it. By equating these two values, we can solve for the magnitude and direction of the electric field. This approach is particularly useful for infinite sheets of charge, where other methods may be more complex.
| Characteristics | Values |
|---|---|
| Definition of Gauss' Law | The total electric flux out of a closed surface is equal to the charge enclosed divided by the permittivity. |
| Formula | $\oint{\vec.\vecs=\frac{1}{{{\in }_{0}}}q} |
| Electric Flux | The electric field multiplied by the area of the surface projected in a plane and perpendicular to the field. |
| Application | Used to determine the electric field of charge distributions with symmetry. |
| Steps to solve for the electric field | 1. Identify the spatial symmetry of the charge distribution. |
| 2. Choose a correct Gaussian surface with the same symmetry as the charge distribution. | |
| 3. Evaluate the integral \(\Phi_sE\) over the Gaussian surface and then calculate the flux through the surface. | |
| 4. Find the amount of charge enclosed by the Gaussian surface. | |
| 5. Evaluate the electric field of the charge distribution. | |
| Types of symmetry | 1. Cylindrical symmetry |
| 2. Calculations of inappropriate coordinate systems are to be performed along with the correct Gaussian surface for the particular symmetry. | |
| Gaussian Surface | A surface through which the electric flux is calculated. |
What You'll Learn

Choosing a Gaussian surface
When applying Gauss' Law to a sheet of charge, choosing the correct Gaussian surface is crucial. Here are some guidelines and considerations for selecting an appropriate Gaussian surface:
Understanding Gaussian Surfaces
Before delving into the specifics of choosing a Gaussian surface, let's briefly understand what a Gaussian surface is. A Gaussian surface is a closed surface in three-dimensional space used to calculate the flux of a vector field, often the electric field in this context. The choice of the Gaussian surface depends on the symmetry of the charge distribution.
Identifying Symmetry
The first step in choosing a Gaussian surface is to identify the symmetry of the charge distribution. There are three common types of symmetry: spherical symmetry, cylindrical symmetry, and planar symmetry. If the charge distribution is spherically symmetric, a spherical Gaussian surface is chosen. If it has cylindrical symmetry, a cylindrical Gaussian surface is used. For planar symmetry, a planar Gaussian surface, such as a box or a slab, is selected.
Exploiting Symmetry
Once the symmetry of the charge distribution is identified, the next step is to choose a Gaussian surface with the same symmetry. This choice simplifies the calculations. For example, if the charge distribution has spherical symmetry, the electric field at any point on the Gaussian surface will be radially directed, and the magnitude of the electric field will be the same everywhere on the surface.
Examples of Gaussian Surfaces
- Spherical Gaussian Surface: Used for finding the electric field or flux produced by a uniformly distributed spherical shell of charge or any other charge distribution with spherical symmetry. The spherical surface is chosen to be concentric with the charge distribution.
- Cylindrical Gaussian Surface: Used for finding the electric field or flux produced by an infinitely long line of uniform charge, an infinite plane of uniform charge, or an infinitely long cylinder of uniform charge. The axis of the cylinder is chosen to be perpendicular to the sheet of charge.
- Planar Gaussian Surface: Used for charge distributions with planar symmetry, such as a uniform charge spread over a large flat surface. The Gaussian surface is typically chosen as a box or a slab that straddles the plane of charges, with its normal directed along the electric field lines.
Simplifying Calculations
The choice of the Gaussian surface should aim to simplify the calculations. For example, choosing a surface where the electric field is uniform and perpendicular to the surface can make the integration easier.
Problem-Solving Strategy
- Identify the spatial symmetry of the charge distribution.
- Choose a Gaussian surface with the same symmetry and identify its consequences.
- Evaluate the integral of the electric field over the Gaussian surface to calculate the flux.
- Determine the amount of charge enclosed by the Gaussian surface.
- Evaluate the electric field of the charge distribution using the results from steps 3 and 4.
Open Carry Laws: Rifles Included or Excluded?
You may want to see also

Calculating the total charge enclosed
To calculate the total charge enclosed, we must first understand the concept of Gauss's Law. Gauss's Law states that the total electric flux out of a closed surface is equal to the charge enclosed divided by the permittivity. In other words, the total flux linked with a closed surface is directly proportional to the charge enclosed by the closed surface. Gauss's Law can be expressed as:
> Φ = → E.d → A = qnet/ε0
Where Φ is the total flux, E is the electric field, dA is the vector representing an infinitesimal element of the area of the surface, and ε0 is the electric constant.
Now, let's consider an example of a sheet of charge. We want to find the magnitude of the electric field a certain distance away from the sheet. In this case, we can use Gauss's Law to solve the problem. First, we need to determine the field and choose an appropriate Gaussian surface. The field should be uniform and perpendicular to the sheet, going outward if the charge is positive and inward if the charge is negative. For the Gaussian surface, we can choose a cylinder whose axis is perpendicular to the sheet.
Next, we need to answer two questions: What is the total charge enclosed by the surface? And, what is the net electric flux passing through the surface? The total charge enclosed is given by qenc = σA, where σ is the charge per unit length and A is the cross-sectional area of the cylinder. To find the net flux, we consider the ends of the cylinder, as the electric field is parallel to its side. The net flux is equal to the electric field multiplied by the area of one end of the cylinder, since the field is uniform.
Finally, we can apply Gauss's Law to solve for the electric field:
> By Gauss's Law, the net flux = qenc/ε0
Therefore, we have calculated the total charge enclosed by the surface and found the electric field using Gauss's Law.
Meiosis and Mendel's Law: Understanding the Connection
You may want to see also

Calculating the net electric flux
To calculate the net electric flux, you need to follow these steps:
Step 1: Understand the Charge Distribution
Firstly, identify the type of charge distribution you are dealing with. This could be spherical symmetry, cylindrical symmetry, or planar symmetry. For example, an isolated point charge exhibits spherical symmetry, while an infinite line of charge displays cylindrical symmetry.
Step 2: Choose a Gaussian Surface
Select a Gaussian surface that matches the symmetry of the charge distribution. For instance, for a charge distribution with spherical symmetry, you would choose a closed spherical surface centred on the distribution.
Step 3: Calculate the Total Enclosed Charge
Determine the total amount of charge enclosed by the Gaussian surface. This can be done by integrating the charge density function over the volume enclosed by the surface.
Step 4: Compute the Electric Flux
Calculate the electric flux through the chosen Gaussian surface. This involves considering the electric field passing through the surface and its orientation relative to the surface. The electric flux is given by the surface integral of the electric field vector dotted with the differential area vector.
Step 5: Apply Gauss' Law
Utilise Gauss' Law to relate the electric flux to the enclosed charge. Gauss' Law states that the net electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space (ε₀). Mathematically, this is expressed as:
Φ = Q/ε₀
Where:
- Φ is the electric flux through the closed surface
- Q is the total electric charge inside the surface
- Ε₀ is the vacuum permittivity or permittivity of free space
By equating the calculated electric flux to the expression given by Gauss' Law, you can solve for the magnitude of the electric field.
Step 6: Determine the Electric Field
Finally, use the calculated electric flux and the known properties of the Gaussian surface to determine the magnitude and direction of the electric field at a specific point.
Florida Fertilizer Laws: Homeowner Compliance Requirements
You may want to see also

Applying Gauss' Law
Gauss's Law states that the total electric flux out of a closed surface is equal to the charge enclosed, divided by the permittivity. The electric flux in an area is defined as the electric field multiplied by the area of the surface projected in a plane and perpendicular to the field.
To apply Gauss's Law to a sheet of charge, we need to determine the electric field. The steps to do so are as follows:
- Identify the spatial symmetry of the charge distribution: In the case of a sheet of charge, the distribution has planar symmetry.
- Choose a Gaussian surface with the same symmetry: A suitable Gaussian surface for a sheet of charge could be a cylinder whose axis is perpendicular to the sheet.
- Evaluate the integral over the Gaussian surface to calculate the flux: The electric field is parallel to the ends of the cylinder and perpendicular to its sides. Thus, the flux through the ends contributes to the total flux, while the flux through the sides is zero.
- Determine the amount of charge enclosed by the Gaussian surface: This is given by the charge per unit length multiplied by the cross-sectional area of the cylinder.
- Apply Gauss's Law to find the electric field: By Gauss's Law, the net flux is equal to the charge enclosed divided by the permittivity. Solving for the electric field, we can find its magnitude.
The direction of the electric field will depend on the sign of the charge. For a positive charge, the field will be directed outward from the sheet, while for a negative charge, it will be directed inward.
Driving Laws: Private Property Exemptions and Confusions
You may want to see also

Finding the magnitude of the electric field
To find the magnitude of the electric field using Gauss's Law, we can follow these steps:
Step 1: Identify the spatial symmetry of the charge distribution
The first step is to identify the symmetry of the charge distribution. This will help us choose the appropriate Gaussian surface. For example, an isolated point charge has spherical symmetry, while an infinite line of charge has cylindrical symmetry.
Step 2: Choose a Gaussian surface with the same symmetry
Select a Gaussian surface that has the same symmetry as the charge distribution. This will allow us to easily determine the electric field direction relative to the surface normal. For instance, if the charge distribution has spherical symmetry, we would choose a sphere as our Gaussian surface.
Step 3: Evaluate the flux through the Gaussian surface
Calculate the flux of the electric field through the chosen Gaussian surface. The symmetry of the charge distribution and the Gaussian surface should allow us to simplify the calculation. For example, if the electric field is uniform and perpendicular to the surface, the flux calculation becomes straightforward.
Step 4: Determine the amount of charge enclosed
Find the total charge enclosed by the Gaussian surface. This may involve integrating the charge density over the volume, surface, or length enclosed by the surface, depending on the type of charge distribution.
Step 5: Apply Gauss's Law to find the electric field
Finally, we can apply Gauss's Law to relate the flux and the charge enclosed to the electric field. Gauss's Law states that the electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space. By rearranging this equation, we can solve for the magnitude of the electric field.
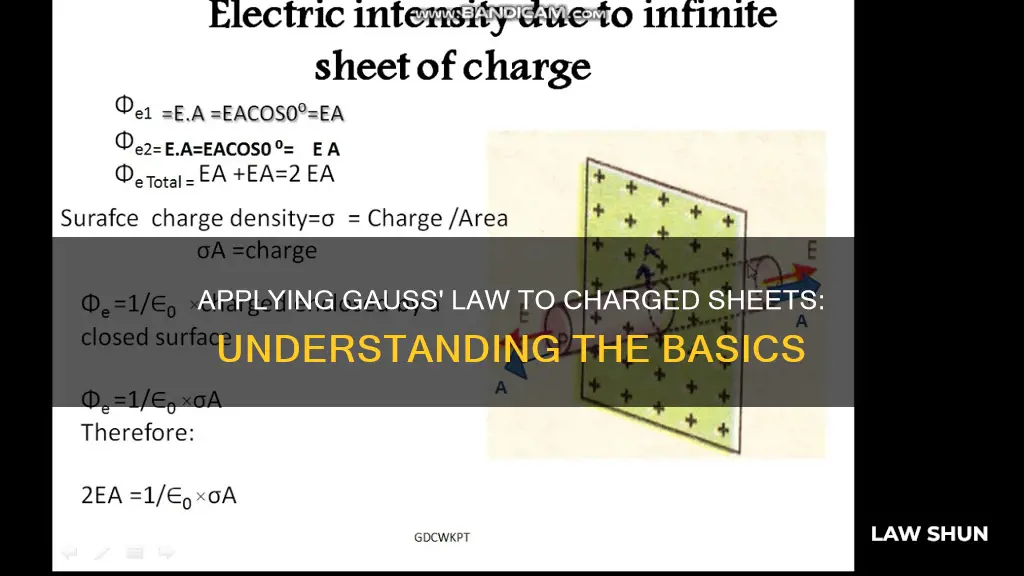
Example: Infinite Sheet of Charge
Let's consider an example of an infinite sheet of charge with uniform charge density σ. We want to find the magnitude of the electric field a distance 'r' from the sheet.
Choosing the Gaussian surface
In this case, we can choose a cylinder whose axis is perpendicular to the sheet. The cylinder has a cross-sectional area 'A' and runs from -a to +a, enclosing the sheet.
Evaluating the flux and charge enclosed
The electric field is uniform and perpendicular to the ends of the cylinder, so the flux through the ends contributes to the total flux. The electric field is parallel to the side of the cylinder, so there is no contribution to the flux from the side surface. Thus, the net flux is given by 2E(a)A, where E(a) is the electric field strength at x=+a.
The charge enclosed by the cylinder is simply the charge density σ multiplied by the cross-sectional area A.
Applying Gauss's Law
According to Gauss's Law, the net flux is equal to the charge enclosed divided by the permittivity of free space ε0. Setting up the equation, we have:
2E(a)A = σA/ε0
Solving for the electric field magnitude, we get:
E = σ/2ε0
So, the magnitude of the electric field due to an infinite sheet of charge is σ/2ε0, and it is directed outward if the charge is positive and inward if the charge is negative.
CISG vs Domestic Law: How Far Does It Reach?
You may want to see also







