Several gas laws apply in space, including Avagadro's law, which helps determine the amount of gas in a given volume, and Boyle's law, which is used to understand the pressure of gas that can be applied without causing damage. These laws are essential for astronauts and space travellers, as they help predict the behaviour of gases and prevent issues such as decompression sickness. The ideal gas law, though not perfect, also provides a good approximation for understanding gas behaviour under various conditions. Charles' Law and Dalton's Law are other gas laws that are relevant in space.
| Characteristics | Values |
|---|---|
| Avagadro's Law | States that at a constant temperature and pressure, the number of atoms/gas are directly proportional to the volume occupied by the gas |
| Boyle's Law | States that at a constant temperature and number of moles, the pressure exerted by the gas is inversely proportional to the volume occupied |
| Charles' Law | V1/V2 = T1/T2; Gas expands when heated if pressure is kept constant |
| Ideal Gas Law | PV = nRT |
What You'll Learn
- Avagadro's Law: At a constant temperature and pressure, the volume occupied by a gas is proportional to the number of atoms
- Boyle's Law: Used to calculate the pressure of gas that can be used without wasting space
- Charles' Law: Similar to Boyle's Law but with a different variable held constant
- Dalton's Law: The total pressure of a gas mixture is the sum of the partial pressures of each gas
- Henry's Law: The solubility of a gas in a liquid is directly proportional to the partial pressure of the gas above the liquid

Avagadro's Law: At a constant temperature and pressure, the volume occupied by a gas is proportional to the number of atoms
Avogadro's Law, also known as Avogadro's hypothesis or principle, is a critical concept in understanding the behaviour of gases. This law is an experimental gas law that relates the volume of a gas to the amount of substance within that gas, while holding temperature and pressure constant. In other words, it states that at a constant temperature and pressure, the volume of a gas is directly proportional to the number of atoms or molecules it contains. This principle is named after Amedeo Avogadro, who hypothesised this relationship in 1811/1812.
The law can be expressed mathematically as:
V ∝ n
V/n = k
Where:
- V represents the volume of the gas
- N is the amount of substance (measured in moles)
- K is a constant for a given temperature and pressure
This equation demonstrates that as the number of moles of gas increases, the volume of the gas also increases proportionally. Conversely, if the number of moles decreases, the volume decreases as well. This relationship is crucial for understanding and predicting the behaviour of gases, particularly in confined spaces.
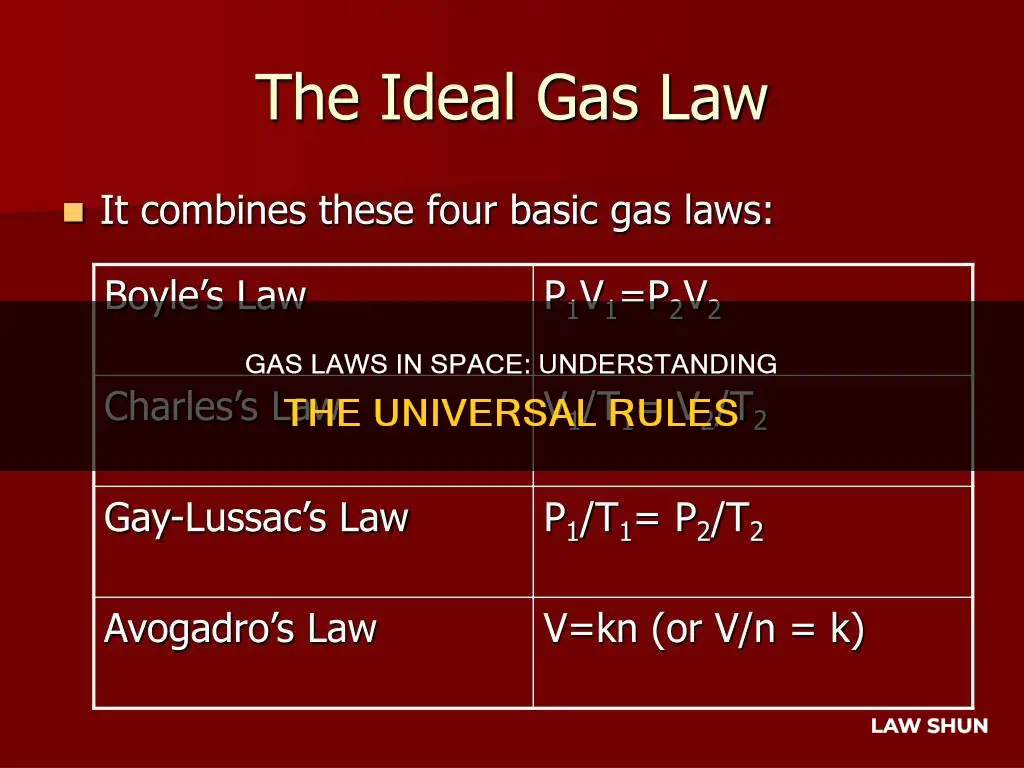
Avogadro's Law is a specific case of the Ideal Gas Law, which combines several other gas laws, including Boyle's Law, Charles' Law, and Gay-Lussac's Law. The Ideal Gas Law describes the relationship between the pressure, volume, and temperature of a gas, and it can be derived from Avogadro's Law.
Avogadro's Law has significant practical applications, especially in the field of space exploration and aeronautics. It is used to determine how much gas can be contained within a limited volume, such as in spacecraft or balloons. Additionally, it helps explain why gases with the same quantity of molecules occupy the same volume, as demonstrated in experimental studies by Charles Frédéric Gerhardt and Auguste Laurent.
In summary, Avogadro's Law provides a fundamental understanding of the relationship between the volume of a gas and the number of atoms or molecules it contains, as long as temperature and pressure are constant. This law has far-reaching implications and is an essential tool for scientists and engineers working with gases in various contexts, including space exploration.
Law and Ethics: Intertwined or Distinct?
You may want to see also

Boyle's Law: Used to calculate the pressure of gas that can be used without wasting space
Boyle's Law, also known as the Boyle-Mariotte Law, is a fundamental law in chemistry that describes the behaviour of a gas held at a constant temperature. The law was formulated by Anglo-Irish chemist Robert Boyle in 1662 and states that at a fixed temperature, the volume of a gas is inversely proportional to the pressure exerted by it. In simpler terms, when gas is introduced into an enclosed space, it adjusts to fit the available space, but the pressure it exerts on that space increases. This principle can be applied in space to determine how much pressure a gas can exert without bursting or wasting any space.
Mathematically, Boyle's Law can be expressed as:
> P ∝ (1/V)
> PV = k
Where:
- P represents the pressure exerted by the gas
- V represents the volume occupied by the gas
- K is a constant value that represents the temperature and amount of gas
This equation demonstrates that as the volume of a gas increases, its pressure decreases proportionally, and vice versa, as long as the temperature and the quantity of gas remain constant.
Boyle's Law can be applied in various scenarios, such as inflating a balloon. When air is blown into a balloon, the pressure of the air pushes against the rubber, causing the balloon to expand. If one end of the balloon is compressed, the volume decreases, and the pressure inside increases, causing the rest of the balloon to expand outward. However, there is a limit to how much the gas can be compressed, as the pressure will eventually become high enough to rupture the balloon.
Another example of Boyle's Law in action is scuba diving. If a scuba diver ascends too rapidly from a deep zone to the surface, the decrease in pressure can cause the gas molecules in their body to expand. These expanding gas bubbles can be harmful to the diver's organs and may even result in death. This illustrates how Boyle's Law can have significant implications in real-world situations.
In the context of space, Boyle's Law can be utilised to maximise the efficiency of gas usage. By understanding the relationship between pressure and volume, scientists and engineers can calculate the optimal amount of gas to use without wasting any valuable space. This application of Boyle's Law ensures that gas is used effectively and safely in the extreme conditions of space.
Family Law Statutes: Civil Cases in California
You may want to see also

Charles' Law: Similar to Boyle's Law but with a different variable held constant
Charles' Law and Boyle's Law are two very important laws concerning gases. They are widely used in fields such as chemistry, thermodynamics, aviation, and even military applications.
Boyle's Law, discovered by the Irish chemist Robert Boyle, states that for a fixed amount of an ideal gas, kept at a fixed temperature, pressure and volume are inversely proportional. In other words, as the pressure on a gas increases, the volume of the gas decreases because the gas particles are forced closer together.
Charles' Law, on the other hand, was first published by the French philosopher Joseph Louis Gay-Lussac, who credited the discovery to Jacques Charles. This law states that for a closed ideal gas system under constant pressure, the volume of the system is directly proportional to the temperature of the system. In simpler terms, when the temperature of the molecules increases, they move faster, creating more pressure on the container of the gas and increasing the volume, if the pressure remains constant.
To summarise, the key difference is that Charles' Law is defined for a system with constant pressure, while Boyle's Law is defined for a system with a constant temperature. Charles' Law deals with the relationship between temperature and volume, whereas Boyle's Law deals with the relationship between pressure and volume.
The President and the Rule of Law: Who Wins?
You may want to see also

Dalton's Law: The total pressure of a gas mixture is the sum of the partial pressures of each gas
Several gas laws apply in space, including Avogadro's Law, which helps determine the amount of gas in a limited space, and Boyle's Law, which helps calculate the amount of gas pressure that can be applied without bursting.
One of the key laws that applies in space is Dalton's Law, also known as the Law of Partial Pressures. This law is particularly relevant when dealing with gas mixtures, as it provides a way to calculate the total pressure exerted by the mixture.
Dalton's Law: Understanding Gas Mixtures
Dalton's Law states that the total pressure of a gas mixture is the sum of the partial pressures of each individual gas within the mixture. This law was first observed by John Dalton in 1801 and published the following year.
Mathematically, this can be expressed as:
\[ p_{total} = p_1 + p_2 + p_3 + ... + p_n \]
Where \( p_1, p_2, ..., p_n \) represent the partial pressures of each gas in the mixture.
The Ideal Gas Mixture Assumption
It's important to note that Dalton's Law is based on the assumption of ideal gas behaviour. In an ideal gas mixture, the molecules are so far apart that they don't interact with each other. This assumption holds true for most real-world gases.
Application in Gas Mixtures
Dalton's Law is particularly useful when dealing with gas mixtures, especially in closed containers. For example, consider a closed container with a mixture of nitrogen (N2), hydrogen (H2), and ammonia (NH3). By applying Dalton's Law, we can calculate the total pressure of the gas mixture:
\[ p = p_{N_2} + p_{H_2} + p_{NH_3} \]
Where:
- \( p \) is the total pressure of the gas mixture
- \( p_{N_2} \) is the partial pressure of nitrogen
- \( p_{H_2} \) is the partial pressure of hydrogen
- \( p_{NH_3} \) is the partial pressure of ammonia
Mole Fraction and Partial Pressure
Dalton's Law also helps establish the relationship between the mole fraction (\( x_i \)) and the partial pressure of an individual gas component in an ideal gas mixture:
\[ x_i = \frac{p_i}{p} = \frac{n_i}{n} \]
Where:
- \( x_i \) is the mole fraction of gas component i
- \( p_i \) is the partial pressure of gas component i
- \( p \) is the total pressure of the gas mixture
- \( n_i \) is the number of moles of gas component i
- \( n \) is the total number of moles in the gas mixture
Volume-Based Concentration
Dalton's Law also provides a way to determine the volume-based concentration of any individual gas component in a mixture:
\[ p_i = p_{total} \times c_i \]
Where:
- \( p_i \) is the partial pressure of gas component i
- \( p_{total} \) is the total pressure of the gas mixture
- \( c_i \) is the concentration of gas component i
Limitations of Dalton's Law
It's important to note that Dalton's Law has limitations and is not strictly followed by real gases. As pressure increases, the deviation from this law becomes more significant. This is because, at high pressures, the volume occupied by the gas molecules becomes significant compared to the free space between them, leading to increased intermolecular forces that substantially change the pressure exerted by the gas.
Criminal Law at Sea: Royal Caribbean's Legal Jurisdiction
You may want to see also

Henry's Law: The solubility of a gas in a liquid is directly proportional to the partial pressure of the gas above the liquid
In physical chemistry, Henry's Law is a gas law formulated by English chemist William Henry in the early 19th century, specifically in 1803. The law states that the solubility of a gas in a liquid is directly proportional to the partial pressure of the gas above the liquid.
Mathematically, this can be represented as:
\[ C = kP_{gas}\]
Where:
- C is the solubility of a gas at a fixed temperature in a particular solvent (in units of M or mL gas/L)
- K is Henry's Law constant (often in units of M/atm)
- Pgas is the partial pressure of the gas (often in units of atm)
In simpler terms, Henry's Law states that when a gaseous mixture (e.g. the atmosphere) is in contact with a solution, the amount of gas in that mixture that dissolves in the solution is directly proportional to the partial pressure of that gas. The partial pressure of a gas is the amount of pressure that the gas contributes to the total pressure of the gas mixture.
For example, when you open a bottle of carbonated soft drinks, the gas above the drink in its container is almost pure carbon dioxide, at a pressure higher than atmospheric pressure. After opening the bottle, this gas escapes, lowering the partial pressure of carbon dioxide above the liquid, which results in degassing as the dissolved carbon dioxide comes out of the solution.
Henry's Law is highly applicable in aerospace medicine, as it helps explain phenomena such as decompression sickness, and it provides a framework for treating such conditions. It is also relevant in underwater diving, where divers experience changes in the dissolution of gases in their blood during decompression.
Ohm's Law and Inductors: A Misunderstood Relationship
You may want to see also
Frequently asked questions
Avagadro's law.
It states that at a constant temperature and pressure, the number of gas atoms/molecules is directly proportional to the volume occupied by the gas.
Boyle's law.
It states that at a constant temperature and number of moles, the pressure exerted by the gas is inversely proportional to the volume occupied.
The formula for the ideal gas law is PV = nRT.







