Gustav Kirchhoff's Voltage Law (KVL) is his second fundamental law and is based on the law of conservation of energy. The law states that the algebraic sum of the potential voltage drops in a closed circuit is zero. In other words, the total energy gained per unit charge must equal the amount of energy lost per unit of charge. This is because a circuit loop is a closed conducting path, so no energy is lost.
| Characteristics | Values |
|---|---|
| Name | Kirchhoff's Voltage Law |
| Other Names | Kirchhoff's second law, Kirchhoff's loop rule, the law of conservation of voltage |
| Application | Series circuits, voltage divider circuits |
| Formula | ΣV = 0 |
| Description | The algebraic sum of all the voltage drops as you go around a closed circuit from some fixed point and return to the same point, taking polarity into account, is always zero. |
What You'll Learn

Conservation of charge
The conservation of charge is a fundamental principle in physics, stating that the total electric charge in an isolated system remains constant over time. In other words, charge can neither be created nor destroyed, only transferred between systems. This principle is supported by all empirical observations and has been proven experimentally.
The conservation of charge is based on the idea that the net charge of a system is determined by the number of electrons and protons it contains. As these subatomic particles carry electric charge, and cannot simply appear or disappear, the total charge of a system must remain the same. This is analogous to the conservation of energy and momentum, but the conservation of charge is considered more intuitive due to its direct dependence on the number of electrons and protons.
In an isolated system, the total charge is conserved, meaning that the amount of charge flowing into a volume is equal to the amount flowing out. This can be expressed mathematically as:
${\displaystyle {\frac {\mathrm {d} Q}{\mathrm {d} t}}={\dot {Q}}_{\rm {IN}}(t)-{\dot {Q}}_{\rm {OUT}}(t)}$
Where dQ/dt is the rate of change of charge in a specific volume at time t, ${\dot {Q}}_{\rm {IN}}$ is the amount of charge flowing into the volume, and ${\dot {Q}}_{\rm {OUT}}$ is the amount of charge flowing out.
The conservation of charge is also connected to Kirchhoff's Voltage Law (KVL), which is the second of Gustav Kirchhoff's fundamental laws for circuit analysis. KVL states that the algebraic sum of all voltages around a closed loop in a circuit is equal to zero. This is because a circuit loop is a closed conducting path, and therefore, no energy is lost. This principle is also known as the conservation of energy.
In summary, the conservation of charge is a fundamental principle stating that the total electric charge in an isolated system is constant, and it is supported by both theoretical and experimental evidence. This principle has important applications in various fields, including circuit analysis and particle physics.
Mentalism Law: Mastering the Mind for Success
You may want to see also

Conservation of energy
Gustav Kirchhoff's Voltage Law (KVL) is his second fundamental law and it deals with the conservation of energy around a closed circuit path.
The law states that for a closed-loop series path, the algebraic sum of all the voltages around any closed loop in a circuit is equal to zero. This is because a circuit loop is a closed conducting path, so no energy is lost. In other words, the algebraic sum of all the potential differences around the loop must be equal to zero.
Kirchhoff's idea is commonly known as the Conservation of Energy. Moving around a closed loop or circuit means you will end up back where you started, with the same initial potential and no loss of voltage around the loop. Therefore, any voltage drops around the loop must be equal to any voltage sources met along the way.
Kirchhoff's Voltage Law is based on the law of conservation of energy, which states that the total energy gained per unit charge must equal the amount of energy lost per unit of charge.
In simple terms, going around a closed loop, Kirchhoff tells you that the sum of the potential differences is zero. For example, in a simple series circuit with three nodes, the sum of the voltages between each node will be zero.
Premption Doctrine: Reviving Dormant Laws?
You may want to see also

Kirchhoff's Voltage Law
KVL states that the algebraic sum of all voltages in a loop must equal zero. In other words, the algebraic sum of all voltage sources and voltage drops within a closed loop must be equal to zero since the algebraic sum of the voltage drops equals the algebraic sum of the voltage sources. This is known as the Conservation of Energy.
Massachusetts PHI: Laws and Compliance
You may want to see also

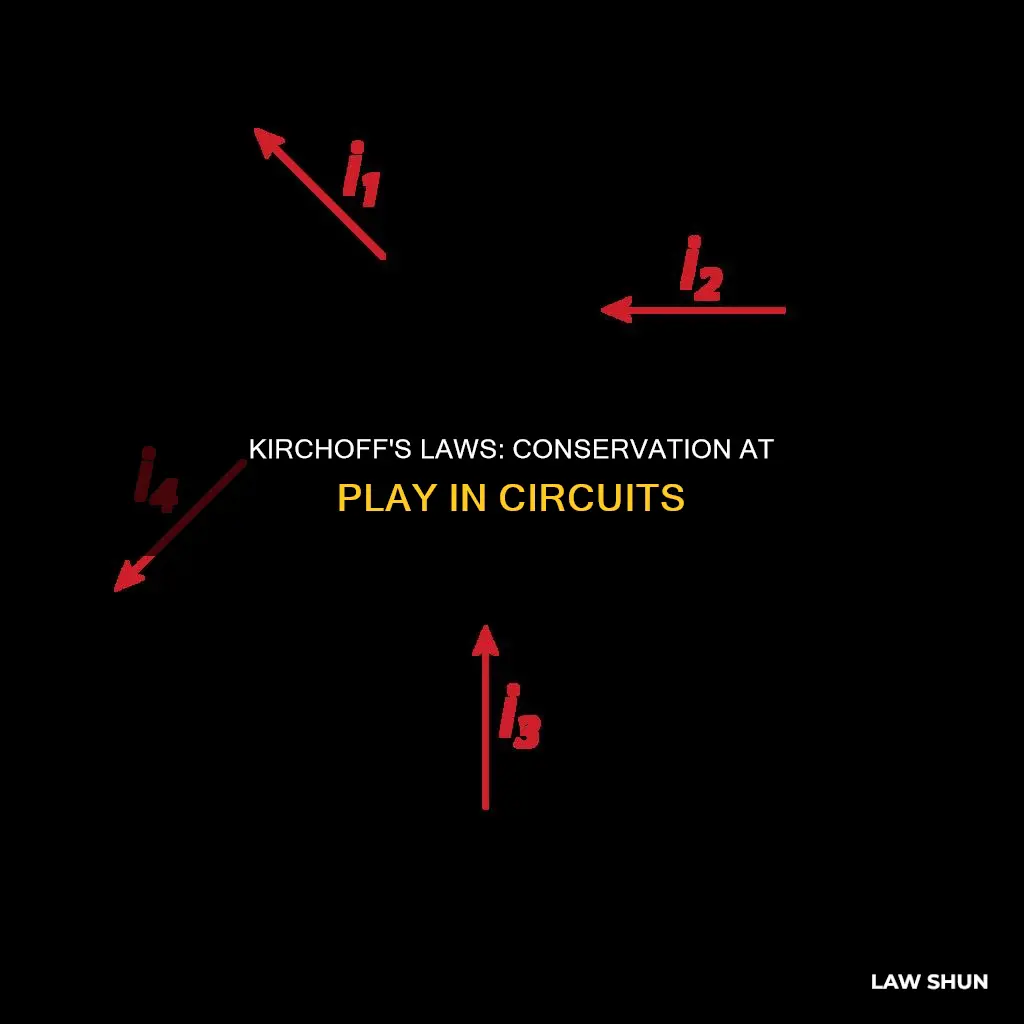
Kirchhoff's Current Law
Σ I_IN = Σ I_OUT
Or
I(exit) + I(enter) = 0
This law is based on the conservation of charge, as no charge is lost in the circuit. It is applicable to any type of lumped network and can be used to analyse both parallel and series circuits.
KCL is one of two laws formulated by German physicist Gustav Kirchhoff in 1845, generalising the work of Georg Ohm. The other law is Kirchhoff's Voltage Law (KVL), also known as Kirchhoff's Second Law or Loop Rule. Together, these laws form a basic tool for analysing electrical circuits and are widely used in electrical engineering.
Understanding Lemon Law Recall Rights and Timelines
You may want to see also

Kirchhoff's Junction Law
The law states that for any node (junction) in an electrical circuit, the sum of currents flowing into that node is equal to the sum of currents flowing out of that node. In other words, the total current entering a circuit junction is exactly equal to the total current leaving the same junction. This is because the current is conserved around the junction, with no loss of current.
Mathematically, Kirchhoff's Current Law can be stated as:
> {\displaystyle \sum _{i=1}^{n}I_{i}=0} where n is the total number of branches with currents flowing towards or away from the node.
This can also be written as:
> Σ IIN = Σ IOUT
HIV Status: Mandatory Reporting Law Applicability
You may want to see also
Frequently asked questions
Kirchhoff's Voltage Law (KVL) is a law that deals with the conservation of energy around a closed circuit path. It states that the algebraic sum of all the voltages around any closed loop in a circuit is equal to zero.
The principle behind Kirchhoff's Voltage Law is the conservation of energy. This is because a circuit loop is a closed conducting path, so no energy is lost.
Kirchhoff's Voltage Law is based on the law of conservation of energy, which states that the total energy gained per unit charge must equal the amount of energy lost per unit of charge.
The formula for Kirchhoff's Voltage Law is ΣV = 0, which means that the algebraic sum of the potential differences in any loop must be equal to zero.
Kirchhoff's Voltage Law is useful for circuit analysis, especially when dealing with series circuits. It allows us to calculate the total resistance, circuit current, current through each resistor, and voltage drop across each resistor in a series circuit.







