The law of cosines, also known as the cosine rule, is used to find the unknown side of a triangle when the lengths of the other two sides are given and the angle between them is known. The formula for the law of cosines is given as:
c^2 = a^2 + b^2 - 2*a*b*cos(C)
Where a, b, and c are the sides of the triangle, and C is the angle between sides a and b.
The law of cosines can be applied to all types of triangles, not just right triangles, and can be used to find any unknown side or angle.
| Characteristics | Values |
|---|---|
| Law of Cosines Formula | a2 = b2 + c2 − 2ab cos(C) |
| Law of Cosines Definition | The square of any one side of a triangle is equal to the difference between the sum of squares of the other two sides and double the product of other sides and cosine angle included between them |
| Application | Computing the third side of a triangle given two sides and their enclosed angle, and for computing the angles of a triangle if all three sides are known |
| Generalization | The law of cosines generalizes the Pythagoras theorem, which applies to a right triangle |
What You'll Learn

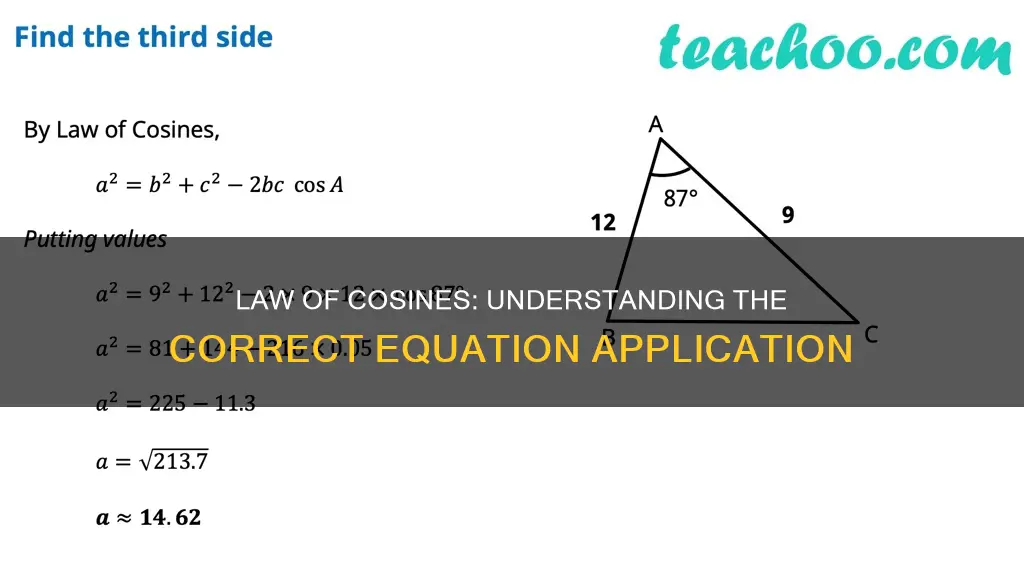
Finding the third side of a triangle
The law of cosines, also known as the cosine rule, is a formula that relates the lengths of the sides of a triangle to the cosine of one of its angles. It can be used to find the length of the third side of a triangle when the length of the other two sides and the angle between them are known.
Let's say we have a triangle with sides of length a, b, and c, and angles of α, β, and γ. The law of cosines can be applied in three different ways, depending on which side and angle we want to find:
Finding side a when sides b and c, and angle α are known:
A^2 = b^2 + c^2 - 2*b*c*cos(α)
Or
A = sqrt(b^2 + c^2 - 2*b*c*cos(α))
Finding side b when sides a and c, and angle β are known:
B^2 = a^2 + c^2 - 2*a*c*cos(β)
Or
B = sqrt(a^2 + c^2 - 2*a*c*cos(β))
Finding side c when sides a and b, and angle γ are known:
C^2 = a^2 + b^2 - 2*a*b*cos(γ)
Or
C = sqrt(a^2 + b^2 - 2*a*b*cos(γ))
Understanding Hooke's Law: Force Magnitude and Elastic Limits
You may want to see also

Finding angles of a triangle
The law of cosines, also known as the cosine rule, relates the lengths of the sides of a triangle to the cosine of one of its angles. It can be used to find the unknown angles of a triangle when the lengths of all three sides are known.
The formula for finding the unknown angles using the law of cosines is:
Cos α = [b^2 + c^2 - a^2]/2bc
Cos β = [a^2 + c^2 - b^2]/2ac
Cos γ = [b^2 + a^2 - c^2]/2ab
Where a, b, and c are the lengths of the sides of a triangle.
For example, let's say we have a triangle with sides a = 10 cm, b = 7 cm, and c = 5 cm, and we want to find angle x.
Using the formula for cos x:
Cos x = (b^2 + c^2 - a^2)/(2*b*c)
We can substitute the given side lengths:
Cos x = (7^2 + 5^2 - 10^2)/(2*7*5)
Cos x = (49 + 25 - 100)/(70)
Cos x = 74/70
Cos x = 0.65
So, the measure of angle x in the triangle is approximately 49.16 degrees or 0.86 radians (rounded to two decimal places).
The law of cosines is a versatile tool in trigonometry, helping us find unknown sides and angles in triangles when sufficient information is provided.
Understanding Castle Law: Apartments and Their Legal Standing
You may want to see also

Finding missing sides
The law of cosines, also known as the cosine rule, relates the lengths of the sides of a triangle to the cosine of one of its angles. It can be used to find the missing sides and angles of any triangle, including right triangles and oblique triangles.
To find a missing side of a triangle using the law of cosines, follow these steps:
- Identify the known values: You need to know the lengths of two sides of the triangle and the measure of the angle between them. Let's call these sides a, b, and c, and the angle between them α.
- Apply the law of cosines formula: Depending on which side is missing, you will use one of the following formulas:
- To find side a: Use the formula a^2 = b^2 + c^2 - 2bccos(α).
- To find side b: Use the formula b^2 = a^2 + c^2 - 2accos(α).
- To find side c: Use the formula c^2 = a^2 + b^2 - 2abcos(α).
- Solve for the unknown side: Plug the known values into the appropriate formula and solve for the missing side.
- Ensure appropriate units: Express the obtained result with suitable units, such as inches, feet, or degrees.
For example, let's say we have a triangle with sides a = 10 cm, b = 7 cm, and an angle α = 60 degrees between them. We want to find the length of the missing side, c. Using the law of cosines formula for side c, we get:
C^2 = a^2 + b^2 - 2*a*b*cos(α)
C^2 = (10 cm)^2 + (7 cm)^2 - 2*(10 cm)*(7 cm)*cos(60 degrees)
C^2 = 100 cm^2 + 49 cm^2 - 2*70 cm^2*0.5
C^2 = 149 cm^2 - 70 cm^2
C^2 = 79 cm^2
C ≈ 8.89 cm
So, the length of the missing side c is approximately 8.89 cm.
The law of cosines is a versatile tool that can be applied to various scenarios involving triangles, making it an essential concept in trigonometry.
Cooling Law: Predicting Death with Science
You may want to see also

Finding unknown angles
The law of cosines, also known as the cosine rule, establishes a relationship between the lengths of a triangle's sides and the cosine of its angles. It is used to find the unknown sides or angles of a triangle.
To find the unknown angles of a triangle using the law of cosines, we need to know the lengths of all three sides of the triangle. The formula to find the unknown angles is:
For angle A:
Cos A = (b^2 + c^2 - a^2) / (2bc)
For angle B:
Cos B = (a^2 + c^2 - b^2) / (2ac)
For angle C:
Cos C = (b^2 + a^2 - c^2) / (2ab)
Where:
- A, B, and C are the angles of the triangle
- A, b, and c are the lengths of the sides of the triangle
For example, let's say we have a triangle with sides a = 4 cm, b = 9 cm, and c = 6 cm, and we want to find angle C. We would use the formula for angle C:
Cos C = (b^2 + a^2 - c^2) / (2ab)
Cos C = (9^2 + 4^2 - 6^2) / (2 * 9 * 4)
Cos C = (81 + 16 - 36) / 72
Cos C = 51 / 72
Cos C ≈ 0.708
Now, to find the measure of angle C, we need to take the arccosine (inverse cosine) of both sides:
C = arccos(cos C)
C = arccos(0.708)
Using a calculator, we find that C ≈ 44.1 degrees.
So, the measure of angle C in the triangle is approximately 44.1 degrees.
Sunshine Law: Does it Shine on Condo Boards?
You may want to see also

Finding the length of a triangle side
The law of cosines, also known as the cosine rule, is used to find the length of a triangle side when the length of the other two sides and the angle between them are known.
The law of cosines formula is:
A^2 = b^2 + c^2 - 2bc x cos(A)
Where:
- A, b, and c are the lengths of the three sides of a triangle, and
- A is the angle between sides b and c.
For example, if we have a triangle with sides of 72 inches, 50 inches, and x inches, with an angle of 49 degrees between the two known sides, we can use the law of cosines to find the length of the third side, x:
X^2 = (72)^2 + (50)^2 - 2(72)(50) x cos(49)
X^2 = 5184 + 2500 - 7200 x 0.6569
X^2 = 7684.31
X = 87.6 inches
The law of cosines can also be used to find the length of the unknown side of a triangle when the length of all three sides is known (SSS congruence). In this case, we first need to find one angle using the cosine law, and then we can find the other two angles using the same law and the angle sum property of triangles (the sum of all three angles in a triangle is equal to 180 degrees).
The law of cosines is a useful tool in trigonometry, helping to establish a relationship between the lengths of sides of a triangle and the cosine of its angles. It generalizes the Pythagorean theorem, which only applies to right triangles.
Copper Cycle Chemistry: Laws and Applications
You may want to see also
Frequently asked questions
The law of cosines relates the lengths of a triangle's sides to the cosine of one of its angles. It is used to find the third side of a triangle when the lengths of the other two sides and the angle between them are known.
The law of cosines states that the square of any one side of a triangle is equal to the sum of the squares of the other two sides minus twice the product of the other two sides multiplied by the cosine of the angle between them.
The law of cosines can be written as:
a^2 = b^2 + c^2 - 2*b*c*cos(A)
or
c^2 = a^2 + b^2 - 2*a*b*cos(C)
The Pythagorean theorem applies only to right triangles, whereas the law of cosines can be applied to all types of triangles.
To find the third side of a triangle using the law of cosines, you need to know the lengths of the other two sides and the angle between them. Plug these values into the equation:
c^2 = a^2 + b^2 - 2*a*b*cos(C), where a, b, and c are the sides of the triangle, and C is the angle between sides a and b.







