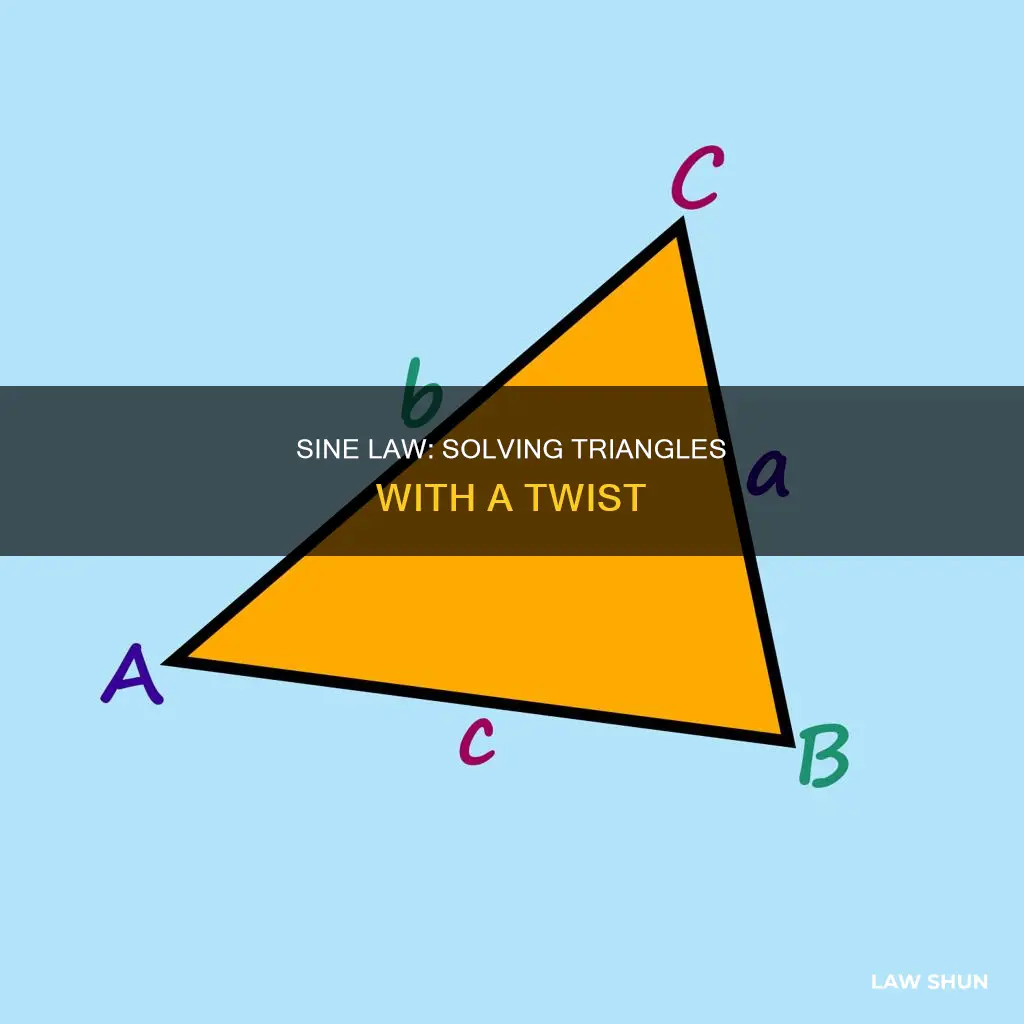
The law of sines, also known as the sine rule or sine formula, is used to find the unknown angle or side of a triangle. It can be applied to both right triangles and oblique triangles. The law of sines states that the ratio of the side length of a triangle to the sine of the opposite angle is the same for all three sides. In other words, if a, b, and c are the sides of a triangle, and A, B, and C are the angles, then (a/sin A) = (b/sin B) = (c/sin C). This law can be used when we know two angles and one side or two angles and one included side, which is known as the ASA (Angle-Side-Angle) or AAS (Angle-Angle-Side) criteria.
| Characteristics | Values |
|---|---|
| Name | Law of Sines, Sine Law, Sine Rule, Sine Formula |
| Purpose | Used to find the unknown angle or side of a triangle |
| Application | Can be used when we have ASA (Angle-Side-Angle) or AAS (Angle-Angle-Side) criteria |
| Formula | a/sin A = b/sin B = c/sin C |
| Application | Can be applied to both the right triangle and oblique triangle or scalene triangle |
What You'll Learn

Finding the unknown angle of a triangle
The law of sines, also known as the sine rule, can be used to find the unknown angle of a triangle. This law applies to any triangle, not just a right triangle, and relates the sides and angles of a triangle.
The law of sines states that the ratio of the side length of a triangle to the sine of the opposite angle is the same for all three sides. In other words, if a, b, and c are the sides of a triangle, and A, B, and C are the angles, then the sine rule or the law of sines is given by:
> (a/sin A) = (b/sin B) = (c/ sin C)
To find an unknown angle using the law of sines, you can follow these steps:
- Substitute the known values into the formula. For example, if you know sides a and b, and angle A, you can use the formula a/sin A = b/sin B to solve for angle B.
- Remove the fraction that is unhelpful. For example, if you are solving for angle B, you can rewrite the formula as sin B/b = sin A/a.
- Solve the remaining equation. Using the example above, input the values into a calculator to find the measure of angle B.
It is important to note that when applying the law of sines to solve a triangle, there may be two possible solutions due to the potential for two different triangles to be created using the given information. Additionally, the law of sines requires at least two angles and their respective side measurements to work.
Doctors' Fee Autonomy: Legal or Ethical Dilemma?
You may want to see also

Finding the unknown side of a triangle
The law of sines is used in trigonometry to find the unknown side or angle of a triangle. It is also known as the sine rule, sine law, or sine formula. This law is applied to both right triangles and oblique triangles or scalene triangles. The law of sines defines the relationship between the sides and angles of a triangle.
The law of sines formula can be written as:
> a/sinA = b/sinB = c/sinC
Where a, b, and c are the sides of a triangle, and A, B, and C are the angles. This means that the ratio of the side length of a triangle to the sine of the opposite angle is the same for all three sides. For example, if we have a triangle with sides a = 10, c = 12.5, and angle C = 42º, we can find the length of side b using the law of sines:
> a/sinA = b/sinB = c/sinC
> 10/sinA = 12.5/sinB = b/sin42º
> b = 12.5 / sinB * sin42º
The law of sines can be used to find the unknown side of a triangle when two angles and one side, or two angles and one included side, are given. This is known as the ASA (Angle-Side-Angle) or AAS (Angle-Angle-Side) criteria. For example, if we have a triangle with sides a = 18 and b = 20, and angle A = 26º, we can use the sine rule to find the measure of angle B:
> sinA/a = sinB/b
> sin26º/18 = sin B/20
It's important to note that there may be two possible solutions when using the law of sines to solve a triangle, as the given information can sometimes create two different triangles.
Oregon Retirement Law: Opt-Out Options for Employees
You may want to see also

Solving oblique triangles
Any triangle that is not a right triangle is an oblique triangle. This means that solving an oblique triangle involves finding the measurements of all three angles and all three sides. To do this, we need to start with at least three of the values, including at least one of the sides.
There are three possible oblique triangle problem scenarios: ASA (angle-side-angle), AAS (angle-angle-side), and SSA (side-side-angle). In ASA, we know the measurements of two angles and the included side. In AAS, we know the measurements of two angles and a side that is not between the known angles. In SSA, we know the measurements of two sides and an angle that is not between the known sides.
The Law of Sines can be used to solve any oblique triangle. The law states that each side of a triangle is proportional to the sine of the opposite angle. In other words, the ratio of the measurement of an angle to the length of its opposite side will be equal to the other two ratios of angle measure to opposite side. All proportions will be equal.
The Law of Sines is represented by the following equations:
$$
\co: 1,3,6<\frac{\sin \alpha }{a}=\frac{\sin \beta }{b}=\frac{\sin \gamma }{c}>
$$
$$
\co: 1<\frac{a}{\sin \alpha }=\frac{b}{\sin \beta }=\frac{c}{\sin \gamma }>
$$
To solve an oblique triangle, use any pair of applicable ratios. To find an unknown side, we need to know the corresponding angle and a known ratio. For example, consider the following problem: A = 35°, B = 49°, and a = 7. First, calculate the third angle, C: C = 180° - 35° - 49° = 96°. Then, using the Law of Sines, b and c can be calculated: b ≈ 9.21, and c ≈ 12.13.
Confederate Article Invalidates State Law: What's the Verdict?
You may want to see also

Finding the area of a triangle
The law of sines is used to find the unknown side or angle of a triangle. It can be applied to both right triangles and oblique triangles or scalene triangles. The law of sines is generally used to solve a triangle when we know two angles and one side or two angles and one included side. This means that the law of sines can be used when we have ASA (angle-side-angle) or AAS (angle-angle-side) criteria. The law of sines can also be used to find the unknown side of a triangle when two sides and one non-included angle are given.
The law of sines formula can be written as:
$$\frac{\sin A}{a} = \frac{\sin B}{b} = \frac{\sin C}{c}$$
Or
$$\frac{a}{\sin A} = \frac{b}{\sin B} = \frac{c}{\sin C}$$
Where $a, b, c$ are the sides of a triangle, and $A, B, C$ are the angles.
To find the area of a triangle, you can use the formula for the area of a parallelogram. You can also use the sine ratio, which is especially handy when dealing with right triangles. To use the sine ratio, you need the lengths of two sides and the measurement of the included angle.
State Powers: Blocking Federal Laws?
You may want to see also

Using Sine Rule to find missing angle or side
The Sine Rule, also known as the Law of Sines, can be used to find a missing angle or side in any triangle, not just right-angled triangles. It defines the relationship between the sides of a triangle and their respective sine angles.
To use the Sine Rule, you need to have at least two angles and their respective side measurements. The rule states that the ratio of the side length of a triangle to the sine of the opposite angle is the same for all three sides. This can be written as:
> a/sin A = b/sin B = c/sin C
Where a, b, and c are the sides of a triangle, and A, B, and C are the angles.
To find a missing angle, you can use the formula:
> sin A/a = sin B/b
To find a missing side, you can rearrange the formula as follows:
> a/sin A = b/sin B
Once you have substituted the given values into the equation, you can solve for the unknown side or angle.
For example, let's say we have a triangle with sides a = 10 units, c = 12.5 units, and angle C = 42 degrees. We want to find the value of angle A. Using the Sine Rule formula, we can set up the equation as follows:
> a/sin A = c/sin C
> 10/sin A = 12.5/sin 42
Now, we can solve for sin A:
> sin A = (10/12.5) x sin 42
> sin A = 0.8
Finally, we can find the angle A by taking the arcsin of both sides:
> A = arcsin(0.8)
> A ≈ 53.13 degrees
Congress' Power: Laws Targeting Individuals?
You may want to see also
Frequently asked questions
The law of sines is used to determine the unknown side of a triangle when two angles and sides are given. It defines the ratio of sides of a triangle and their respective sine angles, which are equivalent to each other.
The law of sines can be used when we have ASA (Angle-Side-Angle) or AAS (Angle-Angle-Side) criteria. It can be used to find the unknown angle or side of an oblique triangle (non-right triangle) when we know the measurements of at least two angles and one side.
The formula for the law of sines is given by (a/sin A) = (b/sin B) = (c/ sin C). To find the unknown side, you need to know the measurements of two angles and one side, or two angles and one included side.
Yes, the law of sines can be applied to both right triangles and oblique triangles. However, it is generally used when at least one angle is unknown, as the trigonometric functions are usually defined in terms of right triangles.







