Kepler's Laws: Do Artificial Objects Obey The Same Rules?

Kepler's laws of planetary motion describe how planetary bodies orbit the Sun. They state that:

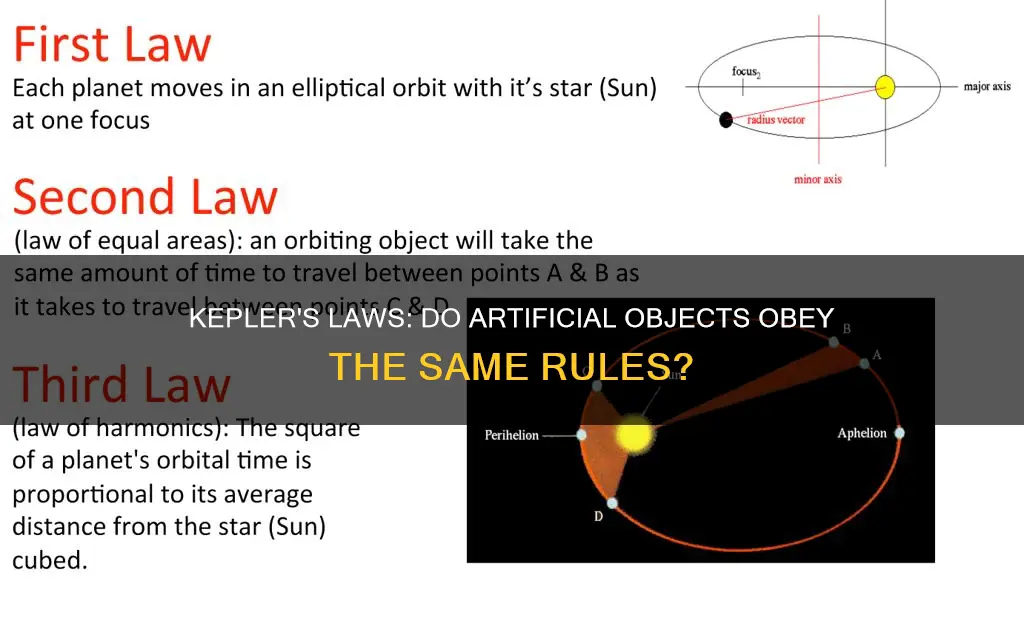
1. Planets move in elliptical orbits with the Sun as a focus.

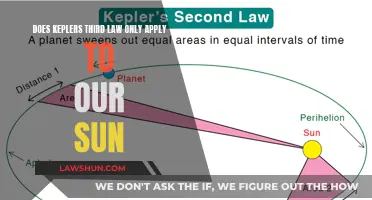
2. A planet covers the same area of space in the same amount of time no matter where it is in its orbit.

3. A planet’s orbital period is proportional to the size of its orbit (its semi-major axis).

These laws were formulated by the German astronomer Johannes Kepler, who believed in the Copernican model of the solar system, which placed the Sun at its centre. Kepler's laws apply to the motions of natural and artificial satellites, as well as to stellar systems and extrasolar planets.

Kepler's laws apply to all bodies satisfying two conditions: the mass of the orbiting object is small compared to the mass of the object it orbits, and the system is isolated from other massive objects

Kepler's laws apply to all bodies that satisfy two conditions: the mass of the orbiting object is small compared to the mass of the object it orbits, and the system is isolated from other massive objects. These laws describe the motion of any two bodies in gravitational orbit around each other, and all that is required is an inverse square central attractive force between the two bodies.

Kepler's laws consist of three laws that describe how planetary bodies orbit the Sun. Kepler's first law states that the orbit of each planet around the Sun is an ellipse with the Sun at one focus. The second law states that each planet moves so that an imaginary line drawn from the Sun to the planet sweeps out equal areas in equal times. The third law states that the squares of the orbital periods of the planets are directly proportional to the cubes of the semi-major axes of their orbits.

These laws were formulated by Johannes Kepler, who lived in Graz, Austria, during the tumultuous early 17th century. Kepler was a German mathematician who worked as an assistant to the famous astronomer Tycho Brahe. Kepler's laws replaced circular orbits and epicycles in the heliocentric theory of Nicolaus Copernicus with elliptical orbits and explained how planetary velocities vary.

Kepler's laws apply to natural and artificial satellites

Kepler's laws of planetary motion describe how planetary bodies orbit the Sun. They apply to all bodies satisfying two conditions: the mass of the orbiting object is small compared to the mass of the object it orbits, and the system is isolated from other massive objects.

Kepler's laws are useful for understanding the motions of both natural and artificial satellites. They can be applied to any body that satisfies the two conditions above and is subject to an inverse-square central attractive force, such as gravitational or Coulombic systems.

Kepler's three laws can be summarised as follows:

• All planets move about the Sun in elliptical orbits, having the Sun as one of the foci.
• A radius vector joining any planet to the Sun sweeps out equal areas in equal lengths of time.
• The squares of the sidereal periods (of revolution) of the planets are directly proportional to the cubes of their mean distances from the Sun.

These laws were formulated by Johannes Kepler based on the astronomical observations of Tycho Brahe. They were instrumental in Isaac Newton deriving his theory of universal gravitation, which explains the unknown force behind Kepler's third law.

Kepler's laws apply to all inverse-square-law forces

Kepler's laws of planetary motion describe the orbits of planets around the Sun. These laws replaced the heliocentric theory of Nicolaus Copernicus, which stated that planets moved in circular orbits and epicycles. Kepler's laws state that:

• The orbit of a planet is an ellipse with the Sun at one of the two foci.
• A line joining a planet and the Sun sweeps out equal areas during equal intervals of time.
• The square of a planet's orbital period is proportional to the cube of the length of the semi-major axis of its orbit.

These laws were derived by Johannes Kepler from the highly precise astronomical observations of Tycho Brahe. Kepler's laws can be applied to all inverse-square-law forces, not just gravitational forces. This includes electric, light, sound, and radiation phenomena.

The usefulness of Kepler's laws extends to the motions of natural and artificial satellites, as well as to stellar systems and extrasolar planets. Kepler's laws can be used to describe the motion of artificial satellites, such as those orbiting the Earth or other planets in our solar system.

In summary, Kepler's laws apply to all inverse-square-law forces and can be used to describe the motion of both natural and artificial objects in orbit.

Kepler's laws apply to all bodies in the solar system

Kepler's laws of planetary motion describe how all bodies in the solar system orbit the Sun. They were formulated by the German astronomer Johannes Kepler, who, in the early 17th century, studied Tycho Brahe's extensive and meticulous observations of planetary motion. Kepler's laws describe the following:

• All planets move in elliptical orbits with the Sun at one focus. The orbit of each planet is an ellipse, with the Sun located at one of the two foci. This was a significant improvement on the Copernican model, which assumed circular orbits.
• A line joining a planet and the Sun sweeps out equal areas during equal intervals of time. In other words, a planet covers the same area of space in the same amount of time, no matter where it is in its orbit. This law establishes that a planet's speed varies along its orbit, and it is fastest when closest to the Sun.
• The square of a planet's orbital period is proportional to the cube of the length of the semi-major axis of its orbit. This law implies that a planet's orbital period increases with the size of its orbit.

These laws were crucial in Isaac Newton's formulation of his theory of universal gravitation, which explains the unknown force behind Kepler's third law. They are also applicable beyond the solar system, to all bodies that satisfy the following two conditions:

• The mass of the orbiting object is small compared to the mass of the object it orbits.
• The system is isolated from other massive objects.

Kepler's laws, therefore, apply to all bodies in the solar system that meet these conditions, including artificial satellites.

Kepler's laws apply to all objects orbiting the same primary

Kepler's laws of planetary motion describe the orbits of planets around the Sun. They state that:

• The orbit of a planet is an ellipse with the Sun at one of the two foci.
• A line segment joining a planet and the Sun sweeps out equal areas during equal intervals of time.
• The square of a planet's orbital period is proportional to the cube of the length of the semi-major axis of its orbit.

These laws apply to all bodies orbiting the same primary body, not just planets. This includes natural and artificial satellites, as well as extrasolar planets and stellar systems. For example, in 1621, Johannes Kepler noted that his third law applied to the four brightest moons of Jupiter.

The laws can be stated more generally as:

• All bodies move about the primary body in elliptical orbits, having the primary body as one of the foci.
• A radius vector joining any body to the primary body sweeps out equal areas in equal lengths of time.
• The squares of the sidereal periods (of revolution) of the bodies are directly proportional to the cubes of their mean distances from the primary body.

Kepler's laws are purely descriptive and do not take into account the gravitational interactions of the various bodies with one another. However, they were crucial in Isaac Newton deriving his theory of universal gravitation, which explains the unknown force behind Kepler's third law.

Frequently asked questions