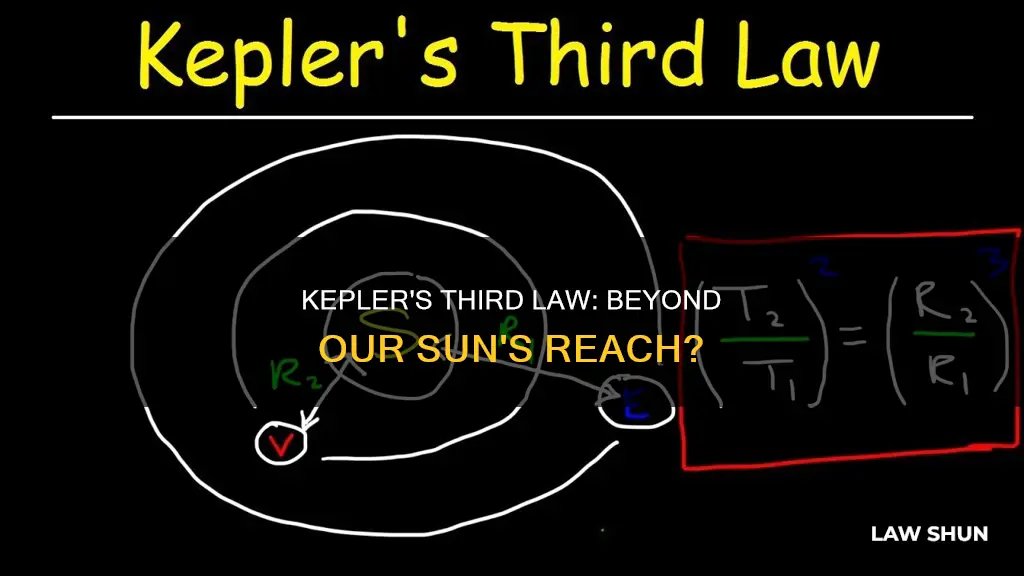
Kepler's Third Law, the last of three revolutionary theorems by German astronomer Johannes Kepler, explains planetary orbits around the Sun. Kepler's laws describe how planets move in elliptical orbits with the Sun as a focus, and that a planet's orbital period is proportional to the size of its orbit. This law applies to the solar system and beyond, as astronomers have used it to calculate the orbits and masses of over 4,000 planets outside the solar system. Kepler's Third Law can be applied to systems with stars of different masses to our Sun by making a slight adjustment to the formula to account for the variation in stellar mass.
| Characteristics | Values |
|---|---|
| What does Kepler's Third Law describe? | The relationship between the distance of planets from the Sun and their orbital periods. |
| What is the formula for Kepler's Third Law? | P2 = a3 |
| What does P refer to? | The period of a planet's orbit |
| What does a refer to? | The size of a planet's orbit or the semi-major axis of its ellipse |
| What is the formula for Kepler's Third Law when applied to Newton's Laws of Motion and Newton's Law of Gravity? | (M1+M2)/M13 = P2/a^3 |
| What do M1 and M2 refer to? | The masses of the two orbiting objects in solar masses |
| Can Kepler's Third Law be applied to objects beyond our solar system? | Yes, it has been used to calculate the orbits and masses of over 4,000 exoplanets |
What You'll Learn

Planetary orbits are ellipses
The first property of an ellipse is that it is defined by two points, each called a focus, and together called foci. The sum of the distances to the foci from any point on the ellipse is always a constant. For planetary orbits, this means that the planet and its star orbit a mutual centre of mass. However, because the star's mass is much larger, this centre of mass is often beneath its surface.
The second property of an ellipse is the amount of flattening, which is called eccentricity. The flatter the ellipse, the more eccentric it is. Each ellipse has an eccentricity with a value between zero (a circle) and one (a parabola).
The third property of an ellipse is that the longest axis is called the major axis, while the shortest is called the minor axis. Half of the major axis is called the semi-major axis.
Kepler's laws of planetary motion replaced circular orbits in the heliocentric theory of Nicolaus Copernicus with elliptical orbits and explained how planetary velocities vary. Kepler's laws were also instrumental in Isaac Newton deriving his theory of universal gravitation, which explains the unknown force behind Kepler's Third Law.
Consumer Law: Applicability in B2B Contracts Explored
You may want to see also

The Sun is at one focus
Kepler's first law states that the orbit of a planet is an ellipse with the Sun at one of the two foci. This means that the Sun is not at the centre of the orbit, but at a focal point of the elliptical orbit. The planet follows the ellipse in its orbit, so the distance between the planet and the Sun is constantly changing as the planet moves.
The orbit of every planet is an ellipse with the Sun at one of the two foci. Mathematically, an ellipse can be represented by the formula:
> r = p / (1 + ε cos θ)
Where p is the semi-latus rectum, ε is the eccentricity of the ellipse, r is the distance from the Sun to the planet, and θ is the angle to the planet's current position from its closest approach, as seen from the Sun.
For an ellipse, 0 < ε < 1. In the limiting case of ε = 0, the orbit is a circle with the Sun at the centre. At θ = 0°, perihelion, the distance is at a minimum:
> rmin = p / (1 + ε)
At θ = 180°, aphelion, the distance is at a maximum:
> rmax = p / (1 - ε)
The semi-major axis, a, is the arithmetic mean between rmin and rmax:
> a = (rmax + rmin) / 2 = p / (1 - ε^2)
The semi-minor axis, b, is the geometric mean between rmin and rmax:
> b = sqrt(rmax * rmin) = p / sqrt(1 - ε^2)
The eccentricity, ε, is the coefficient of variation between rmin and rmax:
> ε = (rmax - rmin) / (rmax + rmin)
The area of the ellipse is:
> A = πab
The special case of a circle is ε = 0, resulting in r = p = rmin = rmax = a = b and A = πr^2.
The Sun's centre is always located at one focus of the orbital ellipse, with the other focus being empty. The orbit of a planet is not a circle with epicycles, but an ellipse. The Sun is not at the centre, but at a focal point of the elliptical orbit.
American Laws on Reservations: An Indian Jurisdiction
You may want to see also

A planet's speed varies
Kepler's Second Law states that a planet does not move at a constant speed along its orbit. Instead, its speed varies so that the line joining the centres of the Sun and the planet sweeps out equal parts of an area in equal time intervals.
When a planet is closest to the Sun, at a point called the perihelion, the line joining the two is shorter. This means that the area it traces is shallower. Therefore, to map out the same area in the same amount of time, the planet must move more quickly. So, when a planet is at perihelion, it is moving at its quickest.
Conversely, when a planet is furthest from the Sun, at a point called the aphelion, the line joining the two is longer. This means that the area it traces is deeper. So, to map out the same area in the same amount of time, the planet must slow down. Thus, when a planet is at aphelion, it is moving at its slowest.
The orbital radius and angular velocity of a planet in an elliptical orbit will vary. This means that the distance from the Sun to the planet is constantly changing as the planet goes around its orbit.
Kepler's Second Law can be used to show that the velocity of a planet changes as it moves along its orbit. This law also demonstrates that the angular momentum of a planet with a radially symmetric force is conserved.
English Law in the US: Who Rules?
You may want to see also

Orbital period is proportional to orbit size
Kepler's Third Law, or the Law of Harmony, states that the square of the orbital period of a planet is proportional to the cube of the semi-major axis of its orbit. In other words, the longer the orbital period, the larger the orbit size. This law applies to all planets in our solar system and can be used to calculate the distance and orbital period of other planets if you know the distance between the Earth and the Sun (1 AU) and that one Earth year is 365 days. For example, Mercury, the closest planet to the Sun, completes an orbit every 88 days, whereas Saturn, the sixth planet from the Sun, takes 10,759 days.
The equation for Kepler's Third Law is P^2 = a^3, where P is the period of a planet's orbit and a is the size of the semi-major axis of the orbit in astronomical units. This law allows us to calculate the masses of planets in our solar system, as well as the masses of artificial satellites placed in orbit around them.
Kepler's Third Law can also be applied to exoplanets, or planets beyond our solar system. To do this, the formula is modified to account for the variation in the star's mass compared to the Sun.
Kepler's laws of planetary motion were formulated by German mathematician and astronomer Johannes Kepler in the early 17th century. Before Kepler's laws, the motion of the planets around the Sun was not well understood, and the Earth was believed to be the centre of the solar system. Kepler's laws shifted the Sun from the centre of the model to a focal point and suggested that planetary bodies move at speeds that vary depending on their proximity to the star.
Leash Laws: Do Cats Need to Follow Them?
You may want to see also

The law applies beyond our solar system
Kepler's Third Law, or the Law of Harmony, is not limited in its application to our solar system. It can be applied to any planetary system, no matter how different it is from our own. This includes systems with binary stars, which German astronomer Johannes Kepler could barely have dreamt of when he formulated his laws in the 16th century.
Kepler's Third Law states that the square of the orbital period of a planet is proportional to the cube of the semi-major axis of its orbit. This means that if we know a planet's distance from its star, we can calculate the period of its orbit, and vice versa. This law helps us understand the mechanics of planetary systems and has been vital in investigating binary star systems.
To apply Kepler's Third Law to exoplanets, the formula is modified to account for the variation in the star's mass compared to that of our Sun. Astronomers use the formula R = (T^2 x Ms)^(1/3), where Ms is the star's mass relative to the mass of our Sun, to calculate the mass of an exoplanet. This adjustment allows astronomers to calculate the orbits and masses of exoplanets beyond our solar system.
Kepler's laws have also enabled us to derive the masses of stars in binary systems, which is crucial for understanding the structure and evolution of stars. By using the binary mass function, which is derived from Kepler's third law and the fact that bodies orbit a mutual centre of gravity, astronomers can calculate the sum of the masses of the two stars in a binary system.
In addition to its applications in astronomy, Kepler's Third Law has been used in the field of physics. When combined with Newton's laws of motion and law of gravity, Kepler's Third Law takes on a more general form that can be used to find the masses of the bodies involved in a system. This generalised form of the equation has been used to calculate the masses of planets, moons, and artificial satellites in our solar system.
HIPAA Laws: Do They Apply to Businesses?
You may want to see also
Frequently asked questions
Kepler's Third Law states that the square of a planet's orbital period is proportional to the cube of the length of the semi-major axis of its orbit.
No. Kepler's Third Law can be used to calculate the orbits and masses of exoplanets.
Kepler's Third Law has been vital in investigating binary star systems and has been used to calculate the masses of stars in these systems.
Kepler's laws of planetary motion were a significant advancement in our understanding of the solar system. They replaced circular orbits in the heliocentric theory with elliptical orbits and explained how planetary velocities vary.







