Compound interest is a powerful tool in finance that can be leveraged to increase savings and investments or make borrowing more expensive. It is the interest calculated on an account's principal and any accumulated interest, resulting in exponential growth. This phenomenon is often referred to as interest on interest and can be applied to various financial instruments, including savings accounts, investments, loans, and credit card debt.
The law of compound interest, or the miracle of compounding, as some call it, is a crucial concept in personal finance. It can significantly impact an individual's financial plans, either as an investor or a borrower. Understanding how compound interest works is essential for building wealth and minimising debt.
| Characteristics | Values |
|---|---|
| Definition | Interest that applies not only to the initial principal of an investment or loan but also to the accumulated interest from previous periods |
| Interest Calculation | Interest calculated on both the initial principal and all previously accumulated interest |
| Interest on Interest | Interest earned on interest |
| Interest Rate | Annual interest rate |
| Number of Compounding Periods | The higher the number of compounding periods, the larger the effect of compounding |
| Compounding Frequency | Interest can be compounded daily, monthly, quarterly, annually, or at any other frequency |
| Comparison with Simple Interest | Compound interest results in higher returns than simple interest |
| Savings | Compound interest can help savings grow faster |
| Borrowing | Compound interest can make borrowing more expensive |
| Debt | Compound interest can increase the amount of debt over time |
| Investments | Compound interest can boost investment returns |
What You'll Learn

How to calculate compound interest
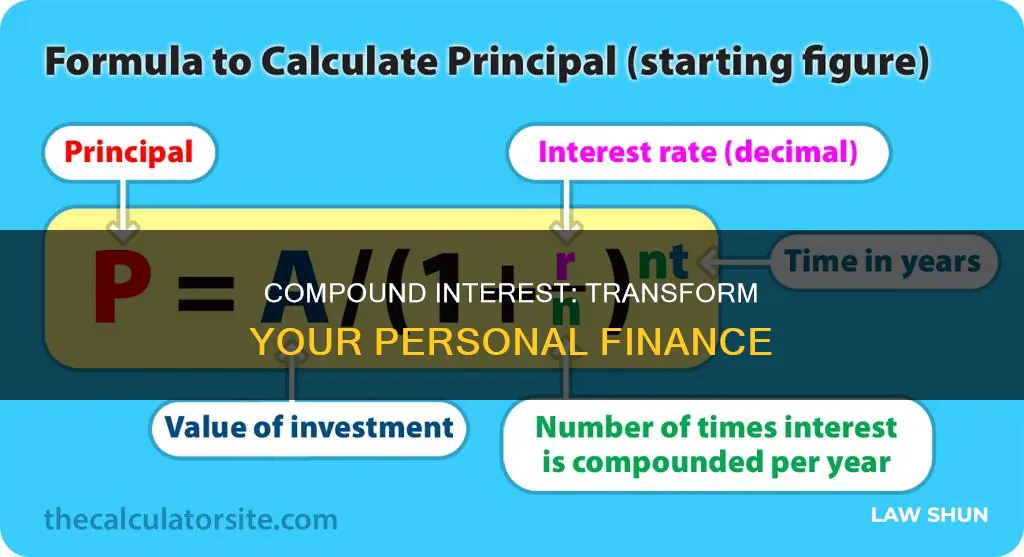
The formula for calculating compound interest is:
Compound interest = total amount of principal and interest in the future (or future value) – principal amount at present (or present value)
= [P (1 + i)n] – P
= P [(1 + i)n – 1]
Where:
- I = annual interest rate
- N = number of compounding periods
- P = principal amount
For example, take a 3-year loan of $10,000 at an interest rate of 5%, compounding annually. The amount of interest would be:
$10,000 [(1 + 0.05)³ – 1] = $10,000 [1.157625 – 1] = $1,576.25
You can also calculate compound interest using a calculator or a spreadsheet.
Using a Calculator
Input any 4 values of principal, interest, rate, time, or compounding frequency and calculate the missing value. Remember to input time in terms of years. So, if your time value is in months, just divide it by 12 to get the equivalent value in years.
Using a Spreadsheet
You can use Microsoft Excel to calculate compound interest in three different ways:
Multiplication
- Suppose you deposit $1,000 into a savings account with a 5% interest rate that compounds annually, and you want to calculate the balance in 5 years.
- In Microsoft Excel, enter "Year" into cell A1 and "Balance" into cell B1.
- Enter years 0 to 5 into cells A2 through A7.
- The balance for year 0 is $1,000, so enter “1000” into cell B2.
- Next, enter “=B21.05” into cell B3.
- Then enter “=B31.05” into cell B4 and continue to do this until you get to cell B7.
- In cell B7, the calculation is “=B61.05”.
- Finally, the calculated value in cell B7—$1,276.28—is the balance in your savings account after five years.
- To find the compound interest value, subtract $1,000 from $1,276.28; this gives you a value of $276.28.
Fixed Formula
- The compound interest formula is ((P(1+i)^n) - P), where P is the principal, i is the annual interest rate, and n is the number of periods.
- Using the same example as above, enter “Principal value” into cell A1 and “1000” into cell B1.
- Next, enter “Interest rate” into cell A2 and “.05” into cell B2.
- Enter “Compound periods” into cell A3 and “5” into cell B3.
- Now you can calculate the compound interest in cell B4 by entering “=(B1(1+B2)^B3)-B1”, which gives you $276.28.
Macro Function
- First, start the Visual Basic Editor, which is located in the developer tab.
- Click the Insert menu, and click on "Module."
- Then type "Function Compound_Interest (P As Double, I As Double, N As Double) As Double" in the first line.
- On the second line, hit the tab key and type in "Compound_Interest = (P(1+i)^n) - P."
- On the third line of the module, enter “End Function.”
- You have now created a function macro to calculate the compound interest rate.
- Continuing from the same Excel worksheet above, enter “Compound interest” into cell A6 and enter “=Compound_Interest(B1, B2, B3).” This gives you a value of $276.28, which is consistent with the first two values.
Unclaimed Property Laws: Do Massachusetts Non-Profits Comply?
You may want to see also

Compound interest on savings
The law of compound interest certainly applies to personal finance, and it can be a powerful tool for growing your savings over time.
Compound interest is essentially earning interest on your interest. It is the result of reinvesting the interest you've earned back into the principal amount, so that the interest itself starts generating interest. This leads to exponential growth in your savings over time.
For example, let's say you invest $1,000 in a savings account with an annual interest rate of 8%. After the first year, your balance will be $1,080. In the second year, you contribute another $1,000, and you earn 8% on the total balance, which now includes the interest from the first year. This means your balance at the end of the second year will be $2,080 plus 8% interest, giving you a total of $2,246.40.
The effect of compound interest becomes more significant over time. While it may seem like you're earning a modest amount of interest in the early years, as time goes on, your compounding interest will grow at an accelerating pace and become the primary driver of growth in your account.
The key to maximising compound interest is to start saving early and consistently. The longer your money remains in a compound interest account, the more it will grow. Additionally, the more frequently interest is compounded, the greater the effect. Interest on savings accounts can be compounded daily, monthly, quarterly, or annually, with daily compounding being the most common.
Here's an example to illustrate the power of compound interest over time. Let's say you start saving $100 a month at age 20, earning an average of 4% annually, compounded monthly, until you reach age 65. By the time you're 65, you would have earned $151,550 in interest, with a total savings of $205,650. On the other hand, if you waited until age 50 to start saving, investing $5,000 initially and then $500 a month for 15 years at the same interest rate, you would only earn $132,147 in interest, with a total savings of $187,147.
Compound interest can be a powerful tool for growing your savings over time, but it's important to remember that it can also work against you when it comes to debt. If you're paying compound interest on loans or credit card debt, the amount you owe can grow exponentially if you're only making minimum payments.
Price Gouging Laws: Private Sales Exempt?
You may want to see also

Compound interest on debt
Compound interest is a double-edged sword. While it can help investors build wealth, it can also be a burden for borrowers, who can find themselves in a "debt cycle".
How Compound Interest Works on Debt
Compound interest is interest that is calculated not just on the principal amount but also on the interest accumulated from previous periods. This is often referred to as "interest on interest". The more frequently interest is compounded, the larger the effect of compounding. Interest can be compounded daily, monthly, quarterly, or annually.
Here's an example of how compound interest can work against you:
Let's say you have a credit card balance of $6,194 (the average credit card debt in the US). If you only make the minimum payment each month, the interest will compound and quickly add up. At the average credit card APR of 16.61%, after 10 years, you will have paid $10,657 in interest alone—more than your initial balance.
How to Avoid the Pitfalls of Compound Interest
- Be discerning about debt. Only take on debt that you can afford to pay back, at an interest rate that won't hinder your ability to save.
- Pay down high-interest debts. If you have high-interest debt, consider refinancing to a lower rate. Make it a priority to pay off your credit card balance in full each month to avoid interest charges.
Kepler's Laws: Do Artificial Objects Obey the Same Rules?
You may want to see also

How to avoid paying compound interest
Compound interest is a powerful tool that can help your savings grow faster, but it can also make borrowing more expensive. It is calculated on an account's principal plus any accumulated interest. This means that you can end up paying interest on your interest, which can make it challenging to pay off your debt.
- Pay off your loans as quickly as possible: The faster you pay off your loan, the less interest you will accrue. Consider making more frequent payments, such as fortnightly instead of monthly. This strategy can help you pay off your loan sooner and reduce the total amount of interest you pay.
- Refinance when interest rates are declining: Keep an eye on interest rates and consider refinancing your loan when rates are on a downward trend. This can help you secure a lower interest rate and reduce the overall cost of your loan.
- Use an offset account facility: If your bank offers an offset account facility, take advantage of it. This can help you minimise the compound interest on your loan.
- Choose loans that charge simple interest: Simple interest is calculated only on the principal amount, not on accumulated interest. Many large loans, such as mortgages and car loans, use a simple interest formula.
- Pay off your credit card balances in full each month: Credit cards often use compound interest, so be sure to pay off your statement balance in full each month to avoid paying additional interest.
- Check for fees on additional payments: Before making extra payments towards your loan, check with your bank to see if there are any associated fees or penalties. For example, partially or fully fixed mortgages may have limits on how much extra you can pay off each year without incurring a penalty.
Good Samaritan Laws: Residence Life's Legal Shield?
You may want to see also

How compound interest affects financial planning
Compound interest is a powerful tool in financial planning, and understanding how it works is crucial for effective money management. Compound interest is the interest calculated not only on the initial amount of money (the principal) but also on the accumulated interest from previous periods. In other words, it involves earning or owing interest on interest, leading to exponential growth or loss over time.
When it comes to financial planning, compound interest can be a double-edged sword. On the one hand, it can significantly accelerate the growth of your savings and investments. For example, consider a savings account with a 5% annual interest rate. In the first year, you earn $50 on a $1,000 deposit. However, in the second year, you earn interest on the new balance of $1,050, resulting in $52.50 in interest. This process repeats, and the growth of your savings account balance accelerates over time.
On the other hand, compound interest can also work against you when it comes to debt. Credit card debt, for instance, compounds, and this is why it can quickly become overwhelming. If you don't pay off the interest each month, it gets added to your credit card balance, and the following month's interest is calculated on this new, higher amount. This cycle continues, causing your balance to skyrocket.
The key factors that influence compound interest are the interest rate, the starting principal, the frequency of compounding (daily, monthly, annually), the duration of the investment or loan, and any deposits or withdrawals made over time. The more frequently interest is compounded, the more significant the effect, whether it is working for or against you.
When planning your finances, it is essential to consider the impact of compound interest. It can help you make informed decisions about saving, investing, and borrowing. Starting early and being consistent with your savings or investments maximizes the benefits of compound interest, allowing your money to grow faster. Conversely, paying down debt aggressively can help prevent compound interest from increasing your debt burden.
Additionally, when comparing financial products, it is crucial to look beyond the annual percentage rate (APR) and consider the annual percentage yield (APY), which accounts for compounding. The more frequently an account compounds interest, the more you'll earn on your savings and investments, and the more you'll owe on your debts.
Understanding Missouri's Homestead Laws: Renters' Rights Explained
You may want to see also
Frequently asked questions
Compound interest is a type of interest that is calculated not only on the initial principal of an investment or loan but also on the interest accumulated from previous periods. In other words, it involves earning or owing interest on interest.
Compound interest can work for or against you in personal finance, depending on whether you are an investor or a borrower. As an investor, compound interest can help your savings grow faster. On the other hand, as a borrower, compound interest can make borrowing more expensive and increase the amount of money you owe over time.
To benefit from compound interest, consider saving or investing in accounts that offer compounding interest. Choose accounts with higher interest rates and more frequent compounding periods to maximise your returns. Additionally, avoid making withdrawals to allow your interest to accumulate and grow exponentially over time.







