Applying Kepler's Third Law: Understanding Orbital Periods And Distances

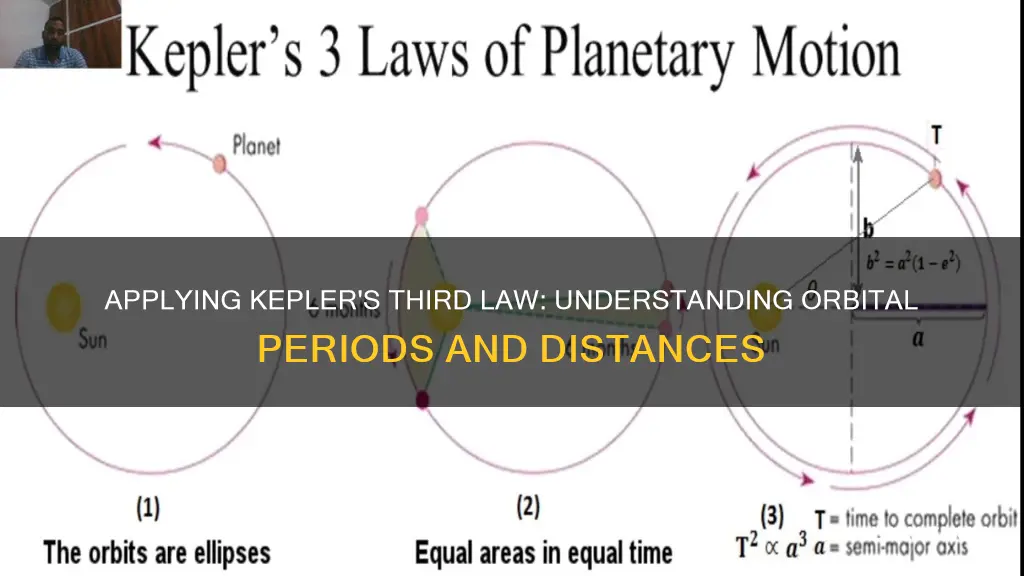
Kepler's Third Law, also known as The Law of Harmony, explains planetary orbits around the sun. Before German mathematician and astronomer Johannes Kepler outlined his laws of planetary motion in the early 17th century, humankind’s knowledge of the solar system was in its infancy. Kepler's Third Law states that the square of the orbital period of a planet is proportional to the cube of the semi-major axis of its orbit. In other words, a planet’s orbital period is proportional to the size of its orbit. This means that as a planet’s distance from the sun increases, the time it takes to orbit the sun increases rapidly. For example, Mercury, the closest planet to the sun, completes an orbit every 88 days, while Saturn, the sixth planet from the sun, takes 10,759 days. Kepler's Third Law can be applied to calculate the masses of planets in our solar system, and it has been vital in investigating binary star systems and discovering planets outside the solar system.

Calculating the mass of a distant star

Kepler's Third Law can be used to calculate the mass of a distant star. This is done by measuring the period of the orbit of a planet orbiting the star in days and the semi-major axis of the orbit in astronomical units (AU).

The semi-major axis of an orbit is the longest axis of an ellipse, which is the shape that planetary orbits form. Half of the major axis is the semi-major axis.

The formula for Kepler's Third Law is:

> P^2 = a^3

Where:

• P = the period of a planet's orbit
• A = the size of the semi-major axis of the orbit

By measuring the period and semi-major axis, the total mass of the system (star and planet) can be determined in units of solar mass.

However, the mass of the star itself cannot be calculated directly from this method, as the constant in Kepler's Third Law depends on the total mass of the two bodies involved. To calculate the mass of the star, the mass of the planet would need to be subtracted from the total mass of the system.

For example, if the total mass of a system is calculated to be 2 solar masses and the mass of the planet is 0.5 solar masses, then the mass of the star would be 1.5 solar masses.

It is important to note that this method assumes that the orbit of the planet is elliptical, which is a result of Kepler's First and Second Laws.

Determining the mass of the Sun

Kepler's Third Law, or The Law of Harmony, states that "the square of the orbital period of a planet is proportional to the cube of the semi-major axis of its orbit". This law allows us to determine the mass of the Sun using data for the Earth.

The first step is to write Kepler's Third Law as:

> a^3/T^2 = G(M + m)/4π^2 = constant

Where:

• A is the semi-major axis of the orbit
• T is the orbital period
• G is the gravitational constant
• M is the star's mass
• M is the planet's mass

Next, we multiply the equation by 4π^2/G:

> a^3/T^2 * 4π^2/G = (M + m)

Then, we insert the values for Earth's orbit:

• T (Earth) = 1 year
• A (Earth) = 1 astronomical unit (AU)

This gives us:

> (1 AU)^3/ (1 year)^2 * 4π^2/G = M + m

Now, we can solve for M (the mass of the Sun) by subtracting the mass of the Earth (m) from the above equation:

> M = ( (1 AU)^3/ (1 year)^2 * 4π^2/G) - m

The mass of the Sun is approximately 2 x 10^30 kg.

This calculation assumes that the orbit of the Earth around the Sun is a perfect circle. However, as Kepler discovered, the orbits of planets are not circular but elliptical. Therefore, this calculation provides an approximation for the mass of the Sun.

Applying the law to planets beyond the solar system

Kepler's Third Law can be applied to planets beyond our solar system. Over 4,000 exoplanets have been discovered, and thanks to Kepler's laws, astronomers can calculate their orbits and masses.

The formula for exoplanets is modified to account for the variation in the star's mass compared to the Sun. The formula used is R = (T^2 x Ms)^(1/3), where Ms is the star's mass relative to the Sun's mass. This allows astronomers to calculate the mass of an exoplanet.

Kepler's Third Law, in combination with his Second Law, has enabled scientists to derive the masses of stars in binary systems. This is vital for understanding the structure and evolution of stars. To do this, astronomers use the binary mass function, which is derived from Kepler's Third Law and the fact that bodies orbit a mutual centre of gravity.

Kepler's Third Law can also be applied to planets and their moons, as well as artificial satellites placed in orbit around them. For example, the recent placement of artificial satellites around Venus has enabled scientists to accurately calculate the mass and mean density of the planet.

Using the law to launch satellites

Kepler's Third Law can be used to launch satellites by calculating the mass of the body the satellite is orbiting. This is done by measuring the period of the orbit and the semi-major axis of the orbit in astronomical units.

The equation for Kepler's Third Law is P^2 = a^3, where P is the period of the orbit and a is the semi-major axis of the orbit. This equation can be rearranged to solve for the mass of the body being orbited, which is useful when launching satellites into orbit around a planet or moon.

For example, to launch a satellite into orbit around Earth, you would measure the period of the satellite's orbit and the semi-major axis of its orbit in astronomical units. Then, using Kepler's Third Law, you can calculate the mass of the Earth. This information can be used to inform the satellite's launch trajectory and speed.

Similarly, if you want to launch a satellite into orbit around another planet, such as Mars, you would use Kepler's Third Law to calculate the mass of Mars. This calculation would be based on measurements of the period and semi-major axis of a satellite orbiting Mars.

It is important to note that Kepler's Third Law assumes that one body is much more massive than the other, which is typically true for a satellite orbiting a planet or moon. However, when dealing with binary star systems or planets with significant moons, the masses of both bodies may need to be considered.

In summary, Kepler's Third Law can be applied to launch satellites by providing a way to calculate the mass of the body being orbited. This calculation, along with other orbital measurements, helps inform the satellite's launch trajectory and speed.

Calculating the mass of a parent body from the orbits of its satellites

Kepler's Third Law can be used to calculate the mass of a distant star or planet by measuring the period of its orbit and the semimajor axis of the orbit. The formula for this is:

> M = 4π^2r^3/GT^2

Where M is the mass, r is the orbital radius, T is the orbital period, and G is the universal gravitational constant.

This formula can be used to calculate the mass of a star or planet with a circular orbit. For example, if a planet is discovered orbiting a distant star with a period of 105 days and a radius of 0.480 AU, the formula can be used to calculate the mass of the star.

It is important to note that the accuracy of the mass calculation depends on the precision of the measurements. Small errors in the measurements of the orbital period, apparent angular size of the orbit, and distance to the star can affect the calculated mass and introduce uncertainty.

Additionally, Kepler's Third Law assumes that one central mass is much larger than the other, such as in the case of a planet orbiting a star. In reality, the formula should account for the sum of the masses of the two objects, as represented by the equation:

> M1 + M2 = 4π^2a^3/(GP^2)

Where M1 and M2 are the masses of the two objects, a is the semi-major axis, and P is the orbital period. This formula recognises that the mass of the orbiting body cannot be ignored when calculating the total mass of the system.

Kepler's Third Law has been successfully applied to calculate the masses of planets in our solar system, as well as artificial satellites placed in orbit around them. It has also been used to determine the mass of the Pluto-Charon system and to study the orbits of comets around the Sun.

Frequently asked questions