Stokes' Law: Understanding Marine Biology With Physics

Stokes' Law is a mathematical equation that explains the drag force experienced by small spherical particles passing through a viscous fluid medium. It was derived by George Gabriel Stokes in 1851 and is used to determine the terminal velocity of an object. The law assumes that the drag force F acting upward in resistance to the fall is equal to 6πrηv, where r is the radius of the sphere, η is the viscosity of the liquid, and v is the velocity of fall. Stokes' Law is important for understanding the swimming of microorganisms and sperm, as well as the sedimentation of small particles and organisms in water, under the force of gravity.

Raindrop velocity

When a raindrop falls from a great height, it accelerates due to gravity. However, as its velocity increases, a counterforce called viscous drag also increases, acting against the raindrop's descent. This viscous drag force is dependent on the radius of the raindrop, the viscosity of the air, and the raindrop's velocity. As the raindrop continues to fall, a point is reached where the force of gravity and the viscous drag force are equal. At this moment, the raindrop's acceleration ceases, and it falls with a constant velocity known as the terminal velocity.

The terminal velocity of a raindrop is crucial in understanding why raindrops do not harm us. Due to their small size, raindrops have a low terminal velocity, preventing them from reaching extremely high speeds during their free fall. As a result, when raindrops hit our skin, we do not experience significant pain or injury.

Stokes' Law also sheds light on the phenomenon of cloud formation. Small water droplets, similar in size to raindrops, have low terminal velocities, causing them to remain suspended in the upper atmosphere for extended periods. These suspended droplets form clouds. As the droplets combine and increase in size, their terminal velocity also increases, eventually becoming heavy enough to fall as rain.

Furthermore, Stokes' Law helps explain why larger raindrops tend to hurt more when they hit us. Larger raindrops have a larger terminal velocity, and when they make contact with our skin, the impact is more pronounced, resulting in a greater sensation of pain.

In summary, Stokes' Law provides valuable insights into raindrop velocity, cloud formation, and the varying impact of raindrops on our bodies. By considering the forces acting on raindrops as they fall through the air, we can comprehend the underlying principles that govern their behaviour and velocity.

Terminal velocity

Stokes' Law is an empirical law in fluid dynamics that describes the frictional force, or drag force, exerted on spherical objects with very small Reynolds numbers in a viscous fluid. It was derived by George Gabriel Stokes in 1851 and has found applications in several areas, including the settling of sediment in freshwater and measurements of the viscosity of fluids.

The law assumes laminar flow in homogeneous fluids and that the particle is smooth, spherical, and not interacting with other particles. Stokes' Law states that the viscous force on a solid body moving through a fluid depends on the speed of the body, the coefficient of viscosity of the fluid, and the shape and size of the body. The drag force F acting on the sphere is given by the equation:

> F = 6πrηv

Where r is the radius of the sphere, η is the viscosity of the fluid, and v is the velocity of the sphere.

At terminal velocity, the excess force Fe due to the difference between the weight and buoyancy of the sphere is given by:

> Fe = (ρp - ρf) * g * (4/3)πR^3

Where ρp is the mass density of the sphere, ρf is the mass density of the fluid, g is the gravitational acceleration, and R is the radius of the sphere.

Equating the drag force Fd and the excess force Fe, and solving for velocity v, gives the terminal velocity vs. This velocity is reached when the sum of the frictional and buoyant forces on the particle due to the fluid exactly balances the gravitational force.

The terminal velocity is directly proportional to the square of the radius of the sphere and inversely proportional to the viscosity of the fluid. This means that a larger sphere will have a greater terminal velocity than a smaller sphere of the same material.

Stokes' Law can be used to investigate sediments in water, determine the viscosity of fluids, and analyze rain clouds and fog. It also helps explain why large raindrops fall faster and have a greater impact when they hit a surface compared to smaller ones.

Cloud formation

Stokes' Law is a mathematical equation that explains the drag force experienced by small spherical particles passing through a viscous fluid medium. It was first proposed by the Anglo-Irish physicist and mathematician George Gabriel Stokes in 1851.

Stokes' Law can be used to explain the formation and floating of clouds. Raindrops are smaller in size, so their terminal velocities are small, and they remain suspended in the air in the form of clouds. As they increase in size, their terminal velocities also increase, and they begin to fall in the form of rain.

The upward drag force on a spherical body falling through a column of liquid will eventually equal the gravitational force, and the body will fall at a constant velocity. The force that slows a sphere moving through a viscous fluid is directly proportional to the velocity and radius of the sphere, as well as the viscosity of the fluid.

The viscosity of the fluid, the radius of the particle, and the velocity of the particle relative to the fluid all play a role in Stokes' Law, which gives the drag force acting on a spherical particle as it passes through a liquid under the influence of gravity.

The drag force F acting upward in resistance to the fall is equal to 6πrηv, where r is the radius of the sphere, η is the viscosity of the liquid, and v is the velocity of the fall.

The force acting downward is equal to 4/3πr3 (d1 − d2)g, where d1 is the density of the sphere, d2 is the density of the liquid, and g is the acceleration due to gravity.

At a constant velocity, called the terminal velocity, the upward and downward forces are balanced. Equating the two expressions given above and solving for v yields the required velocity:

V = 2/9(d1 − d2)gr2/η

Stokes' Law is valid only when the velocity of the object remains constant under the influence of a gravitational force that is counterbalanced by the drag force of the liquid.

Parachutes

When applied to parachutes, Stokes' Law explains the phenomenon of air resistance or drag, which acts against the motion of an object falling through a fluid, in this case, air. As the parachute falls, it experiences a drag force that increases as its velocity increases. Eventually, this drag force balances out the force of gravity, causing the parachute to reach a constant velocity known as the terminal velocity.

The size and shape of the parachute, air density, and velocity all influence the drag force acting on a parachute. Additionally, factors such as wind speed and direction, weight, and design can also impact the descent of a parachute.

Stokes' Law is crucial in understanding the mechanics of parachutes. It explains why parachutes are designed with a large surface area, as a larger surface area increases the drag force, helping to slow down the object's descent. This understanding of air resistance and the role of surface area in parachutes is essential for their design and function.

In summary, Stokes' Law provides valuable insights into how parachutes work, helping to ensure safe landings by controlling the descent of objects through the manipulation of air resistance and surface area.

Sedimentation

Stokes' Law is a mathematical equation that expresses the drag force resisting the fall of small spherical particles through a fluid medium. It was derived by George Gabriel Stokes in 1851 and is particularly useful for understanding the sedimentation of small particles and organisms in water.

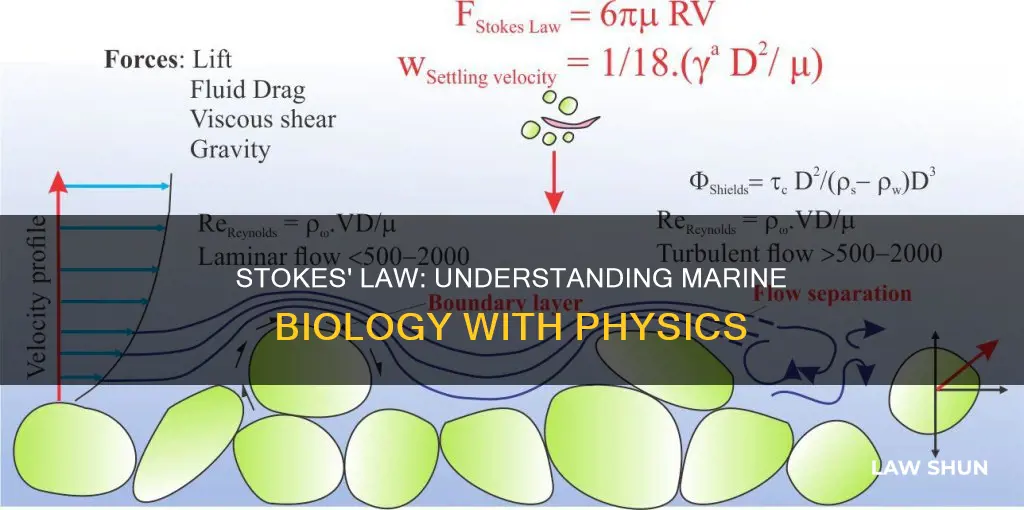
The settling velocity (ws) of a particle is reached when its submerged weight equals the drag force acting on it. This occurs when the particle reaches a constant speed, known as the terminal velocity. Stokes' Law expresses the fluid drag (Fd) as:

Fd = 6πμRV

Where:

• Fd is the frictional force, or drag force, acting on the interface between the fluid and the particle
• Μ is the dynamic viscosity of the fluid
• R is the radius of the spherical object
• V is the flow velocity relative to the object

The submerged weight of the particle can be calculated as:

Submerged weight = Weight of particle – Buoyancy force

The buoyancy force is equal to the weight of the displaced fluid, which is the volume of the spherical particle multiplied by the density of the fluid. Therefore, the equality can be written as:

1/6)πD3γ = 3πμVD

Where:

• D is the diameter of the particle
• Γ is the submerged weight per unit volume, calculated as: γ = (ρs – ρw)g, where ρs is the density of the solid grains and ρw is the density of water

Solving for the settling velocity, we get:

Ws = 1/18. (γ D2/ μ)

Or:

Ws = 1/18. (ρs – ρw)g D2 /μ

Stokes' Law is based on several assumptions, including that the particles are spherical, smooth, and not interacting with each other, and that the fluid exhibits laminar flow. These assumptions can be violated in real-world scenarios, such as during hydraulic fracture treatments, leading to deviations from the predicted settling velocities. However, despite these limitations, Stokes' Law remains a valuable tool for understanding and predicting sedimentation in marine environments.

Frequently asked questions