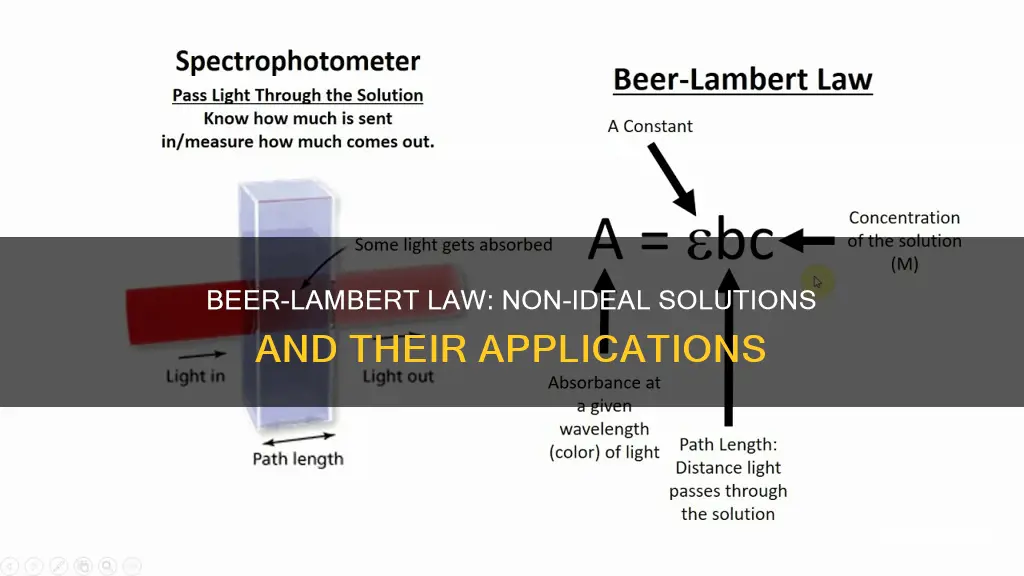
The Beer-Lambert law, or Beer's law, is a fundamental principle in physics and chemistry, describing the relationship between the absorption of light and the properties of a material. It states that the absorption of light is directly proportional to the concentration of the absorbing species in a solution, the path length of light through the solution, and the molar absorptivity of the species. However, this law has limitations and does not hold true for all solutions, especially at high and low concentrations.
Despite these deviations, the Beer law still finds wide application in modern science, including medicine testing, organic chemistry, and quantification. This paragraph will explore why the Beer law remains applicable and relevant even for non-ideal solutions, delving into the underlying principles, assumptions, and its utility in various fields.
| Characteristics | Values |
|---|---|
| Relationship | The Beer-Lambert Law states the relationship between the attenuation of light through a substance and the properties of that substance. |
| Absorbance | The absorbance of a transition depends on two external assumptions. |
| Concentration | The Beer-Lambert Law states that there is a linear relationship between the concentration and the absorbance of the solution. |
| Light Path Length | The Beer-Lambert Law states that there is a linear relationship between the light path length and the absorbance of the solution. |
| Molar Absorptivity | The larger the molar absorptivity, the more probable the electronic transition. |
What You'll Learn
- The Beer-Lambert law states that the absorbance of light is directly proportional to the concentration of the absorbing species
- The law is used in modern-day labs for testing medicines, organic chemistry and tests with quantification
- The law is not obeyed at high and low concentrations of a particular metallic solution
- The law is also not obeyed when the analyte molecules interact with each other
- The law assumes that the incident radiation is monochromatic

The Beer-Lambert law states that the absorbance of light is directly proportional to the concentration of the absorbing species
The Beer-Lambert law is derived from two separate laws: Beer's Law and Lambert's Law. Beer's Law, stated by August Beer, says that concentration and absorbance are directly proportional to each other. Meanwhile, Johann Heinrich Lambert's Lambert's Law states that absorbance and path length are directly proportional.
The Beer-Lambert law is expressed as:
> A is the amount of light absorbed for a particular wavelength by the sample
>
> ε is the molar extinction coefficient
>
> L is the distance covered by the light through the solution
>
> c is the concentration of the absorbing species
The Beer-Lambert law can be written as:
> \(\epsilon =\frac{A}{Lc}\)
The Beer-Lambert law is used to define the relationship between the intensity of visible UV radiation and the exact quantity of the substance present. It is used in modern-day labs for testing medicines, organic chemistry, and tests with quantification.
The Beer-Lambert law is not always obeyed, especially in high and low concentrations of a particular metallic solution. This is because the linearity of the law is limited to chemical and instrumental factors. When the solution has higher concentrations, the proximity between the molecules of the solution is so close that there are deviations in the absorptivity.
The Beer-Lambert law is also limited by the following:
- A diluted solution is used
- There is no scattering of the light beam
- Monochromatic electromagnetic radiation is used
Despite these limitations, the Beer-Lambert law is still valuable and applicable in many scientific fields.
Contract Law: Which Legal Framework Decides the Fate?
You may want to see also

The law is used in modern-day labs for testing medicines, organic chemistry and tests with quantification
The Beer-Lambert Law is used in modern-day labs for testing medicines, organic chemistry, and tests with quantification.
In medicine, the law is used to determine the concentration of a substance in a solution. For example, it can be used to determine the concentration of glycogen-iodine complex in a solution by measuring the transmission of light through it.
In organic chemistry, the law is used to determine the concentration of a solute in a solution by measuring the amount of light absorbed or transmitted by the solution. This is particularly useful for coloured solutions, as demonstrated by August Beer, who noticed that coloured solutions appeared to exhibit a similar attenuation relation.
The Beer-Lambert Law is also used in tests with quantification, such as spectroscopy, where it is used to determine the concentration of tissue chromophores and contrast agents, including oxy- and deoxy-hemoglobin.
Understanding Boiling Bubbles: Gas Laws in Action
You may want to see also

The law is not obeyed at high and low concentrations of a particular metallic solution
Beer-Lambert law, often referred to as Beer's law, states that the absorption of radiation is directly proportional to the concentration of the absorbing species. However, this law is not obeyed at high and low concentrations of a particular metallic solution.
At high concentrations, the absorbance is higher than 1, meaning that all light is absorbed. Under these conditions, the absorbance is not correct. Additionally, the analyte molecules may interact with each other, altering their ability to absorb radiation. This leads to a negative deviation from Beer's law.
At low concentrations, the amount of radiation absorbed may be undetectable, leading to significant errors in the absorbance measurement. This is because, at low concentrations, we are measuring a small difference between two large numbers, which can be prone to error.
Other factors that may cause deviations from Beer's law include the presence of stray radiation, the use of non-monochromatic radiation, and matrix effects in the sample, such as the presence of components that absorb radiation at the same wavelength as the analyte.
Labor Laws: Do They Extend to Military Personnel?
You may want to see also

The law is also not obeyed when the analyte molecules interact with each other
The Beer-Lambert law is derived from the assumption that the molecules of the analyte do not interact with each other. However, this assumption breaks down at high concentrations, where the analyte molecules are more likely to interact with each other. This effect leads to a negative deviation from Beer's Law.
The deviation occurs because the interaction between analyte molecules can alter their ability to absorb radiation. This is due to the changes in the polarizability of the molecules caused by chemical interactions, and the non-additive nature of attenuation cross-sections resulting from physical interactions.
Additionally, the deviation becomes more pronounced for strong oscillators at high concentrations. The deviation can also be influenced by the nature of the analyte solution, with homogeneity and the presence of scattering playing a role.
Step Therapy Law: Does It Affect Medicare?
You may want to see also

The law assumes that the incident radiation is monochromatic
The Beer-Lambert law is a combination of Beer's law and Lambert's law. It states that the concentration and absorbance are directly proportional to each other. The Beer-Lambert law is expressed as:
> A is the amount of light absorbed for a particular wavelength by the sample
>
> ε is the molar extinction coefficient
>
> L is the distance covered by the light through the solution
>
> c is the concentration of the absorbing species
The Beer-Lambert law assumes that the incident radiation is monochromatic. Monochromatic radiation refers to radiation that consists of a single colour or wavelength. In the case of monochromatic radiation, all transmitted photons have the same energy.
Spectroscopic instruments typically have a device known as a monochromator, which disperses radiation into distinct wavelengths. This is often achieved through the use of a prism. A slit is also used to block the wavelengths that are not of interest, allowing only the desired wavelength to pass through to the sample.
While the use of a monochromator helps to isolate a specific wavelength, it is not possible to obtain purely monochromatic radiation using this method. Nearby wavelengths of radiation will also pass through the slit, resulting in a packet of wavelengths centred on the desired wavelength. This packet of wavelengths is known as the effective bandwidth, and it depends on the slit width and the ability of the dispersing element to divide the wavelengths.
The use of a narrower slit can reduce the effective bandwidth, resulting in more monochromatic radiation and less deviation from linearity in the Beer-Lambert law. However, reducing the slit width also reduces the power of the radiation reaching the sample, which can lead to higher background noise and lower detection limits. Therefore, there is a trade-off between the desire for high source power and the desire for high monochromaticity.
Additionally, the sample may have slightly different molar absorptivities for each wavelength of radiation shining on it. As a result, the total absorbance added over all the different wavelengths may deviate from linearity with concentration. This deviation is more pronounced when there are greater differences in the molar absorptivities at different wavelengths.
In summary, while the Beer-Lambert law assumes monochromatic radiation, it is not possible to achieve purely monochromatic radiation in practice. The use of a monochromator and slit can help to isolate a specific wavelength, but there will always be some nearby wavelengths that pass through as well. The effective bandwidth can be reduced by narrowing the slit, but this also reduces the power of the radiation and can affect the detection limits. Deviations from linearity in the Beer-Lambert law can occur due to the polychromicity of the radiation, especially when there are significant differences in molar absorptivities at different wavelengths.
Equal Protection: Criminal and Civil Law
You may want to see also
Frequently asked questions
Beer's law fails at higher concentrations because the linearity of the law is limited to chemical and instrumental factors. When the solution has higher concentrations, the proximity between the molecules of the solution is so close that there are deviations in the absorptivity. Also, when the concentration is high, the refractive index changes.
The limitations of Beer's law are:
- A diluted solution is used
- There shouldn't be a scattering of the light beam
- Monochromatic electromagnetic radiation should be used
Beer's law states that concentration and absorbance are directly proportional to each other.