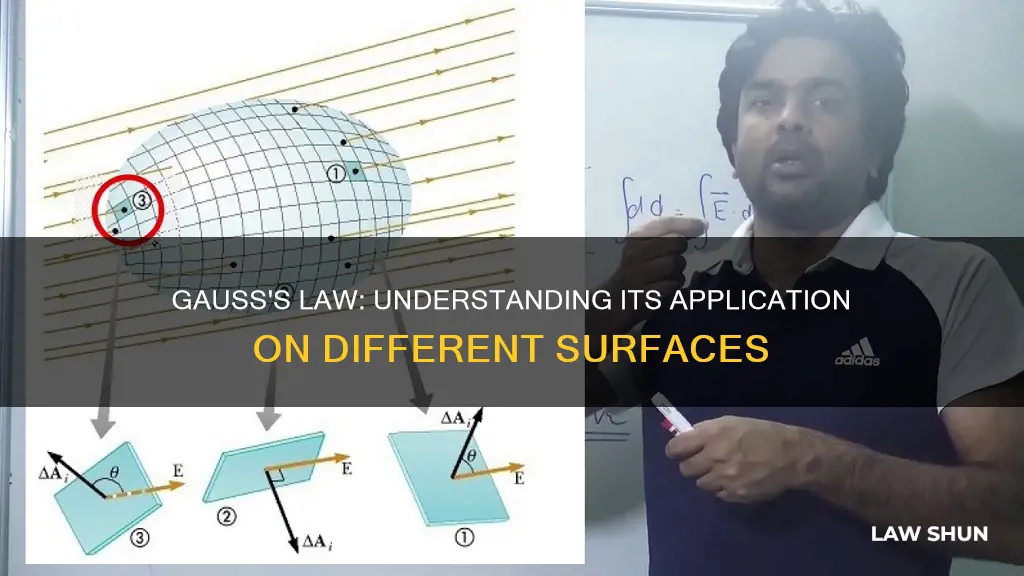
Gauss's law is a fundamental principle in physics, specifically electromagnetism, that relates the distribution of electric charges to the resulting electric field. It is applicable to any closed surface and is an integral part of Maxwell's equations, which form the basis of classical electrodynamics. The law states that the total electric flux out of a closed surface is directly proportional to the charge enclosed within that surface, irrespective of the distribution of the charge. This law simplifies the calculation of electric fields, especially in cases where symmetry is present, as it allows for the determination of the electric field without performing a surface integral. By choosing an appropriate Gaussian surface, where the electric field is either parallel or perpendicular to the surface, and the electric field passing through the surface has a constant magnitude, the calculation of the electric field becomes more manageable. Gauss's law is a versatile tool that can be applied to both uniform and non-uniform electric fields and is not limited to specific surface shapes or charge distributions.
| Characteristics | Values |
|---|---|
| Applicable to | Any closed surface |
| Electric flux | The electric field multiplied by the area of the surface projected in a plane perpendicular to the field |
| Charge enclosed | The total charge within the given surface |
| Permittivity | The electric constant |
What You'll Learn
- Gauss's Law applies to any closed surface
- It can be applied to both symmetric and asymmetric surfaces
- It can be used to determine the electric field of charge distributions with symmetry
- It relates the distribution of electric charge to the resulting electric field
- It can be used to derive Coulomb's law

Gauss's Law applies to any closed surface
The law states that the total electric flux out of a closed surface is equal to the charge enclosed divided by the permittivity. In other words, the net electric flux through any closed surface is equal to the net electric charge enclosed within that surface divided by the permittivity of free space.
Gauss's Law is a powerful tool for calculating electric fields, especially when the charge distribution exhibits certain spatial symmetries. These symmetries include spherical, cylindrical, and planar symmetries. By choosing a closed surface that aligns with the symmetry of the charge distribution, the calculation of the electric field can be simplified.
For example, in the case of a uniformly charged sphere, one can choose a spherical Gaussian surface. Due to the symmetry, the electric field generated will be radially symmetric, and the calculation of the flux integral becomes more straightforward.
It is important to note that Gauss's Law can also be applied to asymmetric surfaces. However, the calculation may become more tedious and involve more complex integrals.
Insider Trading Laws: Exempting Congress?
You may want to see also

It can be applied to both symmetric and asymmetric surfaces
Gauss's Law applies to any closed surface with some charge distribution. It is a general law that can be applied to both symmetric and asymmetric surfaces.
The law states that the total electric flux out of a closed surface is equal to the charge enclosed divided by the permittivity of free space. In other words, the net electric flux through any closed surface is equal to the net electric charge enclosed within that surface.
When there is sufficient symmetry, Gauss's Law simplifies the calculation of the electric field. Symmetric charge distributions allow for the removal of the electric field from the integral, making calculations easier. For example, in the case of a uniformly charged sphere, the electric field generated is radially symmetric. By taking the Gaussian surface to be a sphere of a certain radius, the normal to the sphere and the direction of the electric field coincide, simplifying the calculation.
However, Gauss's Law can also be applied to asymmetric surfaces. In cases where no symmetry exists, the law can be used in its differential form, which relates the divergence of the electric field to the local density of charge. This form is useful when the electric charge distribution is known, and the electric field must be computed.
The key advantage of Gauss's Law is that it allows for the evaluation of the electric field in many practical situations, regardless of the symmetry of the surface. It is a powerful tool that can be applied to a wide range of scenarios.
Understanding Applicable Laws for Municipal Contracts
You may want to see also

It can be used to determine the electric field of charge distributions with symmetry
Gauss's Law is a general law that applies to any closed surface. It states that the total electric flux out of a closed surface is equal to the charge enclosed, divided by the permittivity. The electric flux through an area is defined as the electric field multiplied by the area of the surface projected in a plane perpendicular to the field.
Gauss's Law is a powerful tool for calculating electric fields when they originate from charge distributions with sufficient symmetry. The three types of symmetry that allow Gauss's Law to be used to determine the electric field are:
- Spherical symmetry: The charge distribution depends only on the distance from a point in space and not on the direction.
- Cylindrical symmetry: The charge density depends only on the distance from the axis of a cylinder and must not vary along the axis or with direction about the axis.
- Planar symmetry: Charges are uniformly spread over a large flat surface. The electric field at a space point can only depend on the distance from the plane and has a direction either toward or away from the plane.
In these systems with symmetry, we can find a Gaussian surface over which the electric field has a constant magnitude. This allows us to choose a convenient surface that preserves the symmetry to remove the electric field from the integral. By doing so, we can determine the electric field of charge distributions with symmetry.
Lemon Law and Leased Vehicles: What You Need to Know
You may want to see also

It relates the distribution of electric charge to the resulting electric field
Gauss's Law, also known as Gauss's Flux Theorem, is a general law that applies to any closed surface. It relates the distribution of electric charge to the resulting electric field. In other words, it states that the net electric flux through any closed surface is equal to the net electric charge enclosed within that surface, multiplied by the permittivity of free space. Mathematically, this can be expressed as:
> ΦE = Electric flux through a closed surface S enclosing any volume V
> Q = Total charge enclosed within V
> ε0 = Electric constant
The electric flux ΦE is defined as a surface integral of the electric field, where E is the electric field, dA is a vector representing an infinitesimal element of the surface area, and the dot product represents the dot product of two vectors.
Gauss's Law is particularly useful when there is symmetry in the problem, which ensures that the electric field passes through the surface uniformly. In such cases, if the total flux is known, the electric field can be determined at every point. Examples of symmetries that lend themselves to Gauss's Law include cylindrical symmetry, planar symmetry, and spherical symmetry.
However, it is important to note that Gauss's Law alone is insufficient to determine the electric field across a surface enclosing any charge distribution. This is because the total flux through a given surface does not provide enough information about the electric field, which can have arbitrarily complicated patterns. Nonetheless, Gauss's Law is a powerful tool for calculating electric fields when they originate from charge distributions with sufficient symmetry.
Are Police Exempt from Maryland's AR-15 Laws?
You may want to see also

It can be used to derive Coulomb's law
Gauss's Law is a general law that applies to any closed surface. It relates the distribution of electric charge to the resulting electric field. In other words, it states that the net electric flux through any closed surface is equal to the net electric charge enclosed within that surface, multiplied by the inverse of the electric constant.
Gauss's Law can be used to derive Coulomb's Law, which describes the force between two static point electric charges. Coulomb's Law states that the force between two charges is proportional to the inverse square of the distance between them, acting in the direction of a line connecting them.
To derive Coulomb's Law from Gauss's Law, we can start by drawing a Gaussian sphere of radius 'r' with a charge '+q' located at its centre. Due to symmetry, the electric field 'E' has the same magnitude at every point on the surface of the sphere and is directed radially outward. This allows us to calculate the flux passing through an area element 'dS' on the Gaussian surface, which is given by 'dφ = E.dS'.
Using Gauss's Law, we can relate the flux passing through the entire Gaussian sphere to the charge enclosed within the closed surface. By comparing this equation with the equation for the flux through the area element 'dS', we can derive an expression for the magnitude of the intensity of the electric field 'E' at a point distant 'r' from the point charge '+q'.
By placing a second point charge 'q0' at the same location as the first charge, we can calculate the magnitude of the force acting on the second charge using the equation 'F = q0 * E'. Substituting the value of 'E' from the previous step, we obtain Coulomb's Law, which states that the force between two charges is directly proportional to the product of the charges and inversely proportional to the square of the distance between them.
Therefore, Gauss's Law can be used to derive Coulomb's Law by considering the electric field due to a point charge and applying the principles of symmetry and flux to calculate the resulting electric field and force between charges.
Intimidation and the Law: Where Does It Apply?
You may want to see also
Frequently asked questions
Yes, Gauss's Law applies to any closed surface and for any distribution of charges.
While Gauss's Law is applicable to any surface, symmetry can simplify the calculations. Symmetric charge distributions allow for the choice of a convenient surface that preserves the symmetry and removes the electric field from the integral.
Yes, Gauss's Law can be applied to both uniform and non-uniform electric fields.
A Gaussian surface is an imaginary closed surface constructed around a collection of charges to apply Gauss's Law. It is used to determine the electric field at that surface.
Yes, Gauss's Law can be used to find electric fields both within and outside charge distributions. The process is similar, but the Gaussian surface would be placed inside the distribution for internal fields.







