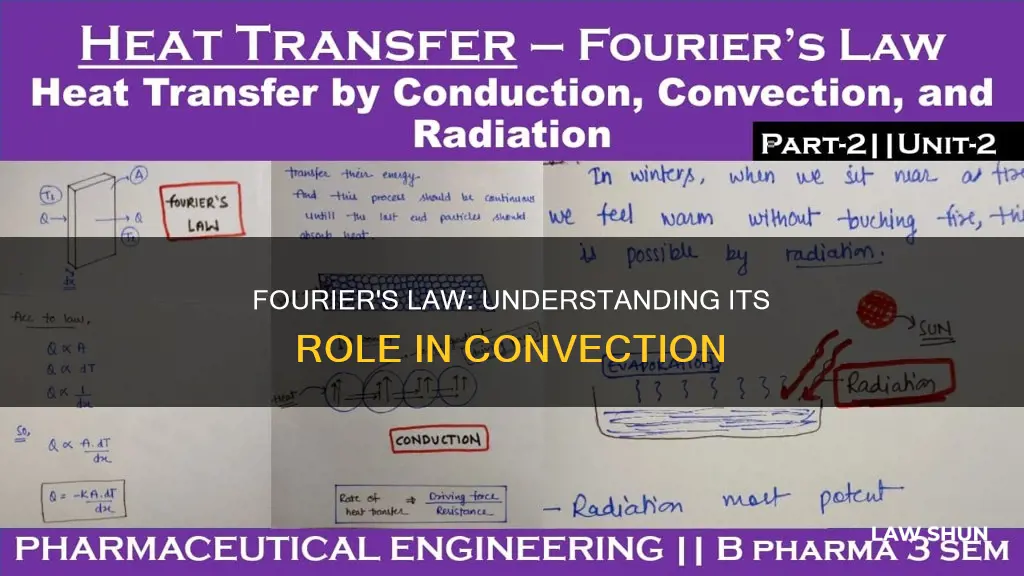
Fourier's Law, also known as the law of heat conduction, states that the rate of heat transfer through a material is proportional to the negative gradient in the temperature and to the area, at right angles to that gradient, through which the heat flows. In other words, the rate of heat conduction through a plane layer is proportional to the temperature gradient across the layer and the heat transfer area of the layer.
Fourier's Law can be written mathematically as:
q = −k∇T
Where:
- q is the local heat flux density in W.m2
- k is the thermal conductivity of the material in W.m-1.K-1
- ∇T is the temperature gradient in K.m-1
Fourier's Law applies to the transfer of heat through conduction, which is the transfer of heat through stationary matter by physical contact. Convection, on the other hand, is the transfer of heat by the macroscopic movement of a fluid.
| Characteristics | Values |
|---|---|
| What it applies to | Heat conduction |
| Law states | The rate of heat conduction through a plane layer is proportional to the temperature gradient across the layer and the heat transfer area of the layer |
| Mathematical form | q = -k∇T |
| q | Local heat flux density in W.m2 |
| k | Thermal conductivity in W.m-1.K-1 |
| ∇T | Temperature gradient in K.m-1 |
What You'll Learn

Fourier's Law and Convection
Fourier's Law, also known as the law of heat conduction, states that the rate of heat conduction through a plane layer is proportional to the temperature gradient across the layer and the heat transfer area of the layer. It is an empirical relation between the rate of heat conduction heat transfer and the temperature gradient in the direction of heat flow.
In mathematical form, Fourier's Law can be written as:
Q/A = -k(ΔT/L)
Where:
- Q is the rate of heat transfer
- A is the cross-sectional area of the layer
- ΔT is the temperature gradient, which is negative in the direction of heat transfer
- K is the thermal conductivity
- L is the thickness of the layer
The negative sign (-) is used to ensure that the rate of heat transfer is a positive quantity in the direction of heat transfer.
Heat transfer can occur through three methods: conduction, convection, and radiation. Fourier's Law applies to the first of these, conduction, which is heat transfer through stationary matter by physical contact. Convection, on the other hand, is the heat transfer by the macroscopic movement of a fluid.
In gases, heat transfer occurs through collisions of gas molecules with one another. In the absence of convection, thermal conduction through a gas phase is highly dependent on the composition and pressure of this phase, and particularly the mean free path of gas molecules relative to the size of the gas gap, as given by the Knudsen number.
In summary, Fourier's Law is a fundamental principle in physics that describes the relationship between the rate of heat conduction and the temperature gradient, specifically in the context of heat transfer through stationary matter via physical contact. It provides a quantitative framework for understanding and predicting heat conduction behaviour in various materials and systems.
Price Gouging Laws: Private Sales Exempt?
You may want to see also

Fourier's Law and Thermal Conduction
Fourier's Law, also known as the law of heat conduction or the law of thermal conductivity, is a principle of heat transfer. It states that the rate of heat conduction through a plane layer is proportional to the temperature gradient across the layer and the heat transfer area of the layer. In other words, the rate of heat transfer is directly related to the area at right angles to the gradient through which the heat flows.
Mathematically, Fourier's Law can be written as:
Q = -k∇T
Where:
- Q is the local heat flux density in W.m2
- K is the thermal conductivity of the material in W.m-1.K-1
- ∇T is the temperature gradient in K.m-1
Fourier's Law can also be written in one-dimensional form:
Qx = -k(dT/dx)
Where:
- Qx is the heat flux in the x direction
- K is the thermal conductivity
- DT/dx is the temperature gradient in the x direction
In three dimensions, Fourier's Law becomes:
Vecq = -k∇T
Where:
- Vecq is a vector representing the heat flux
- K is the thermal conductivity
- ∇T is the temperature gradient
Fourier's Law is an empirical relationship between the rate of heat transfer and the temperature gradient in the direction of heat flow. It is used to calculate the rate equation in thermal conduction. It is important to note that Fourier's Law assumes that heat transfer occurs only through conduction and does not account for other mechanisms such as convection or radiation.
Labor Laws: Nonprofit Organizations' Rights and Responsibilities
You may want to see also

Fourier's Law and Thermal Conductivity
Fourier's Law, also known as the law of heat conduction, describes how heat is transferred through a material. It states that the You may want to see also Fourier's Law is the governing law for heat conduction. It states that the rate of heat conduction through a plane layer is proportional to the temperature gradient across the layer and the heat transfer area of the layer. It is an empirical relation between the rate of heat conduction heat transfer and the temperature gradient in the direction of heat flow. The law refers to the principle of heat transfer where temperature gradients result in the faster dissipation of heat, influenced by the amount of latent heat stored internally in a material. The rate of heat conduction through a plane layer is proportional to the temperature gradient across the layer and the heat transfer area of the layer. The mathematical form of Fourier's Law is: Q = -k∇T Where: The three-dimensional form of Fourier's Law is: Vecq = -k∇T The first equality in the image provided in the source states that the rate of change of temperature in the direction of alpha-hat is the directional derivative. This is because the directional derivative of a scalar field is equal to the dot product of the gradient of the field and the direction in question. You may want to see also Fourier's law is the governing law for heat conduction. It states that the rate of heat conduction through a plane layer is proportional to the temperature gradient across the layer and the heat transfer area of the layer. It is an empirical relation between the rate of heat conduction heat transfer and the temperature gradient in the direction of heat flow. The law is contextual and selective in its operation. It is influenced by the amount of latent heat stored internally in a material. Fourier's law assumes two fundamental quantities: temperature and heat flow. The mathematical form of Fourier's law is: Q = -k∇T Where: Fourier's law can also be stated as: ΔQ/Δt = UA(-ΔT) Where: The three-dimensional form of Fourier's law is: Vecq = -k∇T Fourier's law is also called the law of heat conduction. You may want to see also Fourier's Law, also known as the law of heat conduction, states that the rate of heat transfer through a material is proportional to the negative gradient in temperature and the area at right angles to that gradient through which the heat flows. Fourier's Law can be written as: > q = −k∇T Where: - q is the local heat flux density (W/m^2) - k is the thermal conductivity of the material (W/(m·K)) - ∇T is the temperature gradient (K/m) Conduction is heat transfer through stationary matter by physical contact. Convection is the heat transfer by the macroscopic movement of a fluid. Heat transfer by radiation occurs when microwaves, infrared radiation, visible light, or another form of electromagnetic radiation is emitted or absorbed. Fourier's Law describes the rate of heat transfer through a material. This can be applied to any of the three methods of heat transfer: conduction, convection, and radiation.Employment Laws: Tribal Governments and Job Rights

Fourier's Law and Temperature Gradients
When Drugs Are Involved, Do Dram Shop Laws Apply?

Fourier's Law and Heat Transfer
Traffic Laws on Private Land: What's the Verdict?
Frequently asked questions







