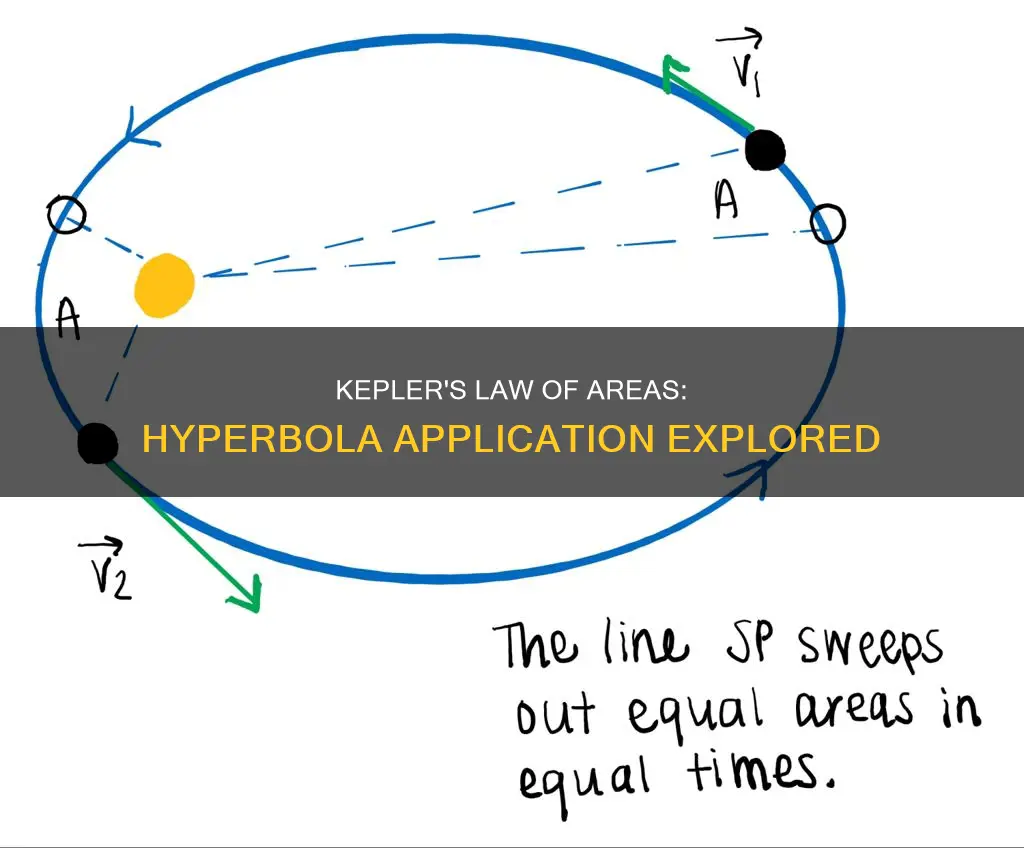
Kepler's laws of planetary motion describe how planetary bodies orbit the Sun. Kepler's second law, also known as the area law, states that a line joining a planet and the Sun sweeps out equal areas of space during equal time intervals as the planet orbits. This law applies to all types of central force motion, including elliptical, hyperbolic, and parabolic orbits.
| Characteristics | Values |
|---|---|
| Does Kepler's Law of Areas apply to hyperbolas? | Yes |
| What is the Law of Areas? | A line that connects a planet to the sun sweeps out equal areas in equal times |
| What is the Second Law of Kepler also known as? | The area law |
| What type of orbits does the Second Law apply to? | All types of central force motion, including hyperbolic and parabolic orbits |
What You'll Learn

Kepler's Second Law
The Law of Areas applies to all types of central force motion, including hyperbolic and parabolic orbits. This is because the angular momentum of the particle remains constant, and the area swept out by the particle in a given time interval is proportional to its rate of change.
Delaware Corporation Law: California Compliance and Considerations
You may want to see also

Hyperbolic Orbits
Kepler's laws of planetary motion describe how planetary bodies orbit the Sun. Kepler's Second Law, also known as the Law of Areas, states that a line that connects a planet to the Sun sweeps out equal areas in equal times. This law applies to all types of central force motion, including hyperbolic orbits.
A body can have three types of orbits around another body: an ellipse, a hyperbola, or a parabola. Kepler's Second Law holds for any central force motion. This can be proven by the fact that the angular momentum of the particle remains constant and the area swept out by the particle in a given time interval is proportional to its rate of change.
The Second Law for hyperbolas states that the line connecting a planet to the Sun sweeps out equal areas in equal time intervals. This law applies to planets that follow a hyperbolic orbit around the Sun. The eccentricity of the planet's orbit and the mass of the Sun affect the application of the Second Law for hyperbolas. A higher eccentricity or a more massive Sun will result in a faster sweeping of areas, while a lower eccentricity or a less massive Sun will result in a slower sweeping of areas.
Antique Firearms: Concealed Carry Law Exemptions?
You may want to see also

Central Force Motion
When we consider the motion of a particle under the influence of a central force, we are examining a type of motion where the force acting on the particle is always directed towards a fixed point in space, often called the center or focus. This concept is essential in understanding the motion of celestial bodies, such as planets, and it plays a crucial role in answering the question of whether Kepler's law of areas applies to hyperbolas.
One of the fundamental principles governing central force motion is the conservation of angular momentum. Due to the nature of the central force always pointing towards a fixed point, the angular momentum of the particle remains constant. This conservation law has profound implications for the shape and orientation of the particle's trajectory. For example, in the case of an attractive central force, the trajectory will always be an ellipse, a parabola, or a hyperbola, which are all types of conic sections.
Now, let's bring this concept back to the question at hand: Does Kepler's law of areas apply to hyperbolas? Kepler's law of areas states that, for a planet moving around the Sun, the line segment connecting the planet to the Sun sweeps out equal areas in equal time intervals. In other words, the rate at which the area is swept out is constant. This law is a direct consequence of the conservation of angular momentum in central force motion.
When we consider the specific case of hyperbolas, we are dealing with trajectories that are open and curved, and they represent situations where the total energy of the system is greater than the potential energy associated with the central force. In this context, Kepler's law of areas indeed applies to hyperbolas. As the particle moves along the hyperbolic trajectory, the line segment connecting the particle to the focus (or center) will still sweep out equal areas in equal time intervals. This is because the conservation of angular momentum holds regardless of the specific shape of the trajectory.
In summary, central force motion encompasses the motion of a particle influenced by a force directed towards a fixed point. This motion results in trajectories that are conic sections, including ellipses, parabolas, and hyperbolas. Kepler's law of areas, which arises from the conservation of angular momentum, applies generally to central force motion, including cases where the trajectory is a hyperbola. Thus, we can conclude that Kepler's law of areas is applicable to hyperbolas in the context of central force motion.
Good Samaritan Laws: Residence Life's Legal Shield?
You may want to see also

Angular Momentum
Kepler's Second Law states that a planet sweeps out equal areas in equal intervals of time. This is also known as the equal-area law or the areal velocity law.
The law is illustrated by considering the orbit of a planet as a series of triangles with the Sun at their apex. Each triangle has the same base (the distance travelled by the planet in a given time interval) and height (the distance of the planet from the Sun). Therefore, each triangle has the same area.
The planet moves faster when it is closer to the Sun and slower when it is farther away. This is because angular momentum is conserved. Angular momentum is the product of the planet's distance from the Sun and its velocity. As the distance from the Sun changes, the velocity must also change to keep the angular momentum constant.
Kepler's Second Law can be expressed mathematically as:
> "Equal areas in equal times" means the rate at which area is swept out on the orbit (dA/dt) is constant. So Kepler's Second Law Revised:
> The rate at which a planet sweeps out area on its orbit is equal to one-half its angular momentum divided by its mass (
> the specific angular momentum). Angular momentum is conserved.
Kepler's Second Law applies to elliptical orbits. Hyperbolas are unbounded or open orbits. Therefore, Kepler's Second Law does not apply to hyperbolas.
Biometric Privacy Laws: Who Are They Targeting?
You may want to see also

Astrodynamics
Kepler's first law states that planets follow an elliptical orbit with the Sun at one of the two foci. This law replaced the previously held belief that planets orbited the Sun in perfect circles. The ellipse can be defined as a set of points where the sum of the distances from two focal points remains constant.
The second law, also known as the area law, states that a line joining a planet and the Sun sweeps out equal areas of space during equal intervals of time. In other words, a planet covers the same area in the same amount of time, regardless of its position in the orbit. This law applies to all types of central force motion, including hyperbolic and parabolic orbits. As a planet moves closer to the Sun, its speed increases, and it covers a longer path in a given time.
The third law, also known as the law of periods, states that the square of a planet's orbital period is directly proportional to the cube of the semi-major axis of its orbit. This law implies that the time it takes for a planet to orbit the Sun increases with the radius of its orbit.
These laws were formulated by Johannes Kepler based on the extensive and precise data collected by Tycho Brahe. Kepler's laws are crucial in understanding the dynamics of our solar system and have applications in astrodynamics, such as calculating the trajectories of spacecraft and studying the motion of celestial bodies like comets.
HOAs and Sunshine Laws: What's the Deal in Pennsylvania?
You may want to see also
Frequently asked questions
Kepler's Law of Areas, also known as his second law, states that a line joining a planet and the Sun sweeps out equal areas in equal time intervals. This means that planets do not move at a constant speed along their orbits.
Yes, Kepler's Law of Areas applies to all types of central force motion, including hyperbolic and parabolic orbits. This is because the angular momentum of the particle remains constant, and the area swept out by the particle in a given time interval is proportional to its rate of change.
Kepler's Law of Areas for hyperbolas is different from that for ellipses because it applies to planets with hyperbolic orbits, while the law for ellipses applies to planets with elliptical orbits.
Kepler's Law of Areas for hyperbolas helps scientists understand the motion of planets in hyperbolic orbits around the Sun. It also allows them to predict a planet's position at a given time, based on the amount of area it has swept in a certain time.







