Hooke's Law is a fundamental principle of physics that states that the force required to extend or compress a spring is directly proportional to the distance of that extension or compression. The law was discovered by 17th-century British physicist Robert Hooke, who was studying springs and elasticity. Hooke's Law can be applied to any elastic object, and is the first classical example of an explanation of elasticity.
| Characteristics | Values |
|---|---|
| Force | F |
| Displacement | X |
| Spring Constant | K |
| Stiffness | K |
| Stress | Force on unit areas within a material |
| Strain | Relative deformation produced by stress |
What You'll Learn

Springs
Hooke's Law is the principle of physics behind the elasticity, torsion, and force involved with springs. The law is named after 17th-century British physicist Robert Hooke, who first stated the law in 1660 as a Latin anagram and published the solution in 1678 as "ut tensio, sic vis", which means "as the extension, so the force" or "the extension is proportional to the force".
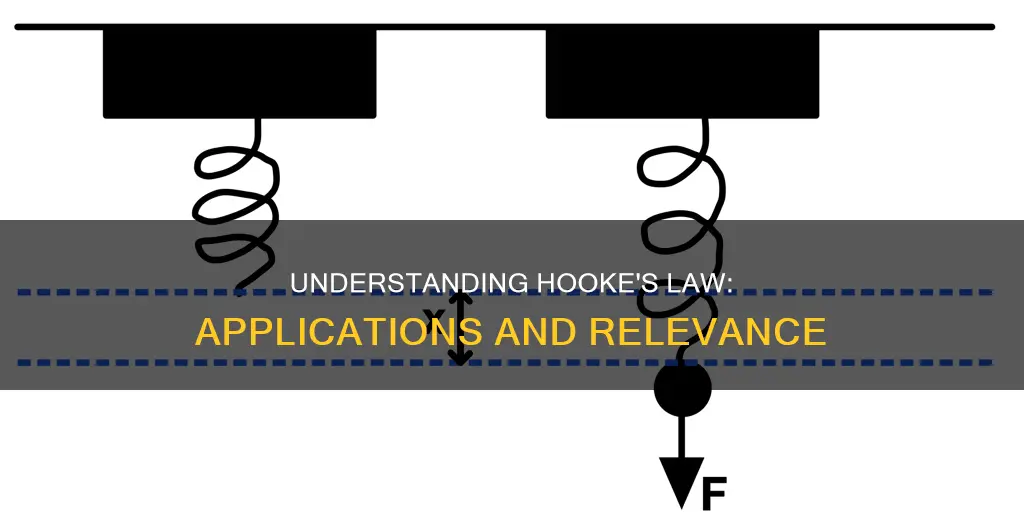
Hooke's Law states that the extension of a spring is proportional to the load that is applied to it. The length of a spring always changes by the same amount when it is pushed or pulled. In the case of a linear spring being pushed or pulled in one direction, the mathematical representation of Hooke's Law is as follows:
- "F" is the amount of push or pull on the spring
- "k" is a constant, indicating the stiffness of the spring
- "x" is the distance the spring was pushed or pulled
The spring constant "k" is related to the rigidity or stiffness of a system. The larger the spring constant, the greater the restoring force and the stiffer the system. The units of "k" are newtons per meter (N/m).
Hooke's Law is a close approximation of all solid bodies, as long as the forces of deformation are small enough. It is extensively used in all branches of science and engineering and is the foundation of many disciplines such as seismology, molecular mechanics, and acoustics.
The Law of Definite Composition: Understanding Its Mixture Applicability
You may want to see also

Elastic objects
Hooke's Law applies to elastic objects, and these can be very diverse. Springs are the most obvious example, but Hooke's Law also applies to anything from rubber bands to tall buildings swaying in the wind. The law can also be applied to continuous media, such as a block of rubber, the wall of a boiler, or a steel bar.
The modern theory of elasticity generalises Hooke's Law, stating that the deformation of an elastic object is proportional to the stress applied to it. This is where the term "linear-elastic" or "Hookean" comes from.
Hooke's Law is a foundational principle in many disciplines, including seismology, molecular mechanics, and acoustics. It is also the basis for the spring scale, the manometer, the galvanometer, and the balance wheel of the mechanical clock.
Hooke's Law is expressed mathematically as F = -kx, where F is the force applied to the spring, X is the displacement of the spring, and k is the spring constant.
The All-or-None Principle: Muscle Contraction Law
You may want to see also

Stress and strain
Hooke's Law is a principle of physics that states that the force required to extend or compress a spring is proportional to the distance of that extension or compression. The law is named after 17th-century physicist Robert Hooke, who discovered it in 1660. It can be expressed as F = -kX, where F is the force applied to the spring, X is the displacement of the spring, and k is the spring constant.
Hooke's Law applies to many situations where an elastic body is deformed, such as inflating a balloon, pulling a rubber band, or measuring the wind force on a tall building. It is also the foundation of many scientific and engineering disciplines, including seismology, molecular mechanics, and acoustics.
Strain is the relative deformation produced by stress. It is the ratio of the total deformation or change in length, ΔL, to the initial length, L0: ε = ΔL/L0. Strain is a unitless quantity.
Hooke's Law can be expressed in terms of stress and strain. For small stresses, stress is proportional to strain. This can be expressed as F = YA(ΔL/L0), where F is the applied force, A is the cross-sectional area, and ΔL is the change in length. This can be rearranged to give F/A = Y(ΔL/L0), which is analogous to Hooke's Law, with stress analogous to force and strain analogous to deformation.
The stress-strain relationship is important in engineering. Engineers carefully select structural materials that can safely endure everyday stress while remaining in the elastic region of the stress-strain curve. This ensures that the materials do not undergo permanent deformation.
The Law and You: Who Does it Affect?
You may want to see also

Energy
Hooke's law is a principle of physics that states that the force required to extend or compress a spring by some distance is directly proportional to that distance. This law applies to many elastic bodies, as long as the load does not exceed the elastic limit of the material.
The energy stored in a spring is known as elastic potential energy. This energy is equal to the work done to stretch or compress the spring, which depends on the spring constant and the distance stretched or compressed. The spring constant, also known as the rate or stiffness, is a property of the spring that characterises its resistance to deformation.
The potential energy stored in a spring can be calculated using the formula:
> PE_el = 1/2 kx^2
Where:
- PE_el is the elastic potential energy
- K is the spring constant
- X is the displacement of the spring
This formula shows that the potential energy stored in a spring is directly related to the square of the displacement. Therefore, as the spring is stretched or compressed further from its equilibrium position, the potential energy stored in it increases.
When a spring is stretched or compressed, work is done against the spring force. This work is stored as potential energy in the spring, which can be released if the spring is allowed to return to its original shape. This principle is utilised in various applications, such as clocks, watches, and musical instruments.
Benford's Law: A Strategy to Win at Roulette?
You may want to see also

Torsion
The behaviour of torsion springs is described by Hooke's Law, an angular form of the law. The torque exerted by the spring is proportional to the angle of twist from its equilibrium position, with the negative sign indicating the opposite direction of the torque and twist. The energy stored in a torsion spring is given by the equation:
> {\displaystyle U={\frac {1}{2}}\kappa \theta ^{2}}
Where:
- {\displaystyle \kappa \,} is the spring's torsion coefficient
- {\displaystyle \theta \,} is the angle of twist in radians
- {\displaystyle U\,} is the energy in joules
Lemon Law in Iowa: Private Sales Protection?
You may want to see also
Frequently asked questions
Hooke's Law is a principle of physics that states that the force required to extend or compress a spring is proportional to the distance of the extension or compression.
The equation for Hooke's Law is F = -kx, where F is the force applied to the spring, X is the displacement of the spring, and k is the spring constant.
Hooke's Law applies to any elastic object where the deformation and stress can be expressed by a single number. Materials that follow Hooke's Law are known as ""Hookean" materials. Examples include springs and the muscular layers of the heart.
Hooke's Law is used in the creation of the balance wheel, which is a fundamental component of mechanical clocks, portable timepieces, spring scales, and manometers. It also serves as the foundation for fields such as seismology, molecular mechanics, and acoustics.







